The graph of the quadratic polynomial y=ax2+bx+c is as shown in the figure.Then
Step by step video & image solution for The graph of the quadratic polynomial y=ax^(2)+bx+c is as shown in the figure.Then by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
The graph of a quadratic polynomial y=ax2+bx+c,a,b,c∈R is as shown.
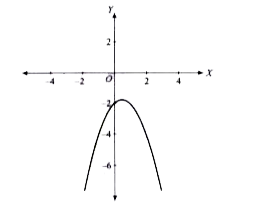
Which one of the is NOT correct ?Ab2−4ac<0Bca<0Cc is negativeDabscissa corresponding to the vertex is (−b2a) - Question 2 - Select One or More
If a,b,c ∈R, then for which of the following graphs of the quadratic polynomial y=ax2−2bx+c(a≠0), the product (abc) is negative ?
A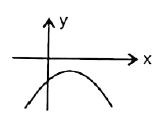 B
B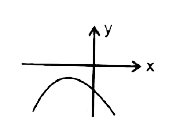 C
C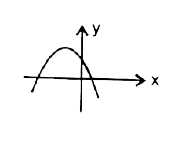 D
D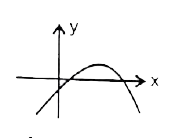
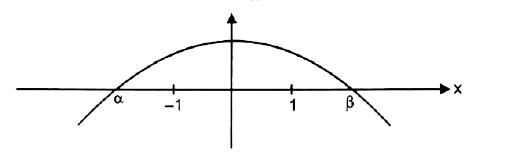
The graph of a quadratic polynomial y=px2−qx+r is as shown in the adjacent figure, then (i)r2−4q<0 (ii)r2−4p<0 (iii) p+q>0 (iv) r−p−q>0
View SolutionThe graph of quadratic polynomical f(x)=ax2+bx+c is shown below
 View Solution
View SolutionGraph of Quadratic Polynomial
View SolutionConsider the graph of quadratic polynomial y=ax2+bx+c as shown below. Which of the following is/are correct?
View SolutionThe graph of the polynomial f(x)=ax2+bx+c is as shown in Fig. 2.20. Write the value of b2−4ac and the number of real zeros of f(x) . (FIGURE)
View SolutionThe graph of a quadratic polynomial y=ax2+bx+c(a,b,c∈R) with vertex on yaxis is as shownin the figure. Then which one of the following statement is CORRECT?(A) Product the roots the corresponding quadratic equation is positive.Aof of(B) Discriminant of the quadratic equation is negative.(C) Nothing can be the sum of the roots, whether positive, negative or zero.definite said about(D) Both roots of the quadratic equation are purely imaginary.
View Solution
