Assume that f(1) = 0 and that for all integers m and n f(m+n) = f(m) + f(n) + 3(4mn-1) then f(19) = मान लें कि f(1)= 0 और कि सभी पूर्णांकों के लिए m और n f(m+n) = f(m)+f(m)+3(4mn-1) फिर f(19)
Video Solution
|
Answer
Step by step video & image solution for Assume that f(1) = 0 and that for all integers m and n f(m+n) = f(m) + f(n) + 3(4mn-1) then f(19) = मान लें कि f(1)= 0 और कि सभी पूर्णांकों के लिए m और n f(m+n) = f(m)+f(m)+3(4mn-1) फिर f(19) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
If f(1) = 1 and f(n + 1) = 2 f(n) + 1, if n ≥ 1, then f(n) is.
A2n+1B2nC2n−1D2n−1 - 1 - Question 2 - Select One
If m and n are positive integers and f(m,n)=∫10xn−1(logx)mdx, then f(m,n) is equal to
Amnf(m−1,n)B−mnf(m−1,n)Cnmf(m−1,n)DNone of these - Question 3 - Select One
If f(x+1)+f(x−1)=2f(x)andf(0)=0, then f(n),n∈Nis
Anf(1)B[f(1)]nC0DNone
Iff(x)=⎧⎪⎨⎪⎩mx2+nx<0nx+m0≤x≤1nx3+mx>1 .
For what integers m and n does both limx→0f(x) andlimx→1f(x) exist?View SolutionLet f:N→N be a function such that f(m+n)=f(m)+f(n) for every m, n∈N. If f(6)=18, then f(2).f(3) is equal to :
View SolutionLet f : R - {n} → R be a function defined by f(x)=x−mx−n, where m≠n. Then,
View SolutionIn Fig if F=4N,m=2kgM=4kg then
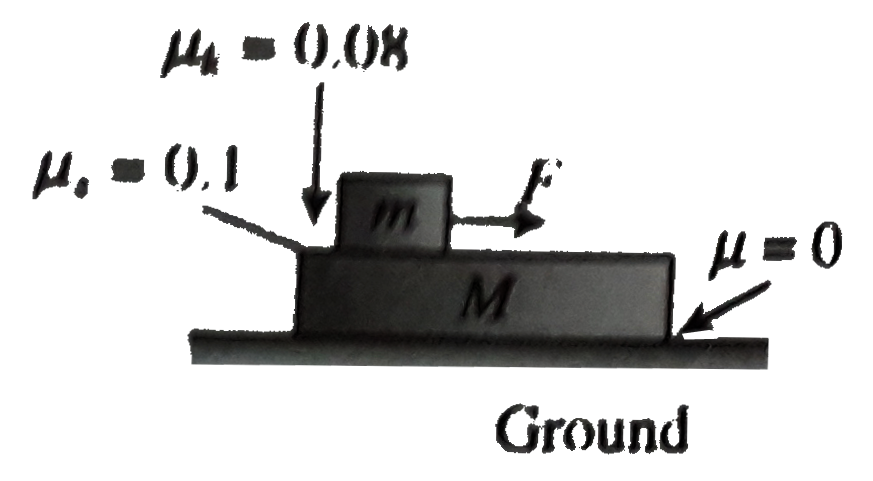 View Solution
View SolutionLet f:N→R be a function such that f(1)+2f(2)+3f(3)+....+nf(n)=n(n+1)f(n), for n≥2 and f(1) = 1 then
View Solution
