Damped harmonic oscillator consists of a block (m=2kg) a spring (k=8π2Nm) and a damping force (F=−bv) .Initially it oscillates with an amplitude of 25cm. Because of the damping the amplitude falls to three-fourth of this initial value at the completion of four oscillations.What is the value of b ? (Assume small damping and take : ln(34)=−0.28
Step by step video & image solution for Damped harmonic oscillator consists of a block (m=2kg) a spring (k=8 pi^(2)N/m) and a damping force (F=-bv) .Initially it oscillates with an amplitude of 25cm. Because of the damping the amplitude falls to three-fourth of this initial value at the completion of four oscillations.What is the value of b ? (Assume small damping and take : ln((3)/(4))=-0.28 by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A block of mass 1 kg attached to a spring is made to oscillate with an initial amplitude of 12 cm. After 2 minutes the amplitude decreases to 6 cm. Determine the value of the damping constant for this motion. (take In 2 =0.693 )
View SolutionFor the damped oscillator shown in Fig, the mass of the block is 200g,k=80Nm−1 and the damping constant b is 40gs−1 Calculate
(a) The period of oscillation
(b) Time taken for its amplitude of vibrations to drop to half of its initial values
(c) The time for the mechanical energy to drop to half initial values
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In damped oscillations, the amplitude is reduced to one-third of its initial value a0 at the end of 100 oscillations. When the oscillator completes 200 oscillations ,its amplitude must be
Aa0/2Ba0/4Ca0/6Da0/9 - Question 2 - Select One
The amplitude of a damped oscillator becomes 1(27)th of its initial value after 6 minutes. Its amplitude after 2 minutes is
AA03BA09CA054DA081 - Question 3 - Select One
A book is resting on shelf that is undergoing vertical simple harmonic oscillations with an amplitude of 2.5 cm. What is the minimum frequency of oscillation of the shell for which the book will lose contact with the shelf ? (Assume that g=10m/s2)
A20 HzB3.18 HzC125.6 HzD10 Hz
A simple pendulum oscillates with an amplitude of 2×10−2m. If the force acting on it at extreme position is 4 N. Then the time that another simple pendulum of length 81 cm takes to execute the same number of oscillations is
View SolutionIf initial amplitude during a damped oscillation of mass m is 12 cm and after 2s it reduces to 6cm then find damping constant(b)
View SolutionThe amplitude of damped oscillator becomes 13 in 2s. Its amplitude after 6s is 1/n times the original. The value of n is
View Solutionmx2−bx+k=0
Find time after which of the energy will be come half of initial maximum value in damped forced oscillation .View Solution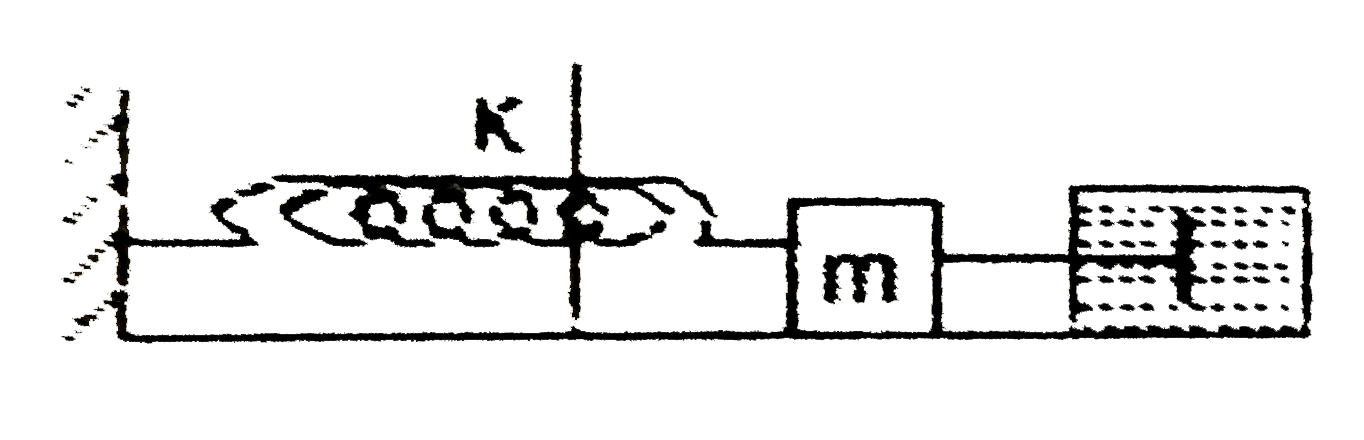
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.View Solution
