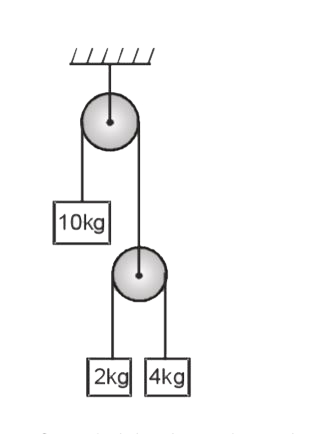
In the figure shown, find out acceleration of each block.
Now F.B.D. of each block and apply Newton's second law on each F.B.D.
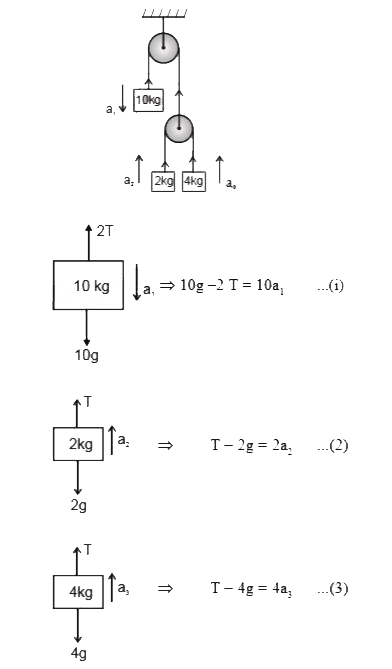
from constrain relation 2a1=a2+a3 ....(4)
Solving equations ( 1), (2), ( 3) and ( 4) we get T=80023N
a1=70/23m/s2 ( downward ) ,a2=170/23m/s2 ( upward),
a3=30/23m/s2 ( downward )
Topper's Solved these Questions
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - SOLVED EXAMPLE
16 Videos
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXERCIS-1
80 Videos
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXERCISE-4 ( LEVEL-II)
15 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 3 Section-B
12 Videos
- ONE DIMENSION MOTIONBOOK - MOTIONCHAPTER - ONE DIMENSION MOTIONEXERCISE - Exercise - 3 |Section - B Previous Year Problems | JEE MAIN
12 Videos
Similar Questions
Knowledge Check
Find the acceleration of each block
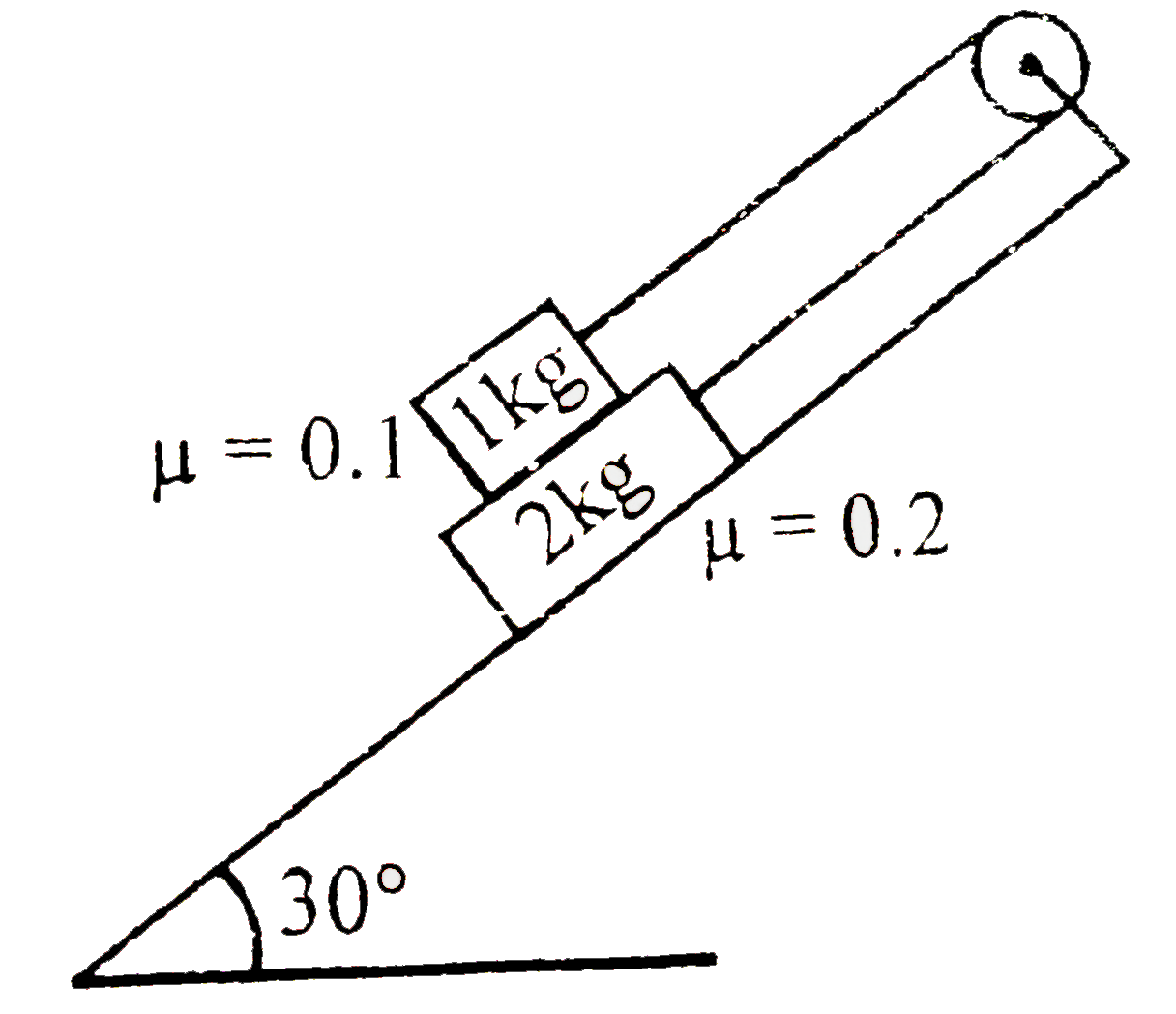 A215m/s2B6215m/s2CzeroD25m/s2
A215m/s2B6215m/s2CzeroD25m/s2In the figure shown, the acceleration of block of mass m2 is 2m/s2. The acceleration of m1 :
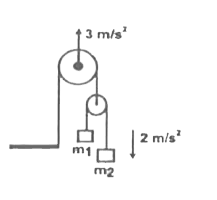 Awill be 8m/s2 upwardBwill be 4m/s2 upwardCwill be 14m/s2 upwardDThe value depends upon actual values of m1 and m2
Awill be 8m/s2 upwardBwill be 4m/s2 upwardCwill be 14m/s2 upwardDThe value depends upon actual values of m1 and m2For the system shown in figure, the acceleration of the block is :-
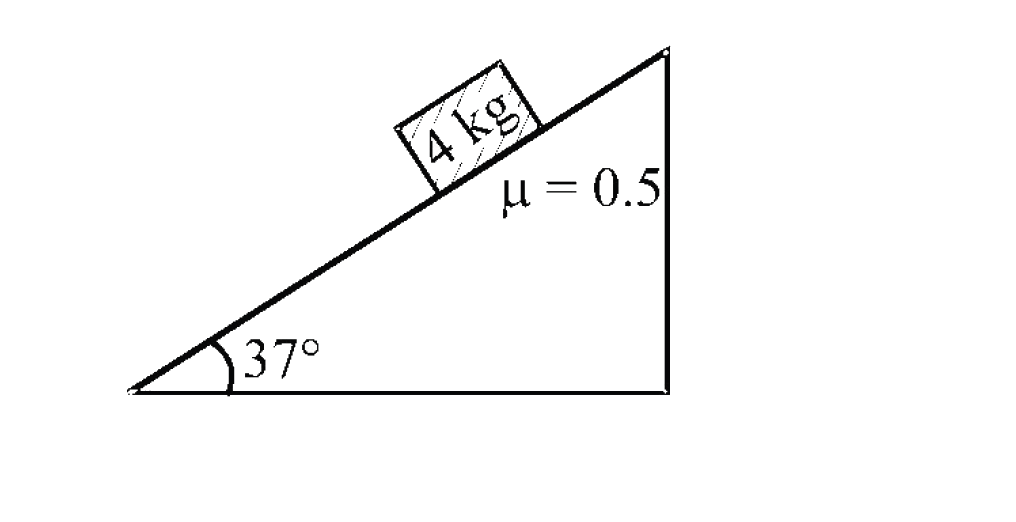 A1m/s2B2m/s2C3m/s2D4m/s2
A1m/s2B2m/s2C3m/s2D4m/s2
Two blocks are attached by a light inextensible string and the system is pulled by applying a force F0 as shown in the diagram. Find the acceleration of each block and tension in the string.
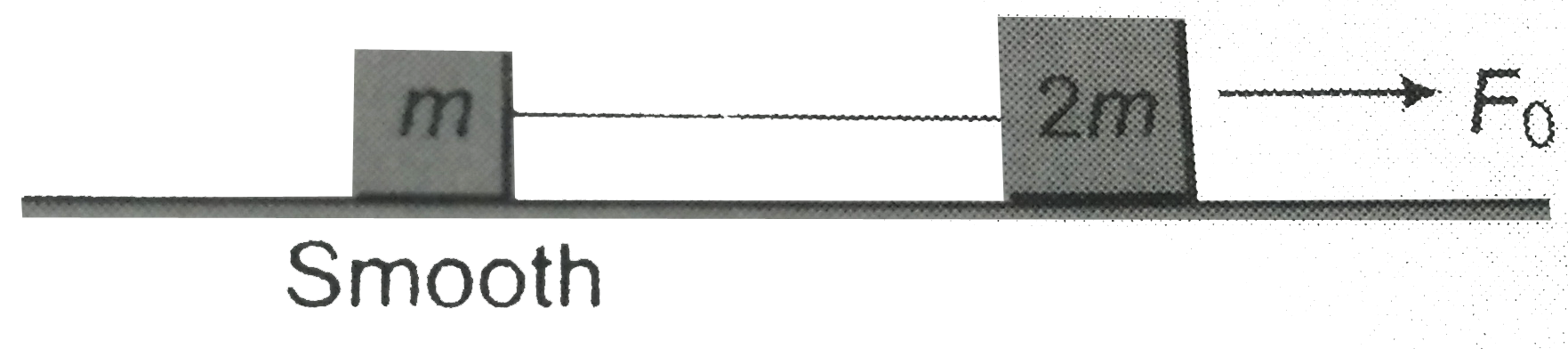
View SolutionConsider the situation as shown in the figgure. The coefficent of friction between the blocks is μ. Find the acceleration of each block.
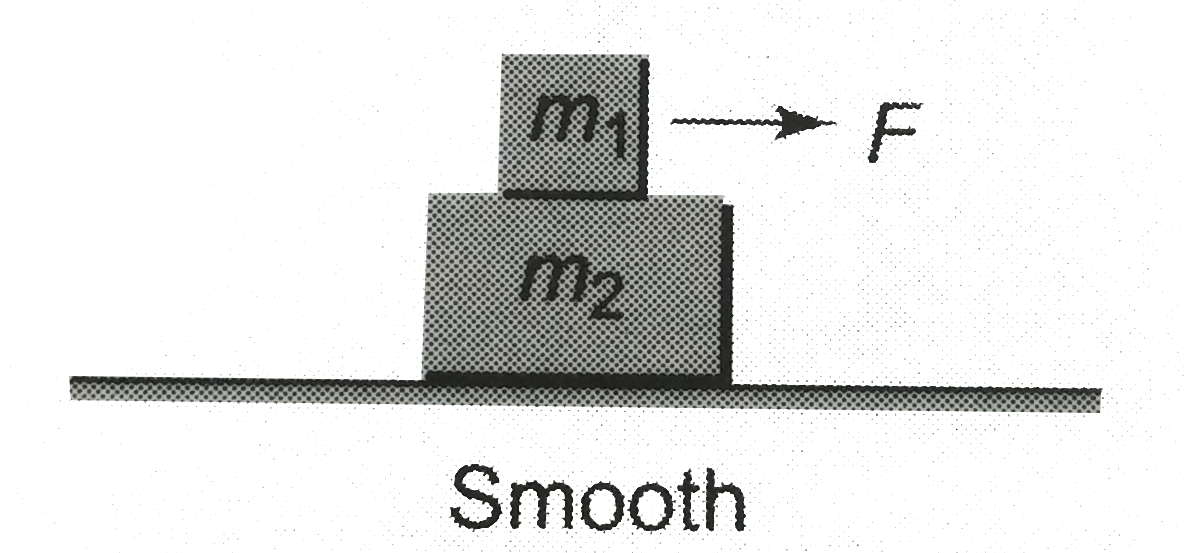
View SolutionMass m1 & m2 are placed on a rough inclined plane as shown in figure. Find out the acceleration of the blocks and contact force in between these surface.
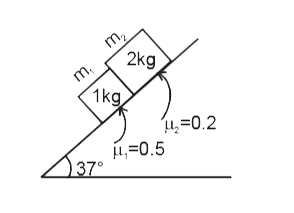
View SolutionIn the given figure , what wil be the acceleration of each block ?
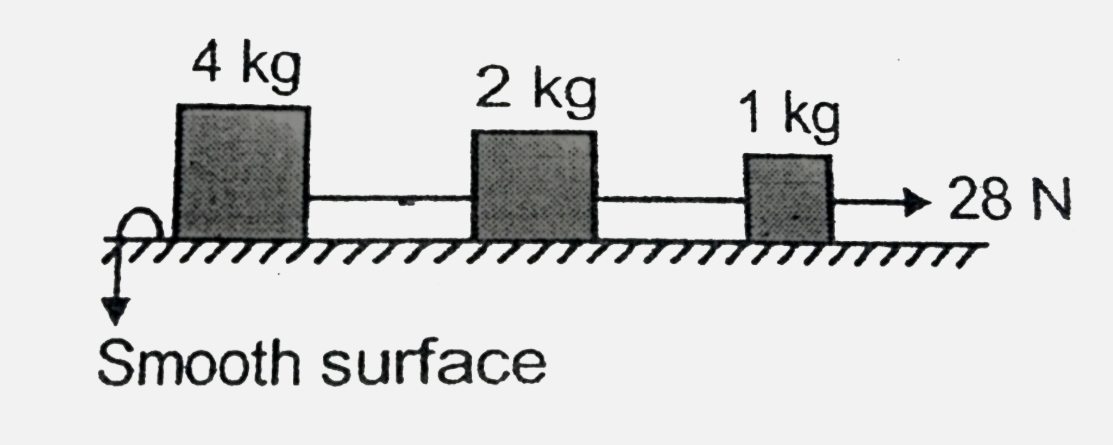
View SolutionConsider the situation shown in the figure. The surface is smooth and the string and the pulley are light. Find the acceleration of each block and tension in the string.
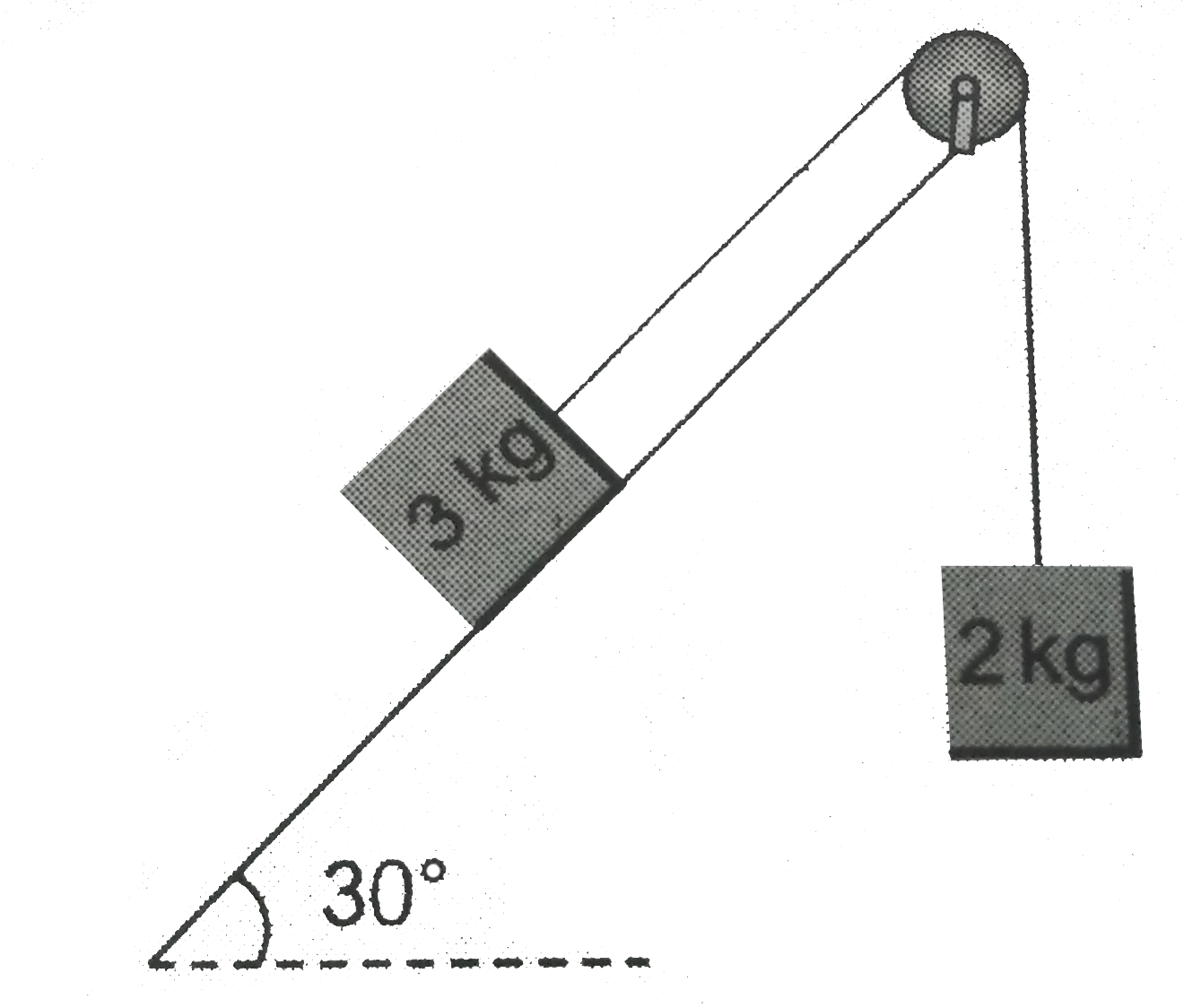
View Solution