Question

Two rods each having length l and mass m joined together at point B as shown in figure.Then findout moment of inertia about axis passing thorugh A and perpendicular to the plane of page as shown in figure.

Explanation

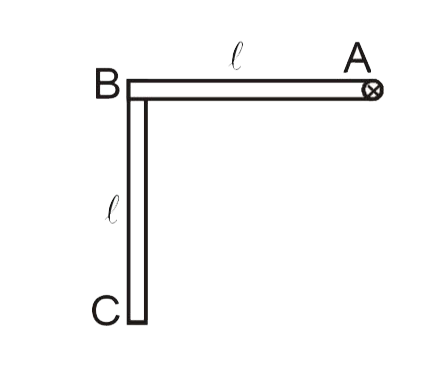
We find the resultant moment of inertia I by dividing in two parts such as
` I = M . I ` of rod AB about A +
M . I of rod BC about A
` I = I_(1) + I_(2) " " . . . . (1)`
first calculate `I_(1) ` :
` I_(1) = (ml^(2))/(3) " " . . . .(2)`
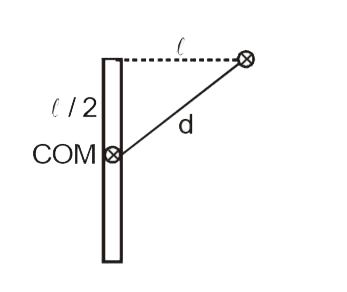
Calculation of `I_(2) ` :
`I_(2) = I_(CM) + md^(2)`
`= (ml^(2))/(12) + m ((l^(2))/(4) + l^(2)) = (ml^(2))/(12) + (5l^(2))/(4)m " ". . . . (3)`
Put value from eq. (2) & (3) into (1)
`rArr I = (ml^(2))/(3) + (ml^(2))/(12) + (5l^(2)m)/(4)`
`I = (ml^(2))/(12) (4 + 1 + 15) rArr " " I = (5 m l ^(2))/(3)`