A magnetic tape isbeing played on a cassette deck. The tension in the tape applies a torque to the supply reel. Assuming the tension remains constant during play, plot this angular acceleration ith reel radius r as the reel becomes empty. Neglect the moment of inertia of empty reel.
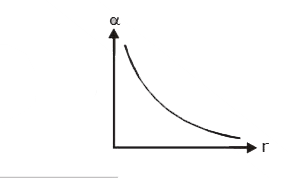
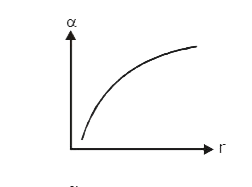
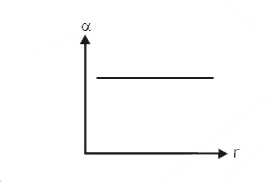
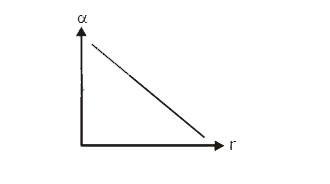
The correct Answer is:A
Step by step video, text & image solution for A magnetic tape isbeing played on a cassette deck. The tension in the tape applies a torque to the supply reel. Assuming the tension remains constant during play, plot this angular acceleration ith reel radius r as the reel becomes empty. Neglect the moment of inertia of empty reel. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 2 (Level -I)51videos
10 ROTATIONAL
- BOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 2 (Level - II)25videos
10 ROTATIONAL
- BOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Example 311videos
10 ROTATIONAL
- BOOK - MOTIONCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - 4 (LEVEL - II)14videos
ALTERNATING CURRENT
Similar Questions
A cotton reel of mass m and moment of inertia I is kept at rest on as smooth horizontal surface. The reel has inner and other radius r and R respectively. A horizontal force F starts actings as shown in figure. Find the

a. acceleration of the centre of mass of reel.
b. angular acceleration of the reel
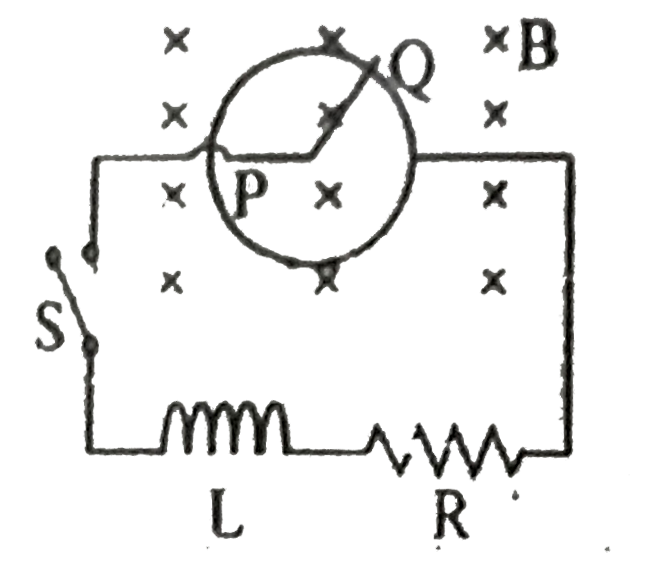
c. net acceleration of point of contact.View SolutionThe diagram shows a circuit having a coil of resistance R=10Ω and inductance L connected to a conducting rod PQ which can slide on a perfectly conducting circular ring of radius 10 cm with its centre at 'P' Assume that friction & gravity are absent and a constant uniform magnetic field of 5 T exists as shown in figure. At t=0 the circuit is switched on and simultaneously a time varying external torque is applied on the rod so that it rotates about P with a constant angular velocity 40 rad//s Find magnitude of this torque (in mu Nm) when current reaches half of its maximum value. Neglect the self inductance of the loop formed by the circuit.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
An accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.

The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
After a long time, when the potential of the sphere reaches a constant value, the trajectory of proton is correctly sketched as
 A1B2C3D4
A1B2C3D4 - Question 2 - Select One
An accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.
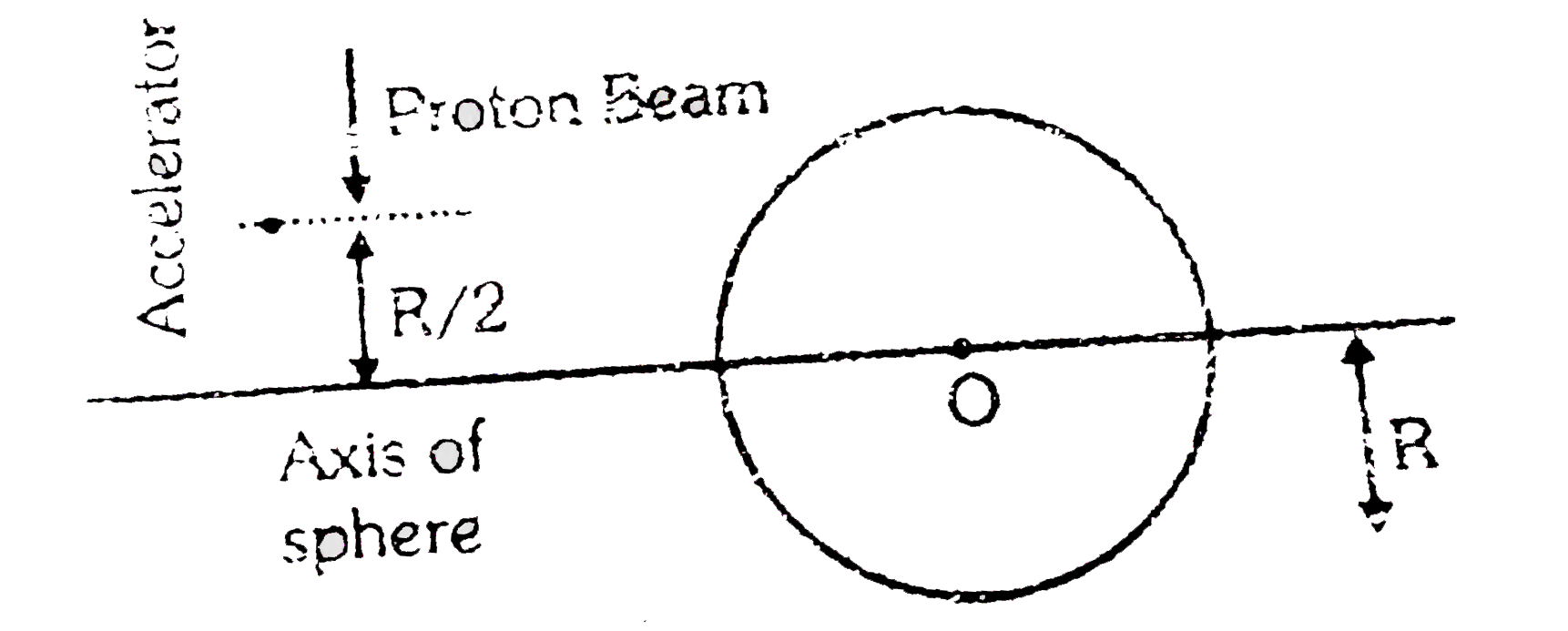
The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
One the potential of the sphere has reached its final, constant value, the minimum speed v of a proton along its trajectory path is given byAv0Bv0/2C2v0DNone of these - Question 3 - Select One
An accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.

The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
The limiting electric potential of the sphere isA3mPv208eB3mPv204eC3mPv202eDNone of these
An accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.
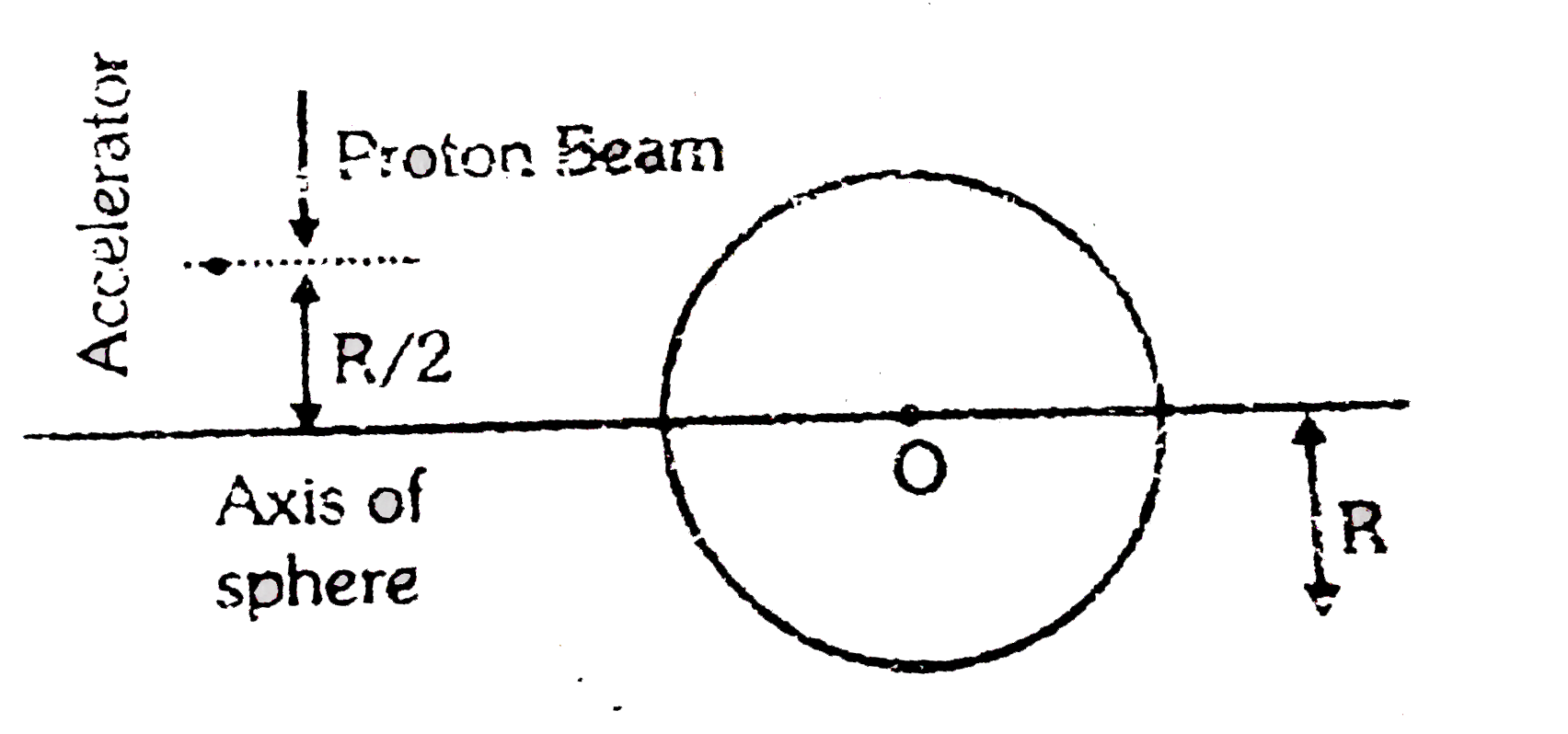
The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
If the initial kinetic energy of a proton is 2.56keV, then the final potential of the sphere isView SolutionAn accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.

The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
The total energy (E) of a proton in the beam travelling with seed v at a distance of r(r≥R) from point O. Assuming that the sphere has acquired an electrostatic charge Q isView SolutionThe reel shown in figure has radius R and moment of inertia I. One end of the block of mass m is connected to a spring of force constant k, and the other end is fastened to a cord wrapped around the reel. The reel axle and the incline are frictionless. The reel is wound counterclockwise so that the spring strectches a distance d from its unstretched position and the reel is then released from rest. The angular speed of the reel when the spring is again unstretched is.
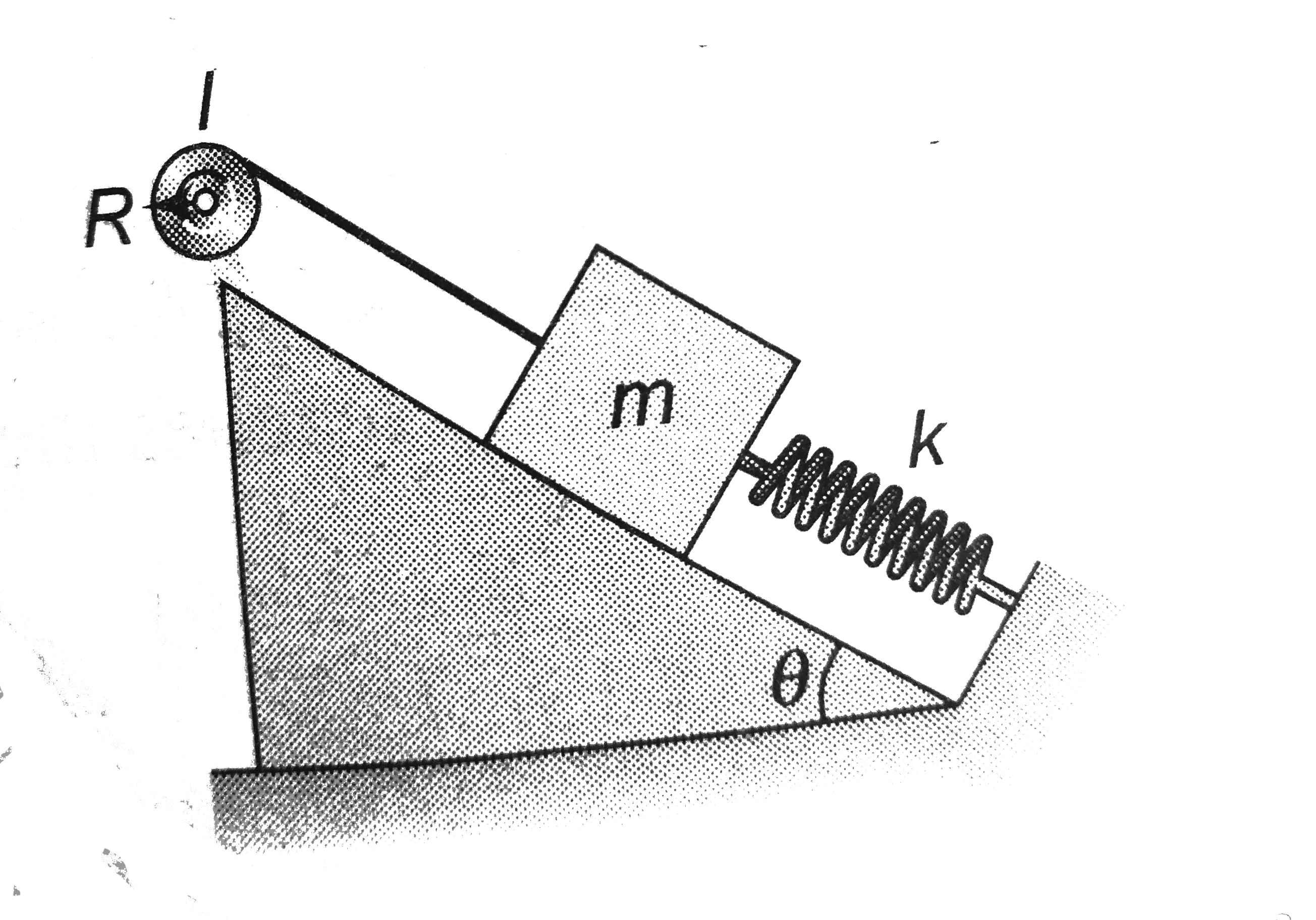 .View Solution
.View SolutionA mass 1kg attached to the end of a flexible rope of diameter d=0.25mis raised vertically by winding the rope on a reel as shown. If the reel is turned uniformly at the rate of 2r.p.s. What is the tension in rope. The inertia of rope may be neglected.
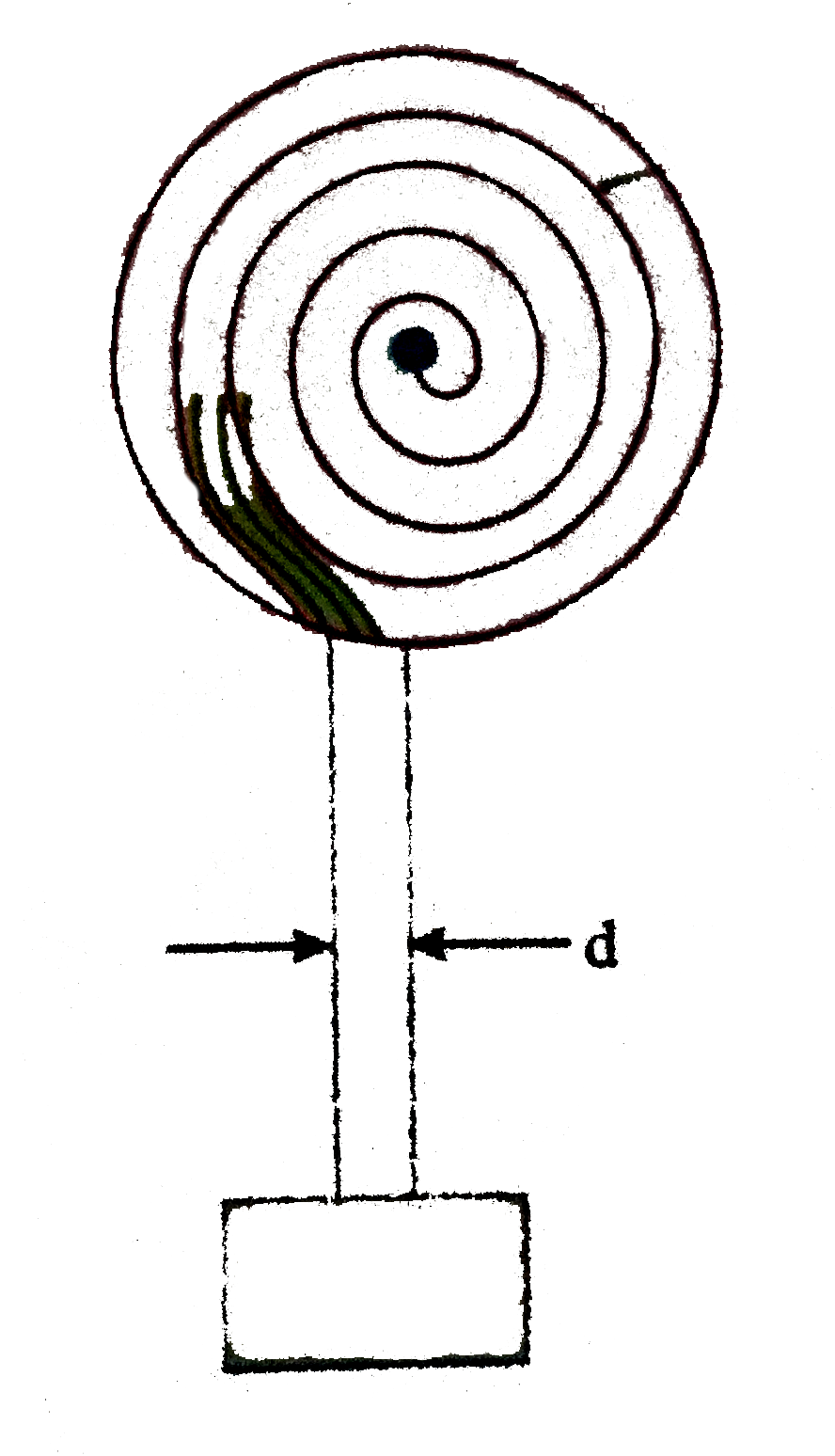 View Solution
View SolutionA point charges Q is moving in a circular orbit of radius R in the x-y plane with an angular velocity ω. This can be considered as equivalent to a loop carrying a steady current Qω2π. S uniform magnetic field along the positive z-axis is now switched on, which increases at a constant rate from 0 to B in one second. Assume that the radius of hte orbit remains constant. The application of hte magnetic field induces an emf in the orbit. The induced emf is defined as the work done by an induced electric field in moving a unit positive charge around a closed loop. It si known that, for an orbiting charge, the magnetic dipole moment is proportional to the angular momentum with a porportionality constant λ.
The charge in the magnetic dipole moment associated with the orbit. at the end of the time interval of hte magnetic field charge, isView Solution

