A cylinder of height h, diameter h/2 and mass M and with a homogeneous mass distribution is placed on a horizontal table. One end of a string running over a pulley is fastended to the top of the cylinder, a body of mass m is hung from the other end and the system is released. Friction is negligible every where. At what minimum ratio m/M will the cylinder tilt?
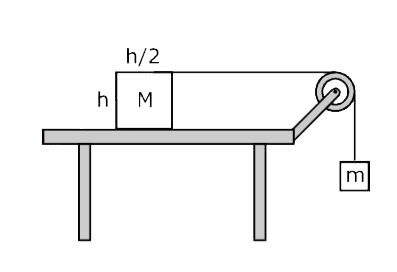
The correct Answer is:1/4
Step by step video & image solution for A cylinder of height h, diameter h//2 and mass M and with a homogeneous mass distribution is placed on a horizontal table. One end of a string running over a pulley is fastended to the top of the cylinder, a body of mass m is hung from the other end and the system is released. Friction is negligible every where. At what minimum ratio m//M will the cylinder tilt? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- 10 ROTATIONALBOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 3 (Level - II)
20 Videos
- 10 ROTATIONALBOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 4 (Level - I)
24 Videos
- 10 ROTATIONALBOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 2 (Level - II)
25 Videos
- ALTERNATING CURRENTBOOK - MOTIONCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - 4 (LEVEL - II)
14 Videos
Similar Questions
A block of mass m1 rests on a horizontal table. A string tied to the block is passed on a frictionless pulley fixed at the end of the table and to the other end of string is hung another block of mass m2. The acceleration of the system is
View SolutionIn the following figure, a body of mass m is tied at one end of a light string and this string is wrapped around the solid cylinder of mass M and radius R. At the moment t = 0 the system starts moving. If the friction is negligible, angular velocity at time t would be
View Solution
Knowledge Check
Two masses m1 and m2 are connected to the ends of a massless string that passes over a pulley fixed at the top of a double incline as shown. Assuming m1>m2 acceleration of the system is:
 Am1gsinθ1+m2gsinθ2m1+m2Bm1gsinθ1−m2gsinθ2m1−m2Cm1gsinθ1−m2gsinθ2m1+m2D(m1+m2)(sinθ1+sinθ2)gm1−m2
Am1gsinθ1+m2gsinθ2m1+m2Bm1gsinθ1−m2gsinθ2m1−m2Cm1gsinθ1−m2gsinθ2m1+m2D(m1+m2)(sinθ1+sinθ2)gm1−m2In the following figure, a body of mass m is tied at one end of a light string and this string is wrapped around the solid cylinder of mass M and radius R. At the moment t = 0 the system starts moving. If the friction is negligible, angular velocity at time t would be
 AmgRt(M+m)B2Mgt(M+2m)C2mgtR(M−2m)D2mgtR(M+2m)
AmgRt(M+m)B2Mgt(M+2m)C2mgtR(M−2m)D2mgtR(M+2m)A uniform horizontal metre scale of mass m is suspended by two vertical strings attached to its two ends. A body of mass 2m is placed on the 75 cm mark. The tensions in the two strings are in the ratio is
A1:2B1:3C2:3D3:4
A block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View SolutionA uniform solid cylinder of mass M and radius 2R rests on a horizontal table top. A string attached to it runs over a pulley (disc) of mass M and radius R thatis mounted on a frictionless axle through its centre. A block of mass M is suspended from the free end of the string. The string doesn't slip over the pulley surface, and the cylinder rolls without slipping on the, table top. Find the acceleration of the block.

[g/3]
View SolutionA mass m rests on a horizontal table. It is attached to a light inextensible string which passes over a smooth pulley and carries a mass m at the other end. If the mass m is raised vertically through a distance h and is then dropped, what is the speed with which the mass 2m begins to rise?

View SolutionA uniform horizontal meter scale of mass m is susopended by two certical string attached to its two ends. A body of mass 2m is place on the 75−cm mark. The tensions in the two strings are in the ratio
View SolutionA block of mass m1 rests on a horizontal table. A string tied to the block is passed on a frictionless pulley fixed at the end of the table and to the other end of string is hung another block of mass m2. The acceleration of the system is
View Solution
