The charge of a parallel plate capacitor is varying as q = q0 sin ωt. Then find the magnitude of displacement current through the capacitor. (Plate Area = A, separation of plates = d)
q0cos(ωt)
q0ωsinωt
q0ωcosωt
q0Aωdcosωt
The correct Answer is:C
To find the magnitude of the displacement current through a parallel plate capacitor with a varying charge given by q=q0sin(ωt), we can follow these steps:
1. Understand the Concept of Displacement Current:
The displacement current Id is defined in the context of Maxwell's equations. It accounts for the changing electric field in regions where there is no conduction current. The formula for displacement current is given by:
Id=ϵ0dΦEdt
where ΦE is the electric flux.
2. Calculate the Electric Flux:
The electric flux ΦE through the capacitor can be expressed as:
ΦE=E⋅A
where E is the electric field between the plates and A is the area of the plates. The electric field E can be related to the charge q on the capacitor:
E=qϵ0A
Substituting q=q0sin(ωt):
E=q0sin(ωt)ϵ0A
Therefore, the electric flux becomes:
ΦE=E⋅A=q0sin(ωt)ϵ0A⋅A=q0sin(ωt)ϵ0
3. Differentiate the Electric Flux:
Now we differentiate the electric flux with respect to time t:
dΦEdt=ddt(q0sin(ωt)ϵ0)=q0ωcos(ωt)ϵ0
4. Substitute into the Displacement Current Formula:
Now substitute dΦEdt back into the displacement current equation:
Id=ϵ0dΦEdt=ϵ0⋅q0ωcos(ωt)ϵ0
The ϵ0 cancels out:
Id=q0ωcos(ωt)
5. Final Result:
Thus, the magnitude of the displacement current through the capacitor is:
Id=q0ωcos(ωt)
The displacement current Id is defined in the context of Maxwell's equations. It accounts for the changing electric field in regions where there is no conduction current. The formula for displacement current is given by:
Id=ϵ0dΦEdt
where ΦE is the electric flux.
The electric flux ΦE through the capacitor can be expressed as:
ΦE=E⋅A
where E is the electric field between the plates and A is the area of the plates. The electric field E can be related to the charge q on the capacitor:
E=qϵ0A
Substituting q=q0sin(ωt):
E=q0sin(ωt)ϵ0A
Therefore, the electric flux becomes:
ΦE=E⋅A=q0sin(ωt)ϵ0A⋅A=q0sin(ωt)ϵ0
Now we differentiate the electric flux with respect to time t:
dΦEdt=ddt(q0sin(ωt)ϵ0)=q0ωcos(ωt)ϵ0
Now substitute dΦEdt back into the displacement current equation:
Id=ϵ0dΦEdt=ϵ0⋅q0ωcos(ωt)ϵ0
The ϵ0 cancels out:
Id=q0ωcos(ωt)
Thus, the magnitude of the displacement current through the capacitor is:
Id=q0ωcos(ωt)
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC WAVES EXERCISE - EXERCISE - 236videos
ELECTRO MAGNETIC WAVES
- BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC WAVES EXERCISE - EXERCISE - 3 (SECTION - A)23videos
ELECTRO MAGNETIC WAVES
- BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC WAVES EXERCISE - EXERCISE - 3 (SECTION - B)8videos
ELECTRO MAGNETIC WAVES
- BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - EXERCISE-4| Section B (Prevous Years Problems )29videos
ELECTRO MAGNETIC INDUCTION
- BOOK - MOTIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-4 (LEVEL-II)13videos
ELECTROMAGNETIC INDUCTION
Similar Questions
The charge on a parallel plate capacitor varies as q=q0cos2πt. The plates are very large and close together (area = A, separation = d). The displacement current through the capacitor is
View SolutionA parallel plate capacitor is charged and then isolated. On increasing the plate separation
View Solution
Knowledge Check
- Question 1 - Select One
If the plates of a parallel plate capacitor are not equal in area then quantity of charge
Aquantity of charge on the plates will be the same, but nature of charge will differ.Bquantity of charge as well as nature of charge on the plates will be differentCquantity of charge on the plates will be different, but nature of charge will be the same.Dquantity of charge as well as nature of charge will be the same. - Question 2 - Select One
If the plates of a parallel plate capacitor are not equal in area then quantity of charge
AOn the plates will be same but nature of charge will differBOn the plates as will as nature of charge will be differentCOn the plates will be different but nature of charge will be sameDAs well as nature of charge will be same - Question 3 - Select One
A parallel plate capacitor is charged and then isolated. On increasing the plate separation
A Charge Potential Difference Capacitanceremains constant remains constant decreasesB Charge Potential Difference Capacitanceremains constant increases decreasesC Charge Potential Difference Capacitanceremains constant decreases increasesD Charge Potential Difference Capacitanceincreases decreases increases
The negative plate of a parallel plate capacitor is given a charge of −20X10−8C. Find the charges appearing on the four surface of the capacitor plates.
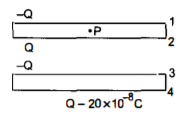 View Solution
View SolutionThe charge on a parallel plate capacitor varies as =q0cos2πft. The plates are very large and close together (area=a,separation=d). Neglecting the edge effects, find the displacement current through the capacitor.
View SolutionFind out the capacitance of parallel plate capacitor of plate area A and plate separation d.
View SolutionA parallel plate capacitor is charged. If the plates are pulled apart
View SolutionA parallel plate capacitor C has a charge Q. The actual charges on its plates are
View Solution

