The cryptogram of T2 coliphage is
D/2, 130/30, X/X, B/O
D/2, 3/7, S/S, V/O
D/2, 137/40, S/S, B/O
R/1,54/92, X/X, V/O
The correct Answer is:A
Step by step video, text & image solution for The cryptogram of T_(2) coliphage is by Biology experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - DINESH PUBLICATIONCHAPTER - VIRUSES VIROIDS AND PRIONSEXERCISE - CYG13videos
VIRUSES VIROIDS AND PRIONS
- BOOK - DINESH PUBLICATIONCHAPTER - VIRUSES VIROIDS AND PRIONSEXERCISE - CYG13videos
VIRUSES VIROIDS AND PRIONS
- BOOK - DINESH PUBLICATIONCHAPTER - VERTEBRATA-AGNATHA AND PISCESEXERCISE - Check Your Grasp9videos
VERTEBRATA-AGNATHA AND PISCES
Similar Questions
The vertices of a triangle are [at1t2,a(t1+t2)], [at2t3,a(t2+t3)], [at3t1,a(t3+t1)] Then the orthocenter of the triangle is (a) (−a,a(t1+t2+t3)−at1t2t3) (b) (−a,a(t1+t2+t3)+at1t2t3) (c) (a,a(t1+t2+t3)+at1t2t3) (d) (a,a(t1+t2+t3)−at1t2t3)
View SolutionThe velocity-time graph of a particle in one-dimensional motion is shown in Fig.:
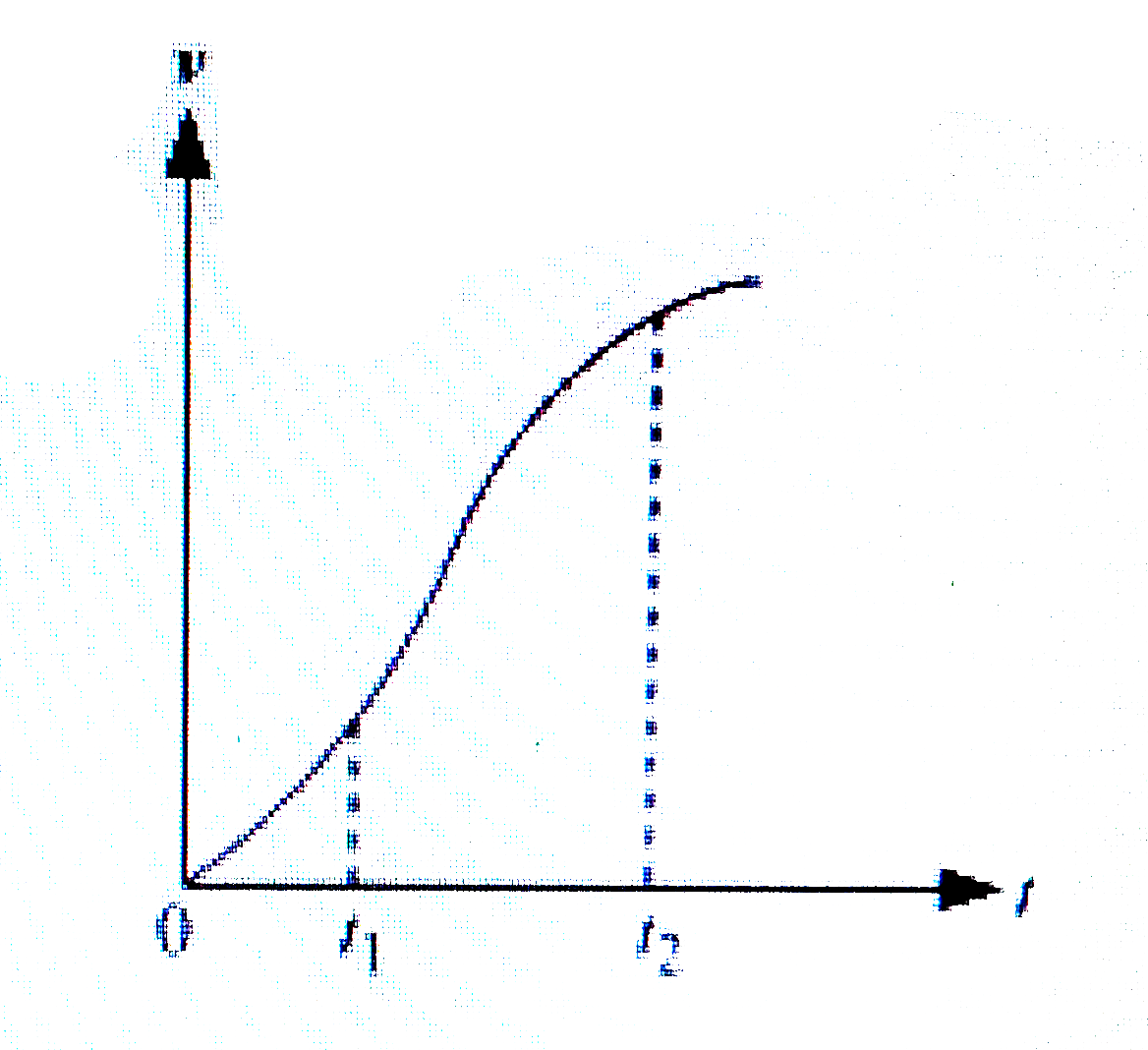
(a) Which of the following formulae are correct for describing the motion of the particle over the time-interval t1 to t2:
(a) x(t2)=x(t1)+v(t1)(t2−t1)+(1/2)a(t2−t1)2
(b) v(t2)=v(t1)+a(t2−t1)
(c) vaverage=(x(t2)−x(t1))/(t2−t1)
(d) aaverage=(v(t2)−v(t1))/(t2−t1)
(e) x(t2)=x(t1)+vaverage(t2−t1)+(1/2)aaverage(t2−t1)2
(f) x(t2)−x(t1) = area under the v−t curve bounded by the t-axis and the dotted line shown.View Solution
Knowledge Check
- Question 1 - Select One
The correct cryptogram for T4, bacteriophage is
AR/1,2.5/30,S/S.V/0BD/2,160/5−7.5,X/⋆,V/OCR/1:2/5:E/E:S/SDD/2:130/40:X/X:B/O - Question 2 - Select One
Cryptogram of TMV is
AR/1, 1/8, S/S, S/AfBR/2, 1/5, E/P, O/SCR/1, 2/5, E/E, S/ODR/1, 2/7, E/E, S/O - Question 3 - Select One
Coliphage contain
ARNABDNACRNA or DNADRNA as well as DNA
If A, B, C, be the centres of three co-axial circles and t1,t2,t3 be the lengths of the tangents of them any piont, prove that
¯¯¯¯¯¯BC.t21+¯¯¯¯¯¯CA.t22+¯¯¯¯¯¯AB.t23=0View SolutionNormals are drawn to the parabola y2=4ax at the points A, B, C whose parameters are t1,t2andt3, respectively. If these normals enclose a triangle PQR, then prove that its area is a22(t−t2)(t2−t3)(t3−t1)(t1+t2+t3)2 Also prove that ΔPQR=ΔABC(t1+t2+t3)2.
View SolutionThe normal at t1 and t2 on the parabola y2=4ax intersect on the curve then t1t2
View SolutionColiphage T2 has
View SolutionA cryptogram has got how many pairs of information
View Solution

