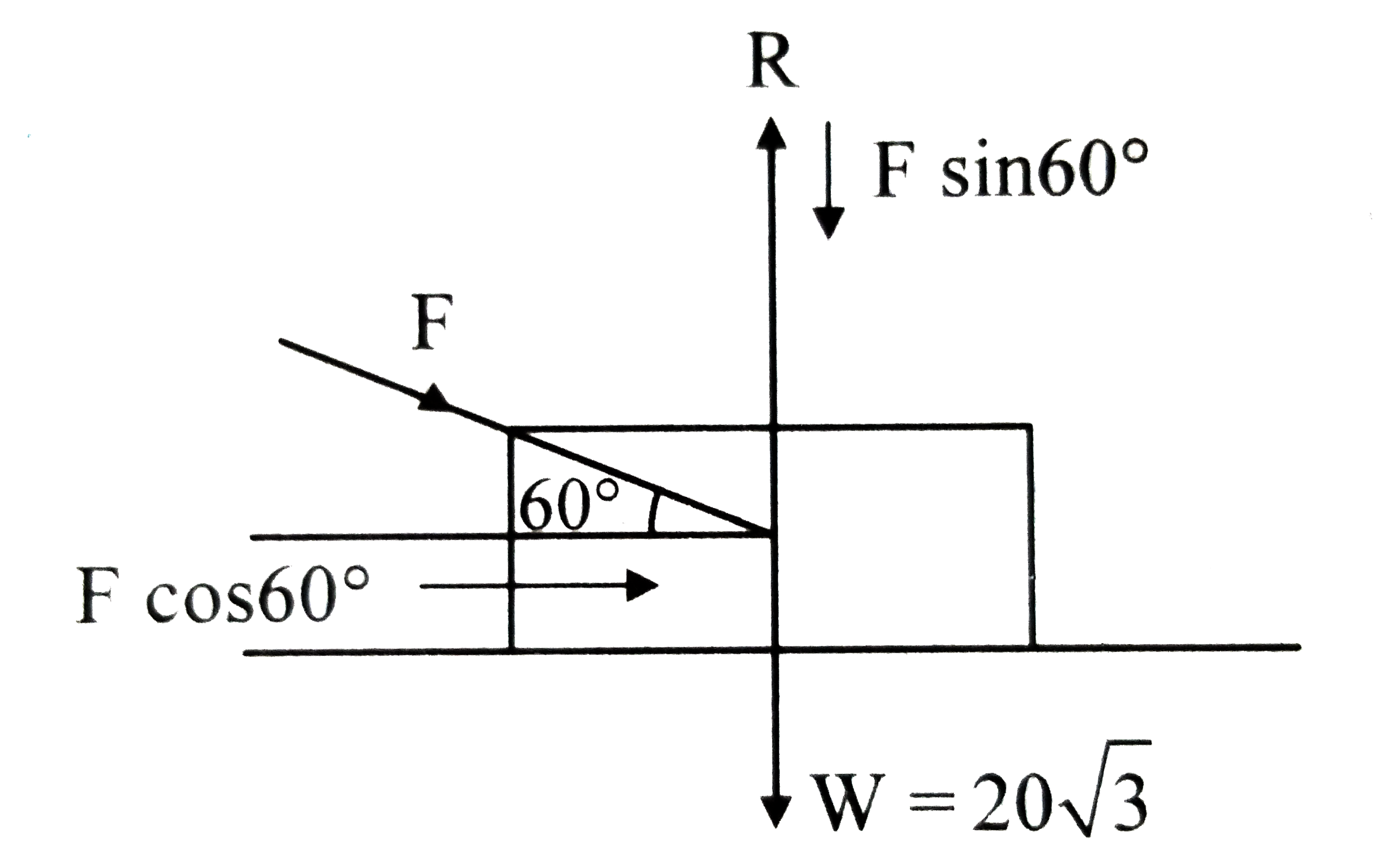
What is the maximum value of the force F such that the block shown in Fig. 7. 165. does not move ?

A

20 N

B

10 N

C

12 N

D

15 N

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

F cos $60^{\circ} = f = μ N \dots . . (i)$
and F sin $60^{\circ} + m g = N$

From Eqns. (i) and (ii) , F cos $60^{\circ} = μ [F {sin 60}^{\circ} + m g]$

$\Rightarrow F = \frac{μ m g}{{cos 60}^{\circ} - μ {sin 60}^{\circ}}$
$\frac{\frac{1}{2 \sqrt{3}} \times \sqrt{3} \times 10}{\frac{1}{2} - \frac{1}{2 \sqrt{3}} \times \frac{\sqrt{3}}{2}} = \frac{5}{\frac{1}{4}} = 20 N$

|

Updated on:21/07/2023

Class 11 PHYSICS FRICTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
What is the maximum value of the force F such that the block shown in the arrangement, does not move?

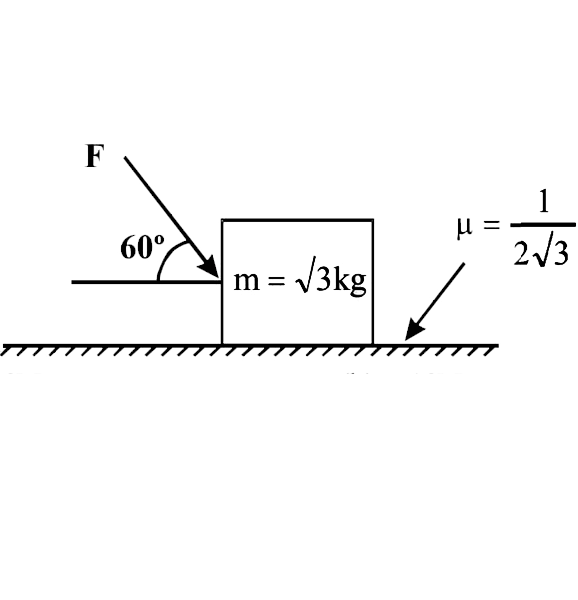A20N
B10N
C12N
D15N
Question 2 - Select One
What is the maximum value of the force F such that the block shown in the arrangement, does not move ?

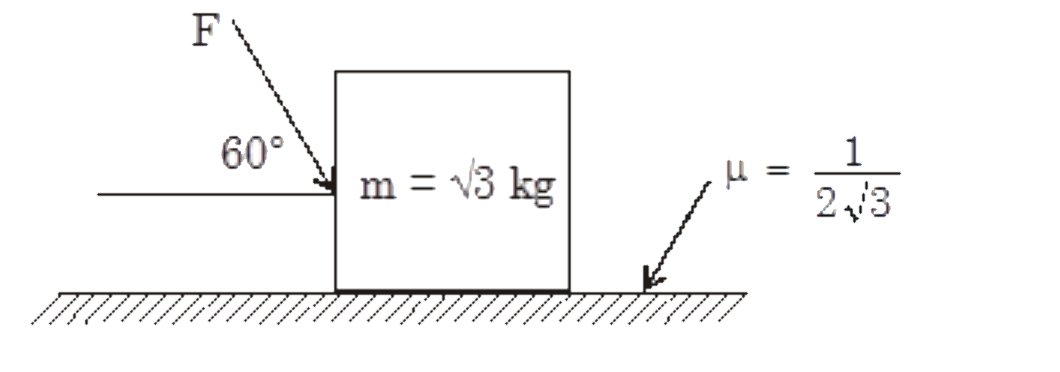A15 N
B5 N
C10 N
D20 N
Question 3 - Select One
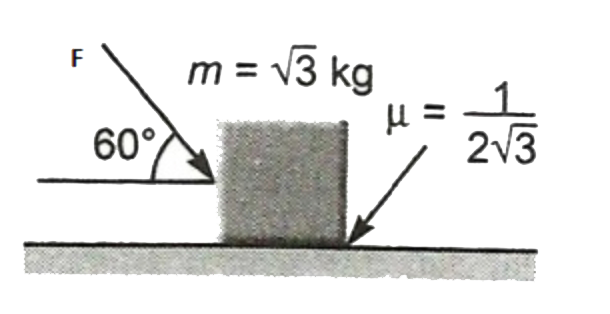
What is the maximum value of the force F such that the block shown in the arrangement, does not move question:

A20 N
B10 N
C12 N
D15 N

GRB PUBLICATION-FRICTION AND CIRCULAR MOTION -OBJECTIVE QUESTIONS ( only one choice is correct )

Home
Ask Doubt
Profile