In the parallelogram ABCD, ¯¯¯¯¯¯AD2−¯¯¯¯¯¯AB2 =
A
4¯¯¯¯¯¯AB. (orthogonal projection of ¯¯¯¯¯¯AD on ¯¯¯¯¯¯AB)
B
2¯¯¯¯¯¯AB. (orthogonal projection of ¯¯¯¯¯¯AD on ¯¯¯¯¯¯AB)
C
¯¯¯¯¯¯AC. (orthogonal projection of ¯¯¯¯¯¯BD on ¯¯¯¯¯¯AC)
D
2¯¯¯¯¯¯AC. (orthogonal projection of ¯¯¯¯¯¯BD on ¯¯¯¯¯¯AC)
Text Solution
The correct Answer is:C
|
Topper's Solved these Questions
- PRODUCTS OF VECTORSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PRODUCTS OF VECTORSEXERCISE - Exercise 1B Cross Product of Vectors
73 Videos
- PRODUCTS OF VECTORSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PRODUCTS OF VECTORSEXERCISE - Exercise 1C (Triple product of Vectors
112 Videos
- PRODUCTS OF VECTORSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PRODUCTS OF VECTORSEXERCISE - Exercise 2 (Special Type Questions) SET -D
14 Videos
- PROBABILITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PROBABILITYEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) SET-4
5 Videos
- PROPERTIES OF TRIANGLES BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - PROPERTIES OF TRIANGLES EXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) Set-4
10 Videos
Similar Questions
Knowledge Check
In parallelogram ABCD, if ¯¯¯¯¯¯AB=¯aand¯¯¯¯¯¯AD=¯b, then the diagonals interm of ¯aand¯b are
A¯a+¯b,¯a−¯bB¯a,¯bC¯a+¯b,¯b−¯aD2¯a,2¯bIf ¯¯¯¯¯¯AC=3ˆi+ˆj−2ˆk,¯¯¯¯¯¯DB=ˆi−3ˆj−4ˆk are the vectors along the diagonals of a parallelogram ABCD and ¯¯¯¯¯¯AE=ˆi+2ˆj+3ˆk is another vector, then the volume of the paralleloP1ped whose co-terminous edges are represented by the vectors ¯¯¯¯¯¯AB,¯¯¯¯¯¯AD,¯¯¯¯¯¯AE is
A2cu. UnitsB10cu. UnitsC6cu. UnitsD12cu. UnitsIn quadrilateral ABCD, if ¯¯¯¯¯¯AB=¯¯¯¯¯¯CD, then it is
AtrapeziumBparallelogramCkiteDrhombus
In triangle ABC, if 2¯¯¯¯¯¯AC=3¯¯¯¯¯¯CB, then 2¯¯¯¯¯¯OA+3¯¯¯¯¯¯OB=
View SolutionIf the points A(¯a),B(¯b),C(¯c) are collinear and 3¯a+2¯b−5¯c=¯0, then
View SolutionIf the points A(¯a),B(¯b),C(¯c) are collinear and 2¯a+3¯b−5¯c=¯0, then
View SolutionIn the figure given below , ¯¯¯¯¯¯ED∣∣∣∣¯¯¯¯¯¯ABand¯¯¯¯¯¯EF∣∣∣∣¯¯¯¯¯¯BC. If ∠FED=40∘and∠DEC=20∘ , then the angle made by ¯¯¯¯¯¯BC at the centre is _______.
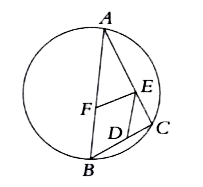
View SolutionIf ¯a,¯band¯c are unit vectors perpendicular to each other, the [¯a¯b¯c]2=
View Solution

