f:R−{0}→R given by f(x)=1x−2e2x−1
can be made continuous at x = 0 by defining f(0) as Solution in Telugu
2
−1
0
1
The correct Answer is:D
Step by step video, text & image solution for f : R - {0} to R " given by " f(x) = (1)/(x) - (2)/(e^(2x)-1) can be made continuous at x = 0 by defining f(0) as by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 2(Special Type Questions)10videos
LIMITS AND CONTINUITY
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - SET-3Match The Following2videos
LIMITS AND CONTINUITY
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 1B( One Sided Limits)71videos
LIMITS AND CONTINUITY
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) (SET - 4)5videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LINEAR PROGRAMMING [APPENDIX-2]EXERCISE - Exercise -133videos
LINEAR PROGRAMMING [APPENDIX-2]
Similar Questions
I. Ltx→0(1+x)2−(1−x)2−2(1+x)3−(1−x)3−3
II. If f(x)=4−7x3x+4andLtx→2f(x)=l,Ltx→0f(x)=m then the equation whose roots are 1/l, 1/m is x2−1=003:15View SolutionMatch the following
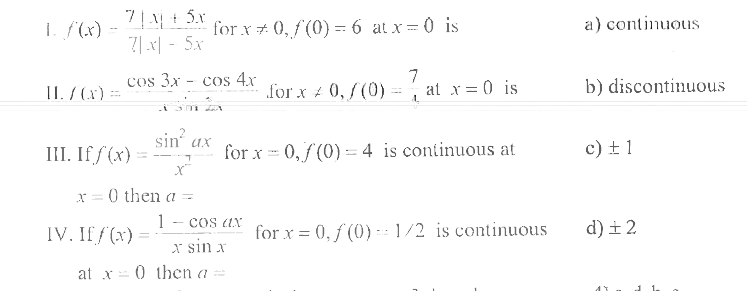 04:24View Solution
04:24View SolutionLtx→0√x=
03:15View SolutionA : The maximum value of f(x)=Sin−1x+Cos−1x−Tan−1x is 3π/4
R : Sin−1x+Cos−1x=π/2∀x∈RView SolutionIf 2x+y≥10,x+2y≥10, then the lesat value of F=x+y is
View SolutionThe function f:R-{0}rarr R given by f(x)=(1)/(x)-(2)/(e^(2)x-1) can be made continuous at x=0 by defining f(0) as
03:47View Solutionf(0) को निम्नलिखित प्रकार से परिभाषित करके फलन f(x)=1x−2e2x−1 को बिंदु x=0 पर सतत बनाया जा सकता हैं:
02:22View SolutionThe function f : R - {0} to R given by f(x) = 1/x - 2/(e^(2x)-1) can be made continuous at x = 0 by defining f(0) as
View SolutionA: f(x)=11+e1/x(x≠0)andf(0)=0 is right continuous at x=0
R: Ltx→011+e1/x=004:24View SolutionIf f is a continuous and differentiable funciton in x∈(0,1) such that Σ10r=0(f(x+r)−|ex−r−1|)=0, then ∫110f(x)dx is
03:34View SolutionIs f defined by f(x)={sin2xxifx≠01ifx=0 continuous at 0?
04:02View SolutionThe function f:R/{0}→R given by :
f(x)=1x−2e2x−1 can be made continuous at x = 0 by defining f(0) as :03:32View SolutionThe function f:R~{0}→R given by f(x)=tanx−xx−sinx can be made continuous at x=0 by defining f(0) as
02:27View SolutionIf the function f:R− {0}→R given by f(x)=1x−2e2x−1 is continuous at x=0, then find the value of f(0)
03:09View SolutionThe function f:R~{0}→R given by f(x)=1x−2e2x−1 can be made continuous at x = 0 by defining f(0) as (1) 2 (2) −1 (3) 0 (4) 1
04:57View Solution

