Which of the following could be the four angles of a parallelogram?
(I) 50∘,130∘,50∘,130∘
(II) 125∘,50∘,125∘,60∘
I only
II only
I and II only
None of them
The correct Answer is:A
To determine which of the given sets of angles could represent the angles of a parallelogram, we need to recall the properties of a parallelogram. In a parallelogram, opposite angles are equal, and the sum of the angles in any quadrilateral is 360 degrees.
Let's analyze the options step by step.
Step 1: Analyze the first option
The first option gives us the angles: 50∘,130∘,50∘,130∘.
- Check for opposite angles:
- Angle A = 50∘ and Angle C = 50∘ (opposite angles)
- Angle B = 130∘ and Angle D = 130∘ (opposite angles)
- Check the sum of angles:
50∘+130∘+50∘+130∘=360∘
Since both conditions are satisfied (opposite angles are equal and the sum of angles is 360∘), the first option is valid.
Step 2: Analyze the second option
The second option gives us the angles: 125∘,50∘,125∘,60∘.
- Check for opposite angles:
- Angle A = 125∘ and Angle C = 125∘ (opposite angles)
- Angle B = 50∘ and Angle D = 60∘ (not equal)
Since the opposite angles are not equal (Angle B and Angle D), this option does not satisfy the properties of a parallelogram.
Conclusion
Based on the analysis:
- The first option 50∘,130∘,50∘,130∘ is valid.
- The second option 125∘,50∘,125∘,60∘ is not valid.
Thus, the correct answer is that only the first option could represent the angles of a parallelogram.
The first option gives us the angles: 50∘,130∘,50∘,130∘.
- Angle A = 50∘ and Angle C = 50∘ (opposite angles)
- Angle B = 130∘ and Angle D = 130∘ (opposite angles)
50∘+130∘+50∘+130∘=360∘
The second option gives us the angles: 125∘,50∘,125∘,60∘.
- Angle A = 125∘ and Angle C = 125∘ (opposite angles)
- Angle B = 50∘ and Angle D = 60∘ (not equal)
Based on the analysis:
- The first option 50∘,130∘,50∘,130∘ is valid.
- The second option 125∘,50∘,125∘,60∘ is not valid.
Similar Questions
Knowledge Check
Which of the following is equal to i50+i0?
A1B2C−1D0Which of the following integers is closest to √502?
A3B4C5D13Which of the following will show optical activity ?
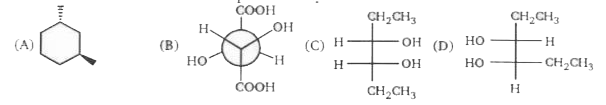
(E) 50/50 mixture of C and DAA, D and EBA and E onlyCB, C and DDAll except C
Write the absolute value of each of the following:
(i) 14
(ii) −25
(iii) 0
(iv) −125
View SolutionWrite each of the following divisions as fractions: (i)6÷3 (ii) 25÷5 (iii) 125÷50 (iv) 55÷11
View SolutionThe sum of two opposite angles of a parallelogram is 1300. Find all the angles of the parallelogram.
View SolutionWrite the following numbers in words in the Indian system of numeration: (i)25,05,05,500(ii)5,50,50,050 (iii)5,03,04,012
View SolutionWhich of the following holds true?
(A) 10150−10050>9950
(B) 10150−9950<10050
(C) (1000)1000>(1000)999
(D) (10001)999<(1000)1000
View Solution
