Column AThe average ages of the players on team A and team B , are 20 and 30 years, respectively. The Average age of the players of the teams togeter is 26Column BThe number of players on team AThe number of players on team B
If column A is larger
If column B is larger
If the columns are equal
If there is not enough information to decide
The correct Answer is:B
To solve the problem, we need to compare the number of players on team A and team B based on the average ages provided. Here’s a step-by-step breakdown of the solution:
Step 1: Define Variables
Let:
- a = number of players in team A
- b = number of players in team B
Step 2: Write Total Ages
The average age of players in team A is 20 years, so the total age of players in team A is:
Total Age of Team A=20a
The average age of players in team B is 30 years, so the total age of players in team B is:
Total Age of Team B=30b
Step 3: Write Combined Average Age
The average age of players from both teams combined is given as 26 years. Therefore, we can express the combined average age as:
Combined Average Age=20a+30ba+b
Setting this equal to 26 gives us the equation:
20a+30ba+b=26
Step 4: Clear the Fraction
To eliminate the fraction, we multiply both sides by a+b:
20a+30b=26(a+b)
Step 5: Expand and Rearrange
Expanding the right side:
20a+30b=26a+26b
Now, rearranging the equation to group terms involving a and b:
20a+30b−26a−26b=0
This simplifies to:
−6a+4b=0
Step 6: Solve for the Ratio
Rearranging gives:
4b=6a
Dividing both sides by 2:
2b=3a
Thus, we find the ratio of b to a:
ba=32=1.5
Step 7: Compare the Values
Since ba=1.5, it indicates that b>a. Therefore, the number of players in team B is greater than the number of players in team A.
Conclusion
Since column A represents the number of players in team A and column B represents the number of players in team B, we conclude that:
Column B is larger than Column A.
Thus, the correct option is option 2.
---
Let:
- a = number of players in team A
- b = number of players in team B
The average age of players in team A is 20 years, so the total age of players in team A is:
Total Age of Team A=20a
The average age of players in team B is 30 years, so the total age of players in team B is:
Total Age of Team B=30b
The average age of players from both teams combined is given as 26 years. Therefore, we can express the combined average age as:
Combined Average Age=20a+30ba+b
Setting this equal to 26 gives us the equation:
20a+30ba+b=26
To eliminate the fraction, we multiply both sides by a+b:
20a+30b=26(a+b)
Expanding the right side:
20a+30b=26a+26b
Now, rearranging the equation to group terms involving a and b:
20a+30b−26a−26b=0
This simplifies to:
−6a+4b=0
Rearranging gives:
4b=6a
Dividing both sides by 2:
2b=3a
Thus, we find the ratio of b to a:
ba=32=1.5
Since ba=1.5, it indicates that b>a. Therefore, the number of players in team B is greater than the number of players in team A.
Since column A represents the number of players in team A and column B represents the number of players in team B, we conclude that:
Column B is larger than Column A.
Thus, the correct option is option 2.
Similar Questions
Knowledge Check
- Question 1 - Select One
Column A Column BThe average of three number if the greatest is 20The average of the numbers if the greatest is 2
AIf column A is largerBIf column B is largerCIf the columns are equalDIf there is not enough information to decide - Question 2 - Select One
Column A Column BThe last digit in the average of the numbers 13 and 23The last digit in the average of the numbers 113 and 123
AIf column A is largerBIf column B is largerCIf the columns are equalDIf there is not enough information to decide - Question 3 - Select One
There are 12 players on a basketball team. Before a game, both ankles of each player are taped. Each roll of tape will tape three ankles. Which product can be used to determine the number of rolls of tape needed to tape the player's ankles?
A12 players⋅1 player2 ankles⋅3 ankles1 rollB12 players⋅2 ankles⋅3 rolls1 ankleC12 players⋅1 roll3 players⋅3 anklesrollD12 players⋅2 ankles1 player⋅1 roll3 ankles
The batting scores of two cricket players A and B in 10 innings are as follows:
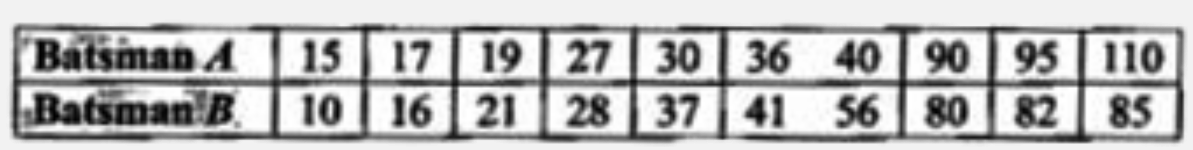
Which of the players is consistent.View SolutionIn a college team there are 15 players of whoom 3 are teachers . In how many ways can a team of 11 players be selected so as to include only one teachers
View SolutionIn a circket charmpionship there are 36 matches. The number of teams if each plays one match with other, is
View SolutionColumn AThe average of a set of six positive number is 30Column BThe average of the number in the set after replacing the smallest number in the set with 0The average of the remaining number in the set after removing the smallest number from the set
View SolutionColumn AThe arithmetic mean (average) of the number a and b is 17. The geometric mean of the numbers a and b is 8. The geometric mean of two numbers is defined to be the square root of their productColumn Bab
View Solution

