If m√27=33mand4m>1, then what is the value of m?
−1
−1/4
0
1
The correct Answer is:D
To solve the equation √(m)27=33m under the condition 4m>1, we can follow these steps:
Step 1: Analyze the inequality 4m>1
We know that 4m>1 implies:
m>0
This is because any positive base raised to a power greater than zero is greater than 1.
Step 2: Rewrite the m-th root of 27
The m-th root of 27 can be expressed as:
√(m)27=271m
Now, we can rewrite 27 in terms of base 3:
27=33
Thus, we have:
√(m)27=(33)1m=33m
Step 3: Set the equation
Now we can set the equation from the problem:
33m=33m
Step 4: Equate the exponents
Since the bases are the same, we can equate the exponents:
3m=3m
Step 5: Solve for m
To solve for m, we can multiply both sides by m (noting that m>0):
3=3m2
Dividing both sides by 3 gives:
1=m2
Step 6: Find the values of m
Taking the square root of both sides, we find:
m=1orm=−1
However, since we established earlier that m>0, we discard m=−1.
Conclusion
Thus, the only solution is:
m=1
Final Answer
The value of m is 1.
---
We know that 4m>1 implies:
m>0
This is because any positive base raised to a power greater than zero is greater than 1.
The m-th root of 27 can be expressed as:
√(m)27=271m
Now, we can rewrite 27 in terms of base 3:
27=33
Thus, we have:
√(m)27=(33)1m=33m
Now we can set the equation from the problem:
33m=33m
Since the bases are the same, we can equate the exponents:
3m=3m
To solve for m, we can multiply both sides by m (noting that m>0):
3=3m2
Dividing both sides by 3 gives:
1=m2
Taking the square root of both sides, we find:
m=1orm=−1
However, since we established earlier that m>0, we discard m=−1.
Thus, the only solution is:
m=1
The value of m is 1.
Similar Questions
Knowledge Check
- Question 1 - Select One
If 7m−√3=√3m+42m, what is the value of m ?
A−3.464B−1.978C−0.918D1.978
The mid-point of the segment joining (3m, 6) and (-4, 3n) is (1, 2m, -1). Find the values of m and n.
View SolutionIf the tangent at any point P(4m2,8m3) of y2=x3 is a normal also to the curve , then the value of 27m2 is ...........
View SolutionIf m=3√125 and n=3√64 , find the value of m−n−1m2+mn+n2.
View SolutionThree blocks m1,m2, and m3 are arranged as shown in fig. if m2=5kg and m3=8kg, at what value of m1 will 8kg mass be at rest?
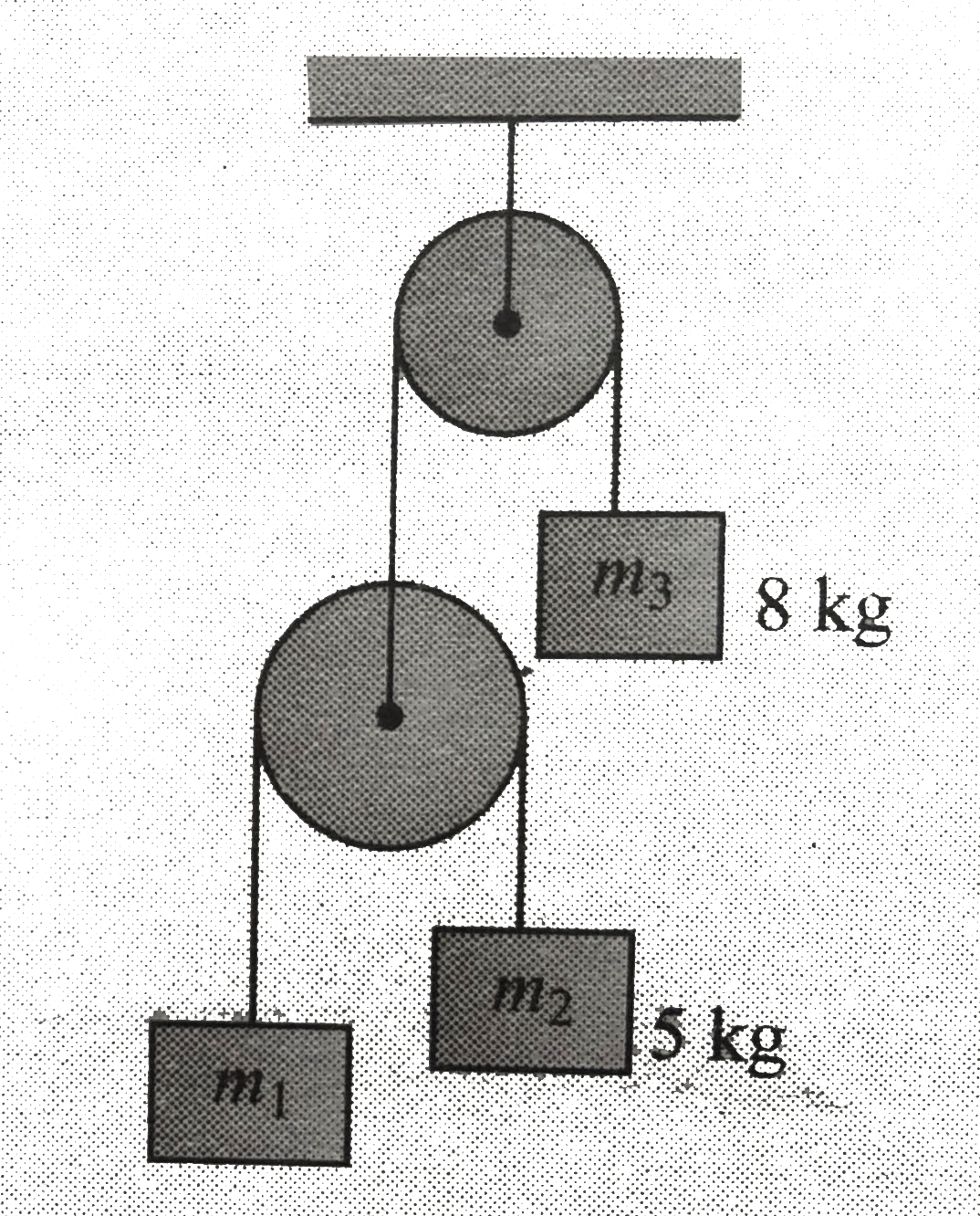 View Solution
View SolutionIf M and m are the maximum and minimum values of yx for pair of real numbers (x, y) which satisfy the equation (x−3)2+(y−3)2=6, then the value of 1M+1m is
View SolutionIf m1,m2 are the slopes of the two tangents that are drawn from (2,3) to the parabola y2=4x , then the value of 1m1+1m2 is
View SolutionIf λ be the ratio of the roots of the quadratic equation in x, 3m2x2+m(m−4)x+2=0, then the least value of m for which λ+1λ=1, is k−3√2. The value of k is __________.
View Solution

