Question

Phosgene is a poisonous gas that dissociates at high temperature into two other poisonous gases, carbon monoxide and chlorine. The equilibrium constant $K_p$= 0.0041 atm at 600K. Find the equilibrium composition of the system after 0.124 atm of $COC{l}_2$ initially is allowed to reach equilibrium at this temperature.

Explanation

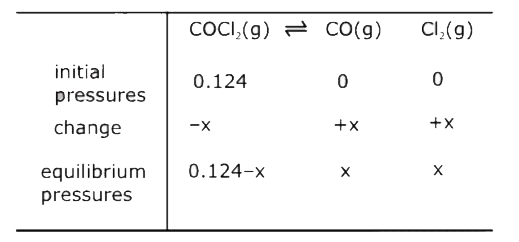
Substitution of the equilibrium pressures into the equilibrium expression gives
`(x^(2))/(0.124-x)=0.0041`
This expression can be rearranged into standard polynomial form `x^(2)+0041x–0.00054=0` and solved by the quadratic formula, but we will simply obtain an approximate solution by iteration.
Because the equilibrium constant is small, we know that x will be rather small compared to 0.124, so the above relation can be approximated by
`(x^(2))/(0.124)=0.0041`
which gives x=0.025. To see how good this is, substitute this value of x into the denominator of the original equation and solve again:
`(x^(2))/(0.124-0.0225)=(x^(2))/(0.102)=0.0041`
This time, solving for x gives 0.0204. Iterating once more, we get
`(x^(2))/(0.124-0.0204)=(x^(2))/(0.104)=0.0041`
and x = 0.0206 which is sufficiently close to the previous to be considered the final result. The final partial pressures are then 0.104 atm for `COCl_(2)`, and 0.0206 atm each for CO and `Cl_(2)`.
Note:
Using the quadratic formula to find the exact solution yields the two roots –0.0247 (which we ignore) and 0.0206, which show that our approximation is quite good. Note: using the quadraticformula to find the exact solution yields the two roots –0.0247 (which we ignore) and 0.0206, which show that our approximation is quite good.