The minimum mass of mixture of A2 and B4 required to produce at least 1 kg of each product is :
(Given At. mass of ‘A’ = 10, At mass of ‘B’ = 120)
5A2+2B4→2AB2+4A2B
2120 gm
1060 gm
560 gm
1660 gm
The correct Answer is:A
To find the minimum mass of the mixture of A2 and B4 required to produce at least 1 kg of each product AB2 and A2B, we will follow these steps:
Step 1: Write the balanced chemical equation
The balanced chemical equation is:
5A2+2B4→2AB2+4A2B
Step 2: Determine the molar masses of the reactants and products
- Molar mass of A2:
Molar mass of A2=2×Atomic mass of A=2×10=20 g/mol
- Molar mass of B4:
Molar mass of B4=4×Atomic mass of B=4×120=480 g/mol
- Molar mass of AB2:
Molar mass of AB2=Atomic mass of A+2×Atomic mass of B=10+2×120=250 g/mol
- Molar mass of A2B:
Molar mass of A2B=2×Atomic mass of A+Atomic mass of B=2×10+120=140 g/mol
Step 3: Calculate the required moles of products
To produce at least 1 kg (1000 g) of each product:
- For AB2:
Moles of AB2=1000 g250 g/mol=4 moles
- For A2B:
Moles of A2B=1000 g140 g/mol≈7.14 moles
Step 4: Determine the limiting reactant
From the balanced equation:
- 2 moles of AB2 are produced from 5 moles of A2 and 2 moles of B4.
- Therefore, for 4 moles of AB2:
Moles of A2=52×4=10 moles
Moles of B4=2×2=4 moles
Step 5: Calculate the mass of reactants required
- Mass of A2:
Mass of A2=10 moles×20 g/mol=200 g
- Mass of B4:
Mass of B4=4 moles×480 g/mol=1920 g
Step 6: Calculate the total mass of the mixture
Total mass=Mass of A2+Mass of B4=200 g+1920 g=2120 g
Final Answer
The minimum mass of the mixture of A2 and B4 required to produce at least 1 kg of each product is 2120 grams.
---
The balanced chemical equation is:
5A2+2B4→2AB2+4A2B
- Molar mass of A2:
Molar mass of A2=2×Atomic mass of A=2×10=20 g/mol
- Molar mass of B4:
Molar mass of B4=4×Atomic mass of B=4×120=480 g/mol
- Molar mass of AB2:
Molar mass of AB2=Atomic mass of A+2×Atomic mass of B=10+2×120=250 g/mol
- Molar mass of A2B:
Molar mass of A2B=2×Atomic mass of A+Atomic mass of B=2×10+120=140 g/mol
To produce at least 1 kg (1000 g) of each product:
- For AB2:
Moles of AB2=1000 g250 g/mol=4 moles
Moles of A2B=1000 g140 g/mol≈7.14 moles
From the balanced equation:
- 2 moles of AB2 are produced from 5 moles of A2 and 2 moles of B4.
- Therefore, for 4 moles of AB2:
Moles of A2=52×4=10 moles
Moles of B4=2×2=4 moles
- Mass of A2:
Mass of A2=10 moles×20 g/mol=200 g
Mass of B4=4 moles×480 g/mol=1920 g
Total mass=Mass of A2+Mass of B4=200 g+1920 g=2120 g
The minimum mass of the mixture of A2 and B4 required to produce at least 1 kg of each product is 2120 grams.
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - MOLE CONCEPTEXERCISE - EXERCISE - 2 (LEVEL - II)17videos
MOLE CONCEPT
- BOOK - MOTIONCHAPTER - MOLE CONCEPTEXERCISE - EXERCISE - 360videos
MOLE CONCEPT
- BOOK - MOTIONCHAPTER - MOLE CONCEPTEXERCISE - EXERCISE - 164videos
MOLE CONCEPT
- BOOK - MOTIONCHAPTER - METALLURGYEXERCISE - EXERCISE 4 (LEVEL - II)17videos
METALLURGY
- BOOK - MOTIONCHAPTER - NOMENCLATURE OF ORGANIC COMPOUNDSEXERCISE - PREVIOUS YEAR8videos
NOMENCLATURE OF ORGANIC COMPOUNDS
Similar Questions
Which would requires a greater force: accelerating a 2kg mass at 5m/s2 or a 4kg mass at 2m/s2?
View SolutionObjective question (only one correct).
I. Which of the following has least mass?
a. 1 mol of S b. 3×1023 atom of C
c. 2g atom of nitrogen d. 7.0gofAg
ii. The simplest formula of a compound containing 50% of element A (atomic mass 10) and 50% of element B (atomic mass 20) is:
a. AB b. A2B c. A2B3 d. AB3View Solution
Knowledge Check
- Question 1 - Select One
The minimum mass of mixture of A2 and B2 required to produce at least 1 kg of each product is : (Given At. Mass of 'A' = 10 : At. Mass of 'B' = 120 )
A2120 gmB1060 gmC560 gmD1660 gm - Question 2 - Select One
Two substance A2(g) and B2(g) are allowed to react to form either of A2B2(l),A2B4(g) or both depending on relative amount.
Answer the following the following question, if 75 g A2 and 200gB2 are mixed and no reactant is left.
[Given :At mass of A =25 , B=50]
Now A2B4 is transferred to another container completey . At high temperature it is completely dissociated in its gaseous elements A2 and B2 . Find average molecular weight of gaseous mixture:A1253B2503C125D250 - Question 3 - Select One or More
In the reaction:
A+B2→AB2
Find out in which option(s) B2 is limiting reagent. [Molar mass : MA=10gmole−1,MB=20gmol−1]A300 atoms of A + 200 molecules of B2B2 mole A+3 gram-atom of BC100 mole A atoms +100 mole B atomsD5 gram-atom of A + 12.5 gram molecule of B2
A2+2B2→A2B4
32A2+2B2→A3B4
Two substance A2&B2 react in the above manner when A2 is limited it gives A2B4 in excess gives A3B4.A2B4 can be converted to A3B4 when reacted with A2. Using this information calculate the composition of the final mixture when the mentioned amount of A%B are taken :c
(a)4 mole A2&4mole B2
(b)12 moles A2&2 moles B2
(c)1.25 moles A2&2 moles B2View SolutionA2+2B2→A2B4and32A2+2B2→A3B4
Two substance A2&B2 are allowed to react completely to from A2B4&A3B4 mixture of leaving none of the reactants. Using this information calculate the composition of final mixture when mentioned amount of A2&B2 are taken.
(i) If 54 moles of A2&2 moles of B2 is taken
(ii) If 72 moles of A2&6 moles of B2 is taken
(iii) If 4 moles of A2&6 moles of B2 is takenView SolutionFactorise the using the identity a2−2ab+b2=(a−b)2.
4a2−4ab+b2View SolutionTwo substance A2(g) and B2(g) are allowed to react to form either of A2B2(l),A2B4(g) or both depending on relative amount.
Answer the following the following question, if 75 g A2 and 200gB2 are mixed and no reactant is left.
[Given :At mass of A =25 , B=50]
What is the volume of all the gases present in the mixture after completion of reaction at 760 mm of Hg and 273∘ C?View SolutionThree masses A, B and C are arranged as shown in the figure, the mass of 'A' is 2 times the mass of 'B' and the mass of B is 10 kg. If the force of attraction between A and B is equal to the force of attraction between B and C, then the mass of C is _________ kg.
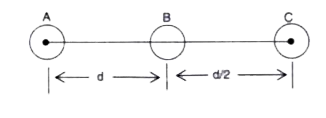 View Solution
View Solution

