If I1,I2,I3 are the centers of escribed circles of ABC, show that are of I1I2I3=abc2r.
Step by step video & image solution for If I_1, I_2, I_3 are the centers of escribed circles of A B C , show that are of I_1I_2I_3=(a b c)/(2r)dot by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
The moment of inertia of thin square plate ABCD of uniform thickness about an axis passing through the center O and perpendicular to the plane of the plate is
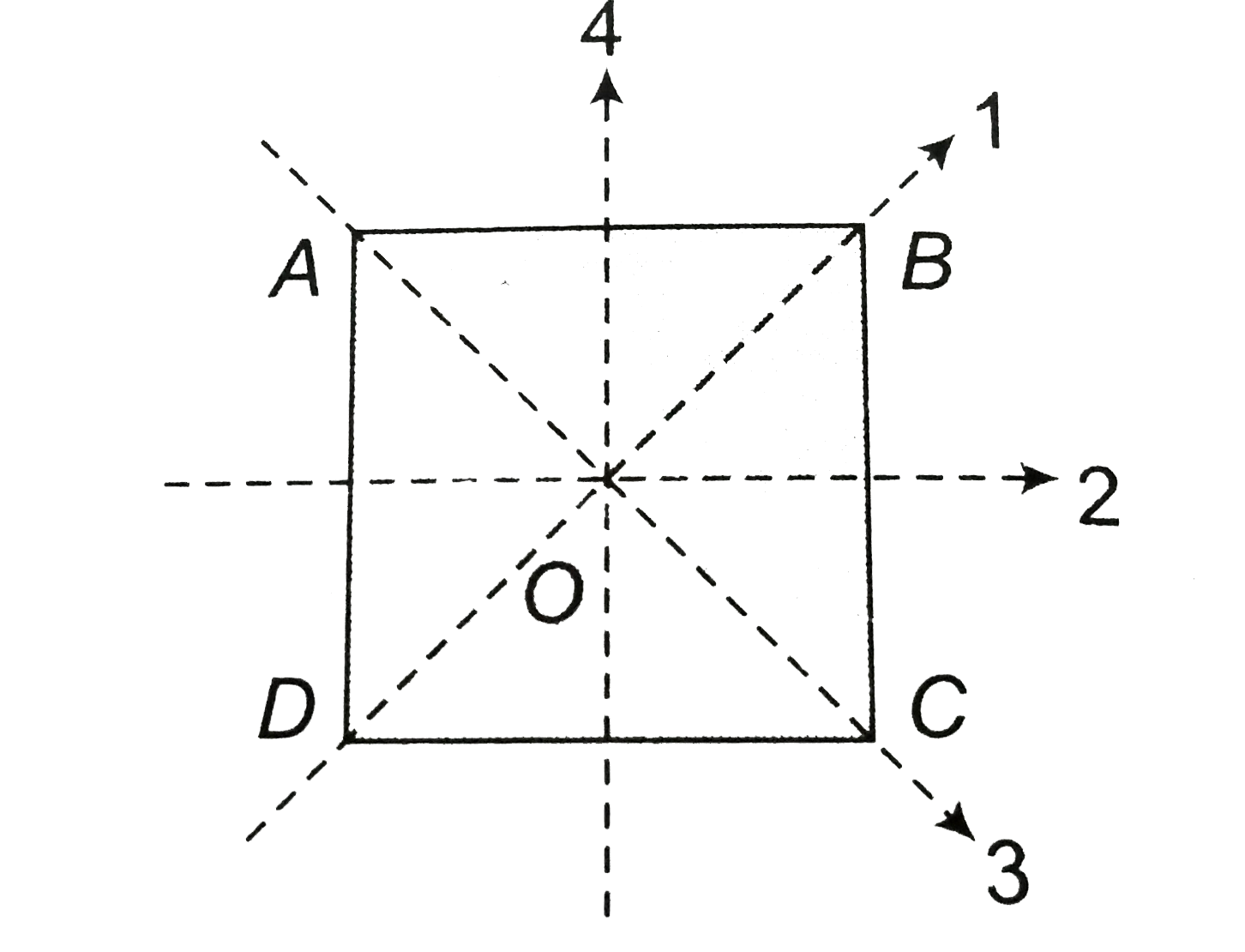
(i) I1+I2
(ii) I2+I4
(iii) I1+I3
(iv) I1+I2+I3+I4
where I1, I2, I3 and I4 are repectively moments of inertia about axes 1, 2, 3 and 4 which are in the plane of the planeA(i),(ii)B(i), (ii), (iii)C(ii), (iii)D(i), (iii)
In a triangle ABC I1,I2,I3 are excentre of triangle then show that II1.II2.II3=16R2r.
View SolutionIf I is the incenter of ABCandR1,R2,andR3 re, respectively, the radii of the circumcircles of the triangles IBC,ICAandIAB, then prove that R1R2R3=2rR2.
View SolutionIn a ΔABC, if (II1)2+(I2I3)2=λR2, where I denotes incentre, I1,I2andI3 denote centres of the circles escribed to the sides BC, CA and AB respectively and R be the radius of the circum circle of ΔABC. Find λ.
View SolutionIn a ΔABC, if (II1)2+(I2I3)2=λR2, where I denotes incentre, I1,I2andI3 denote centres of the circles escribed to the sides BC, CA and AB respectively and R be the radius of the circum circle of ΔABC. Find λ.
View SolutionA semicircular ring has mass m and radius R as shown in figure. Let I1,I2,I3andI4 be the moments of inertia of the four axes as shown. Axis 1 passes through centre and is perpendicular to plane of ring. Then, match the following columns.
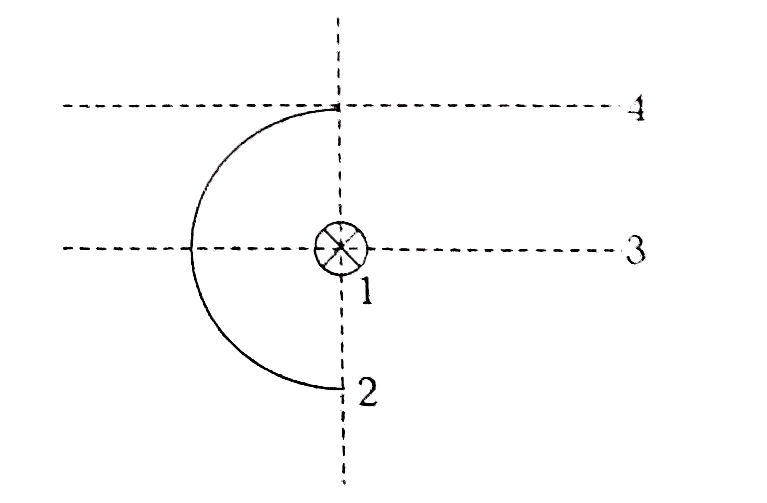
Column-IColumn-II(A)I1(p)mR22(B)I2(q)(3/2)mR2(C)I3(r)mR2(D)I4(s)Data is insufficient
View SolutionFind the Radius of escribed circle (i)r1=Δs−a=stan(A2)=4Rsin(A2)Cos(B2)Cos(C2)
View SolutionIf A2−A+I=0, then the inverse of A is: (A) A+I (B) A (C) A−I (D) I−A
View Solution
