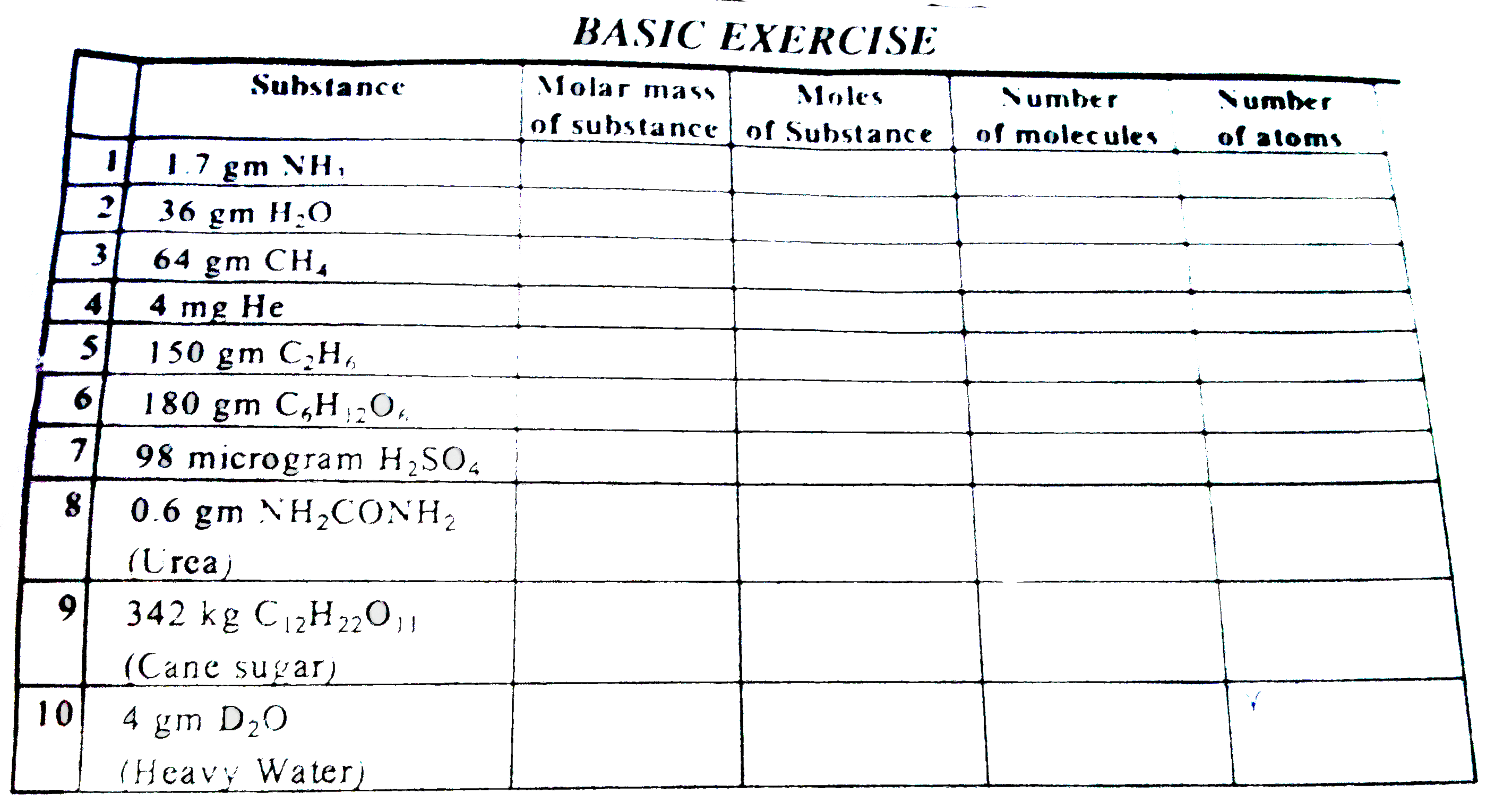
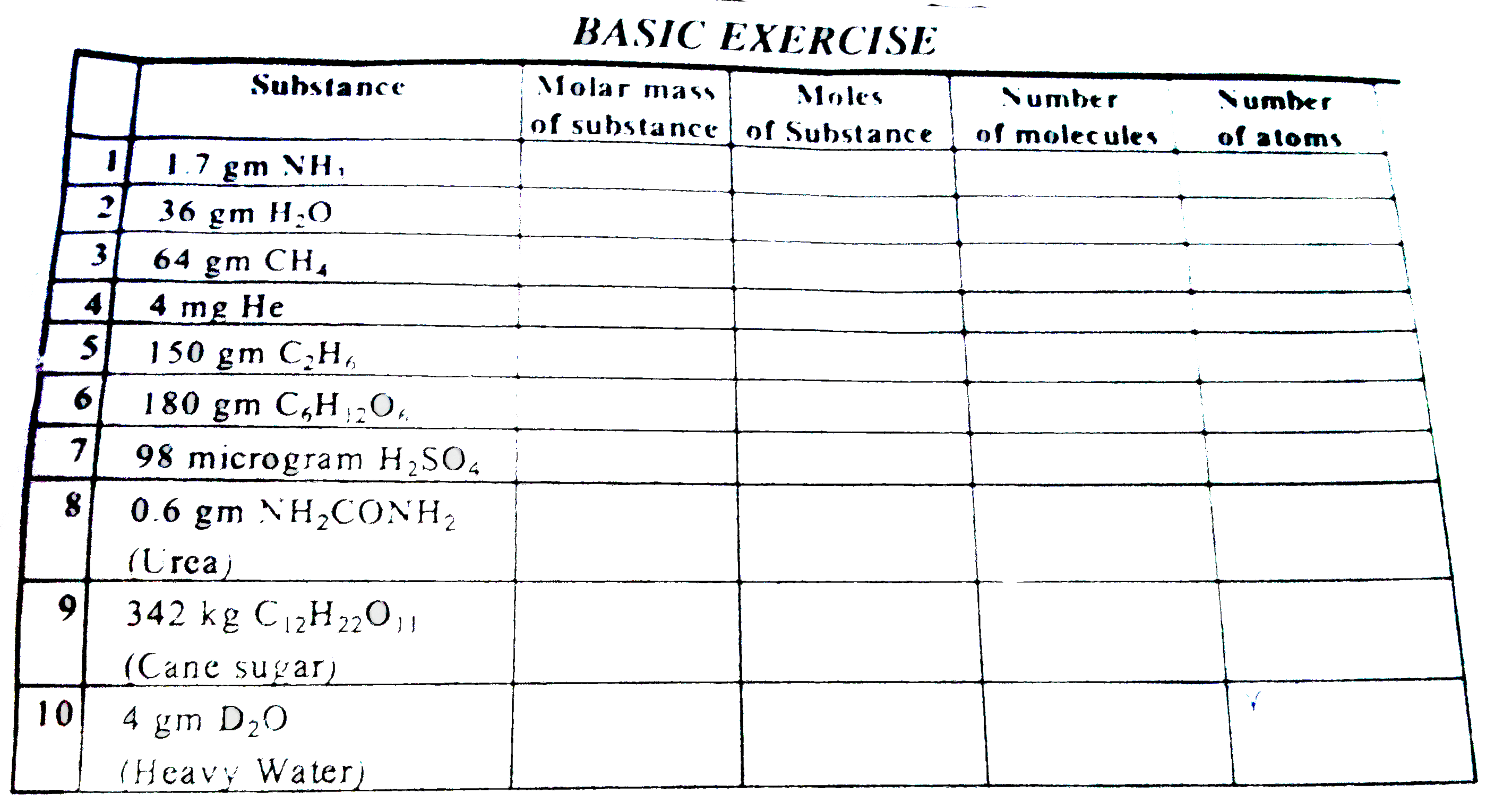
A metal (M∘=100 gm/mole) having density 10 gm/cm3 forms a cubic lattice. If radius of an atom is 1.4 Å find packing fraction. [NA=6×1023 perm ole].
0.74
0.69
0.52
0.90
To find the packing fraction of the metal, we will follow these steps:
Step 1: Calculate the Volume of the Atom
The volume V of a single atom (assuming it is spherical) can be calculated using the formula for the volume of a sphere:
V=43πr3
Given that the radius r=1.4 Å (which is 1.4×10−8 cm), we can substitute this value into the formula.
V=43π(1.4×10−8 cm)3
Step 2: Calculate the Volume of the Unit Cell
For a cubic lattice, the volume of the unit cell Vcell can be calculated using the formula:
Vcell=a3
where a is the edge length of the cube. In a simple cubic lattice, the relationship between the edge length a and the radius r of the atom is given by:
a=2r
Substituting the value of r:
a=2×(1.4×10−8 cm)=2.8×10−8 cm
Now we can calculate the volume of the unit cell:
Vcell=(2.8×10−8 cm)3
Step 3: Calculate the Number of Atoms in the Unit Cell
In a simple cubic lattice, there is 1 atom per unit cell (since there is one atom at each corner contributing 18 of an atom from each of the 8 corners).
Step 4: Calculate the Packing Fraction
The packing fraction PF is defined as the ratio of the total volume of the atoms in the unit cell to the volume of the unit cell:
PF=Z⋅VatomVcell
Where Z is the number of atoms per unit cell (which is 1 for a simple cubic lattice).
Substituting the values we calculated:
PF=1⋅43π(1.4×10−8)3(2.8×10−8)3
Step 5: Simplify and Calculate
Now we can simplify the expression:
PF=43π(1.4)3(2.8)3
Calculating the numerical values:
PF=43π(2.744)21.952
PF≈11.4821.952≈0.523
Conclusion
Thus, the packing fraction of the metal is approximately 0.523.
The volume V of a single atom (assuming it is spherical) can be calculated using the formula for the volume of a sphere:
V=43πr3
Given that the radius r=1.4 Å (which is 1.4×10−8 cm), we can substitute this value into the formula.
For a cubic lattice, the volume of the unit cell Vcell can be calculated using the formula:
Vcell=a3
where a is the edge length of the cube. In a simple cubic lattice, the relationship between the edge length a and the radius r of the atom is given by:
a=2r
Substituting the value of r:
a=2×(1.4×10−8 cm)=2.8×10−8 cm
Now we can calculate the volume of the unit cell:
Vcell=(2.8×10−8 cm)3
In a simple cubic lattice, there is 1 atom per unit cell (since there is one atom at each corner contributing 18 of an atom from each of the 8 corners).
The packing fraction PF is defined as the ratio of the total volume of the atoms in the unit cell to the volume of the unit cell:
PF=Z⋅VatomVcell
Where Z is the number of atoms per unit cell (which is 1 for a simple cubic lattice).
PF=1⋅43π(1.4×10−8)3(2.8×10−8)3
Now we can simplify the expression:
PF=43π(1.4)3(2.8)3
Calculating the numerical values:
PF=43π(2.744)21.952
PF≈11.4821.952≈0.523
Thus, the packing fraction of the metal is approximately 0.523.
Similar Questions
Knowledge Check
The numbr of atoms in 0.1 mole of a triatomic gas is _______________.(NA=6.02×1023mol−1)
A1.800×1022B6.026×1022C1.806×1023D3.600×1023The numbr of atoms in 0.1 mole of a triatomic gas is _______________.(NA=6.02×1023mol−1)
A1.800×1022B6.026×1022C1.806×1023D3.600×1023An ionic solid PQ crystallises in rock salt structure with density 4.0gm/cm3.If the radius of cation and anion is 83 and 167 pm respectively, then the molar mass of solid is [NA=6×1023]
A75 gm/cm3B50gm/cm3C25gm/cm3D150gm/cm3
A box contains 12×1022 number of oxygen atoms. Find moles of O-atoms? (NA=6×1023)
View SolutionAn element with density 11.2gcm−3 forms a f. c. c. lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. (Given : NA=6.022×1023mol−1
View SolutionSilver crystallises in an fcc lattice. The edge length of its unit cell is 4.077×10−8 cm and its density is 10.5gcm−3. Calculate on this basis the atomic mass of silver. [NA=6.02×1023mol−1]
View SolutionThe edge length of unit cell of a metal having molecular weight 75gmol−1 is 5Å which crystallizes in cubic lattice. If the density is 2g^(−1), then find the radius of metal atom (NA=6×1023). Give the answer in pm.
View SolutionAn ionic solid PQ crystallises in rock salt structure with density 4.0gm/cm3 . If the radius of cation and anion is 83 and 167 pm respectively, then the molar mass of solid is
[NA=6×1023]
View Solution