To get a resultant displacement of 10 m, two displacement vectors, one of magnitude 6 m another of 8 m should be combined :
parallel
anti-parallel
at an angle of
perpendicular to each other
The correct Answer is:d
To solve the problem of combining two displacement vectors of magnitudes 6 m and 8 m to achieve a resultant displacement of 10 m, we can follow these steps:
1. Identify the Given Values:
- Let vector A = 6 m
- Let vector B = 8 m
- Resultant vector R = 10 m
2. Use the Formula for Resultant of Two Vectors:
The formula to find the resultant of two vectors A and B at an angle θ is given by:
R=√A2+B2+2ABcos(θ)
3. Substitute the Known Values into the Formula:
Substitute A, B, and R into the formula:
10=√62+82+2⋅6⋅8⋅cos(θ)
This simplifies to:
10=√36+64+96cos(θ)
4. Square Both Sides to Eliminate the Square Root:
102=36+64+96cos(θ)
100=100+96cos(θ)
5. Rearrange the Equation:
Subtract 100 from both sides:
0=96cos(θ)
6. Solve for cos(θ):
cos(θ)=0
7. Determine the Angle θ:
The cosine of an angle is zero at:
θ=90∘
8. Conclusion:
The two displacement vectors of 6 m and 8 m must be combined at an angle of 90 degrees to achieve a resultant displacement of 10 m.
- Let vector A = 6 m
- Let vector B = 8 m
- Resultant vector R = 10 m
The formula to find the resultant of two vectors A and B at an angle θ is given by:
R=√A2+B2+2ABcos(θ)
Substitute A, B, and R into the formula:
10=√62+82+2⋅6⋅8⋅cos(θ)
This simplifies to:
10=√36+64+96cos(θ)
102=36+64+96cos(θ)
100=100+96cos(θ)
Subtract 100 from both sides:
0=96cos(θ)
cos(θ)=0
The cosine of an angle is zero at:
θ=90∘
The two displacement vectors of 6 m and 8 m must be combined at an angle of 90 degrees to achieve a resultant displacement of 10 m.
Similar Questions
Can we add a displacement vector of 20 m to a displacement vector of 30 m. Expalin .
View SolutionThere are two displacement vectors,, one of magnitude 3 metres and the other of 4 metres. How would the two vectors be added so that the magnitude of the resultant vector be (a) 7 metres (b) 1 metre and ( c ) 5 metres.
View Solution
Knowledge Check
To get a rsultant displacement of 10m, two displacement vectors, one of magnetic 6 m and another of 8 m,should be combined :
AparallelBanti-parallelCat an angle 60∘Dperpendicular to eachThe car makes a displacement of 100m towards east and then 200m towards north. Find the magnitude and direction of the resultant.
A223.7m, tan−1(2),N of EB223.7m, tan−1(2),E of NC300m, tan−1(2), N of ED100m, tan−1(2), N of EThe magnitude of pairs of displacement vectors are give. Which pairs of displacement vectors cannot be added to give a resultant vector of magnitude 13 cm :-
(i)4cm,12cm (ii)4cm,8cm
(iii)6cm,8cm (iv)1cm,15cmA(ii, iv)B(i,ii)C(i, iii)D(ii, iii)
Represent graphically: A displacement of 20 m, north east.
View SolutionDisplacemtn vector →A points due east and has a magnitude of 2.00km. Displacement vector →Bp∮sduenorthandhasamagnitudeof3.75Km.Displacement→−−→rvecC points due west and has a magnitude of 2.50km. Displacement vector →D points due south and has a magnitude of 3.00km. Find the magnitude and direction (relative to due west) of the resultant vector →A+→B+→C+→D.
View SolutionThe magnetitude of pairs of displacement vectors are given. Which pairs of displacement vector cannot be added to give a resultant vector of magnitude 13 cm?
View SolutionWhat will be the period of the displacement body of mass m?
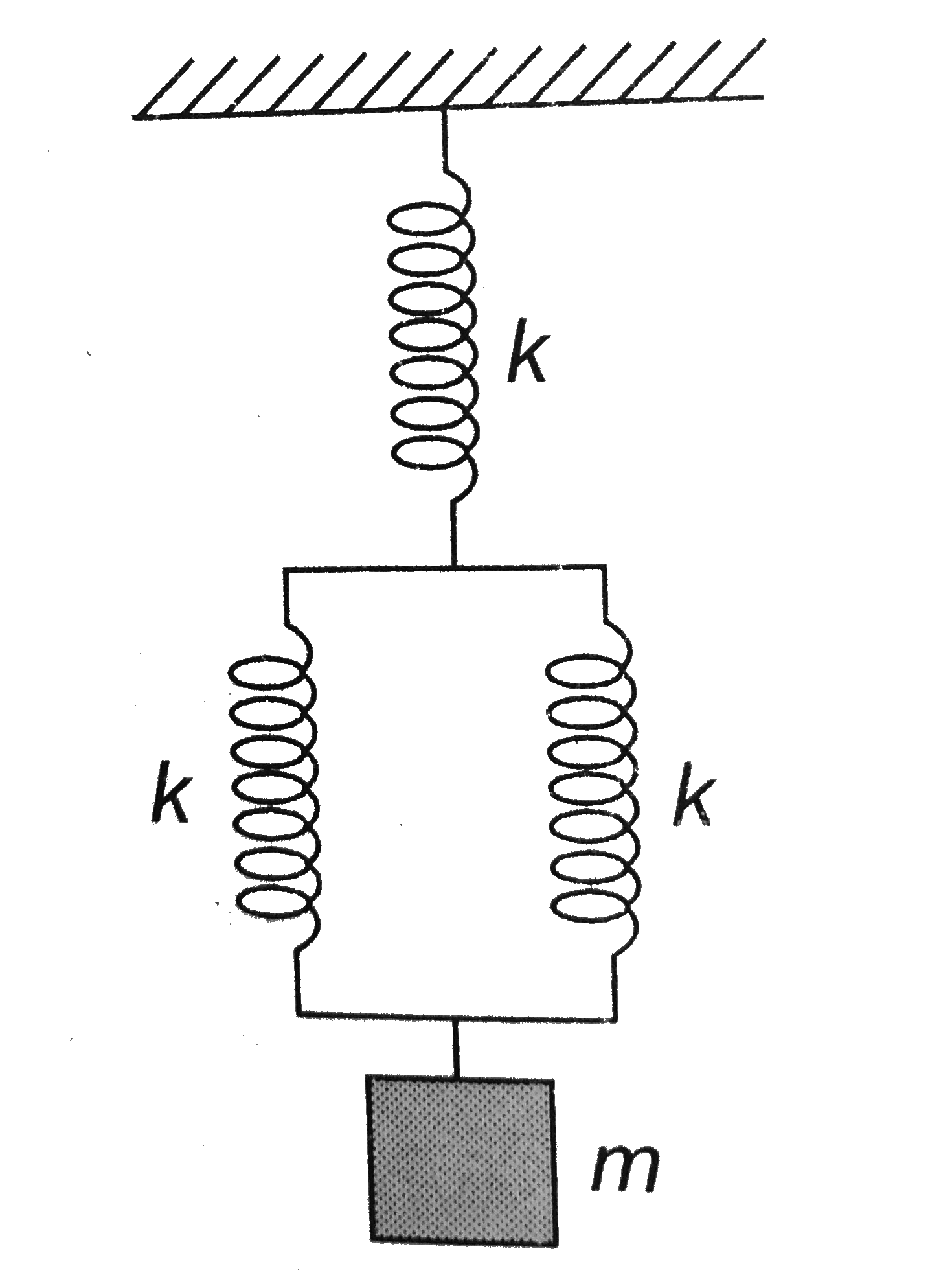
View SolutionMagnitudes of four pairs of displacement vectors are given. Which pair of displacment vectors, under vector addition fails to gives a resultant vectore of magnitude 3 cm ?
View Solution