The simplified value of (secA−cosA)2+(cosecA−sinA)2−(cotA−tanA)2.
0
12
1
2
The correct Answer is:c
(secA−cosA)2+(cosecA−sinA)2−(cotA−tanA)2
=(sec2A+cos2A−2secAcosA)
+(cosec2A+sin2A=2cosecAsinA)
−(cot2A+tan2A−2cotAtanA)
=sec2A−tan2A+cos2+sin2A
+cosec2A−cot2A−2
=3−2=1
Alternate shortcut method
(secA−cosA)2+(cosecA−sinA)2−(cotA−tanA)2
put θ=45∘
=(sec45∘−cos45∘)2+(cosec45∘−sin45∘)2−(cot45∘−tan45∘)2
=(√2−1√2)2+(√2−1√2)2−(1−1)2
=12+12−0=1
Topper's Solved these Questions
Similar Questions
एक रेलगाड़ी 45 किमी. /घंटा की चाल से चल रही है उसे 4 किमी. की दूरी तय करने में लगा समय ज्ञात कीजिए ?
03:19View SolutionPorve that : (cosecA-sinA)(secA-cosA)=1tanA+cotA
02:34View SolutionProve that : (sinA−cosA)(cotA+tanA)=secA−cosecA
03:03View SolutionProve that: sinA(1+tanA)+cosA(1+cotA)=secA+cosecA
02:35View Solution(cosecA−sinA)(secA−cosA)(tanA+cotA) = __________.
04:01View Solution(cosecA−sinA)(secA−cosA)=1tanA+cotA.
02:20View Solutionसिद्ध कीजिए कि
(sinA+cosA)(tanA+cotA)=secA+cosecA.03:22View SolutionProve: (cosec A−sinA)(secA−cosA)(tanA+cotA)=1
05:00View SolutionProve: sinAsecA+tanA−1+cosAcosec A+cotA−1=1
04:49View Solutionआकृति से sinA,cosA,tanA,cosecA,secA तथा cotA के मान ज्ञात कीजिए।
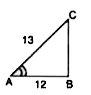 04:24View Solution
04:24View SolutionShow that (sinA)/(SecA+tanA-1)+(cosA)/(cosecA+cotA-1)=1
03:18View SolutionProve that :
1+cotAcosA+1+tanAsinA=2(secA+cosecA)03:03View SolutionPorve that : (cosecA-sinA)(secA-cosA)=1tanA+cotA
02:07View SolutionProve that : (sinA−cosA)(cotA+tanA)=secA−cosecA
02:15View SolutionProve that: sinA(1+tanA)+cosA(1+cotA)=secA+cosecA
02:06View Solution

