For L-R circuit, the time constant is equal to
twice the ratio of the energy stored in the magnetic field to the ratio of dissipation of energy in the resistance
the ratio of the energy stored in the magnetic field to the ratio of dissipation of energy in the resistance
half of the ratio of the energy stored in the magnetic field to the ratio of dissipation of energy in the resistance
square of the ratio of the energy stored in the magnetic field to the ratio of dissipation of energy in the resistance
The correct Answer is:A
Step by step video, text & image solution for For L-R circuit, the time constant is equal to by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
The time constunt of an L−R circuit is 10s. When a resistance of 10ohm is connected in series in a previous circuit, then the time constant becomes. 2s. The self-inductance (in henry) of the circuit is
View SolutionA current i0 is flowing through an L−R circuit of time constant i0. The source of the current is switched off at time t=0. Let r be the value of (−di/dt) at time t=0.Assuming this rate to be constant, the current will reduce to zero in a time interval of
View Solution
Knowledge Check
- Question 1 - Select One
During current growth in an L-R circuit the time constant is the time in which the magnitude of current becomes
Al0Bl0/2C0.63l0D0.37l0 - Question 2 - Select One
The figure shows an L−R circuit the time constant for the circuit is
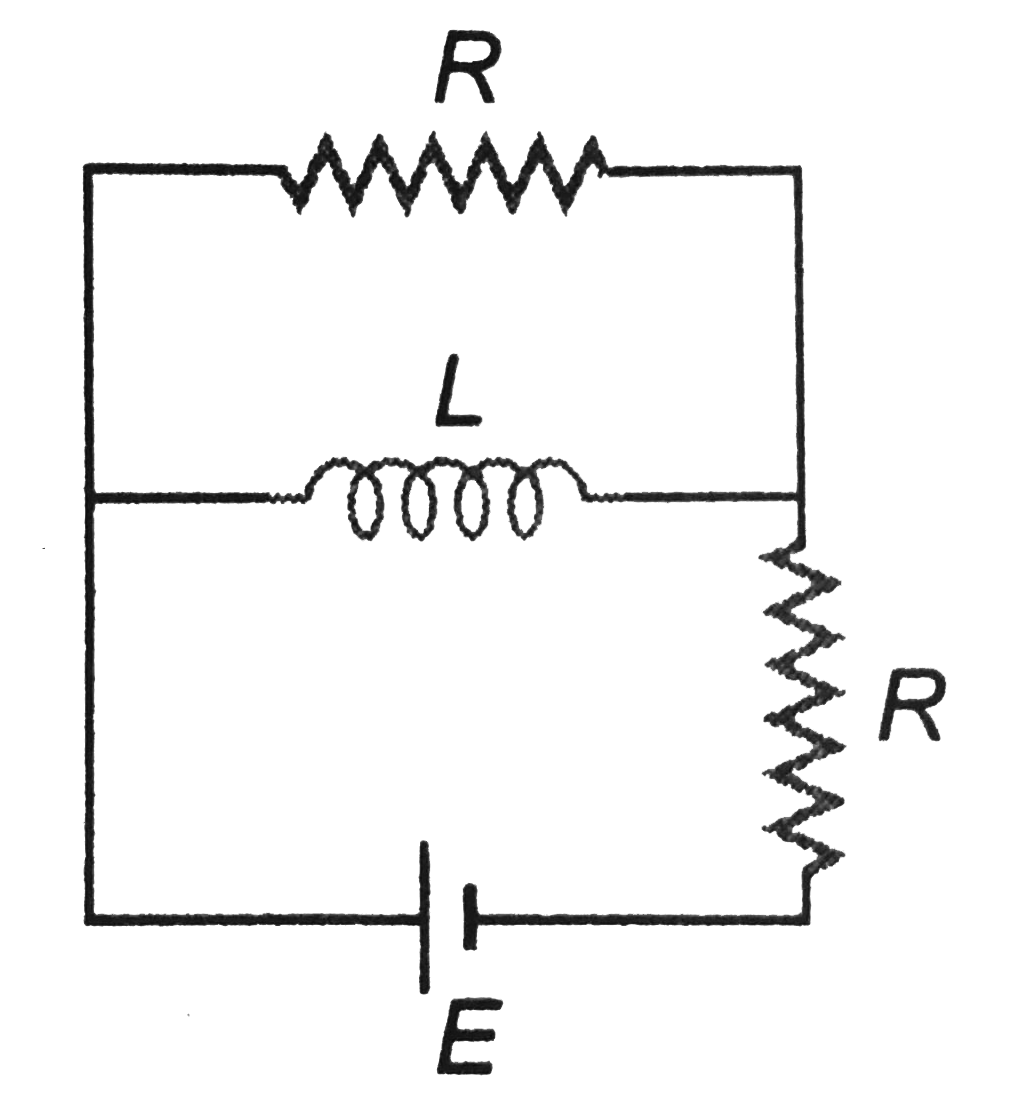 AL2RB2LRC2RLDR2L
AL2RB2LRC2RLDR2L - Question 3 - Select One
The growth of current in an L-R circuit in time t=L/r is equal to about __
A37% of maximumB63% of maximumC57% of maximumD67% of maximum
In a parallel L-C-R circuit spring constant K is analogous to :-
View SolutionIn the transient circuit shown the time constant of the circuit is -
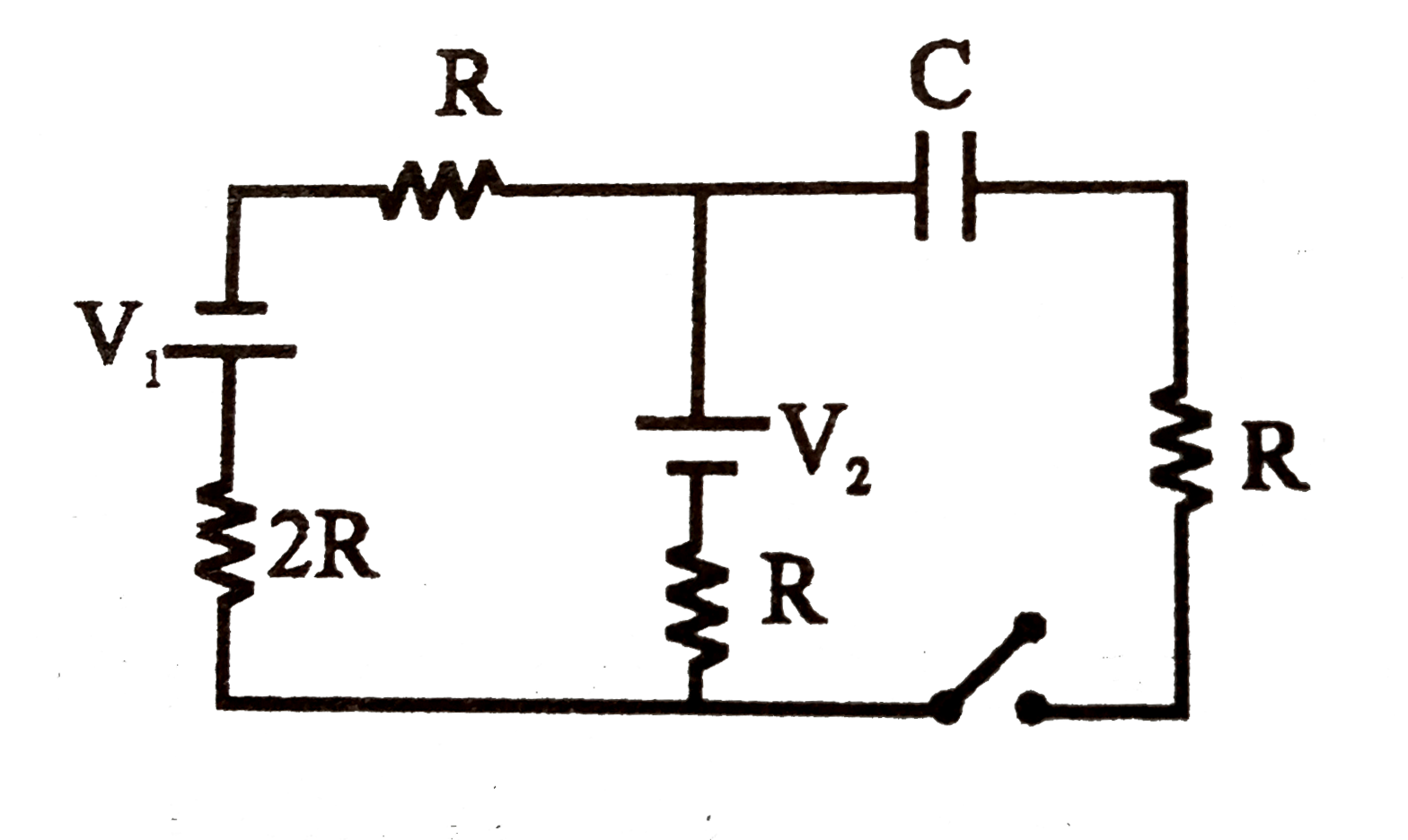 View Solution
View SolutionThe time constant of C-R circuit is
View SolutionGiven L1=1mH, R1=1Ω, L2=2mH, R2=2Ω
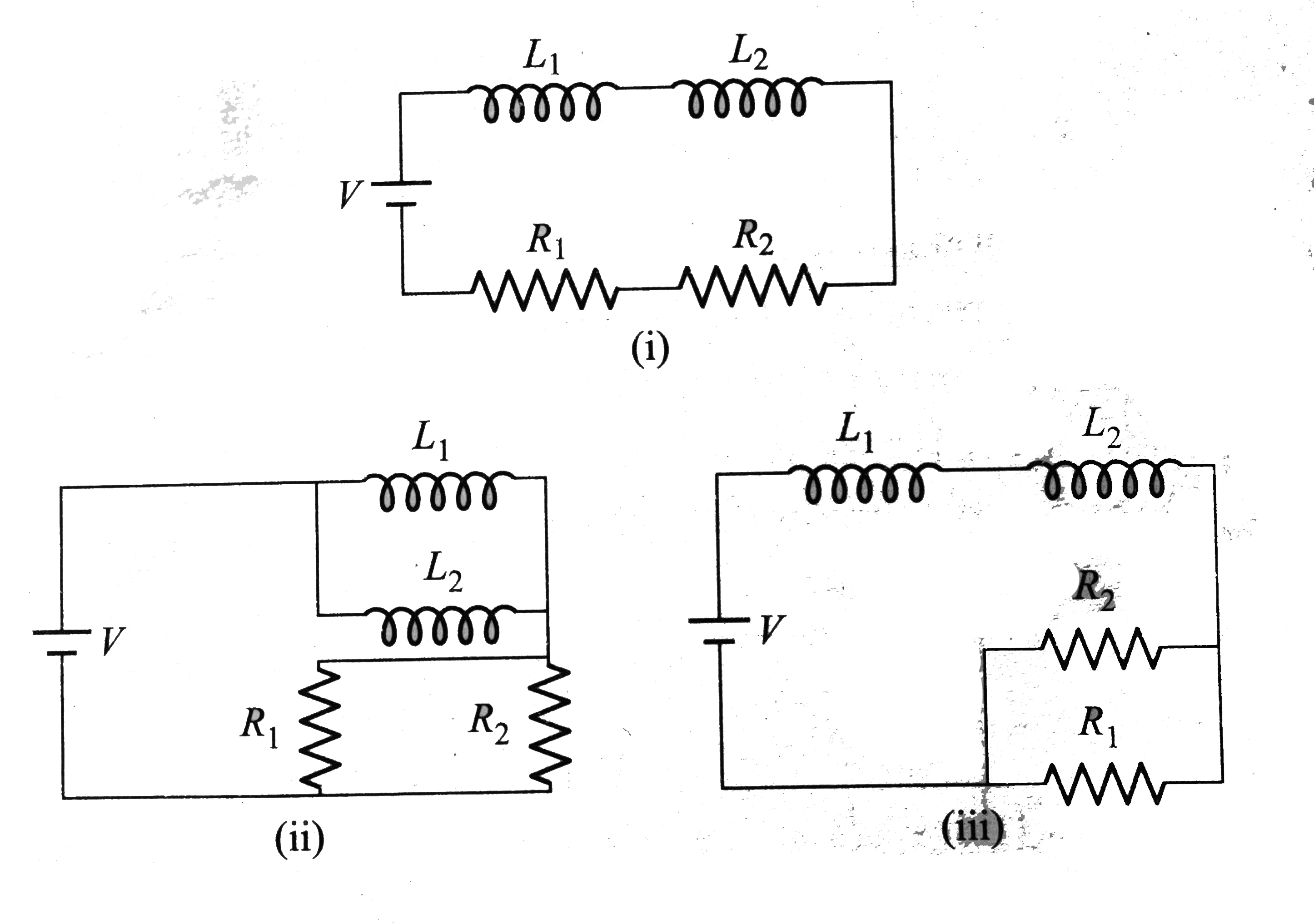


Neglecting mutual inductanec, the time constants (in ms) for circuits (i), (ii), and (iii) areView SolutionIf for above circuit the capacitive reactance is two times of inductive Reactance, and resistance R is equal to inductance Reactance then power factor of circuit is .
 View Solution
View Solution

