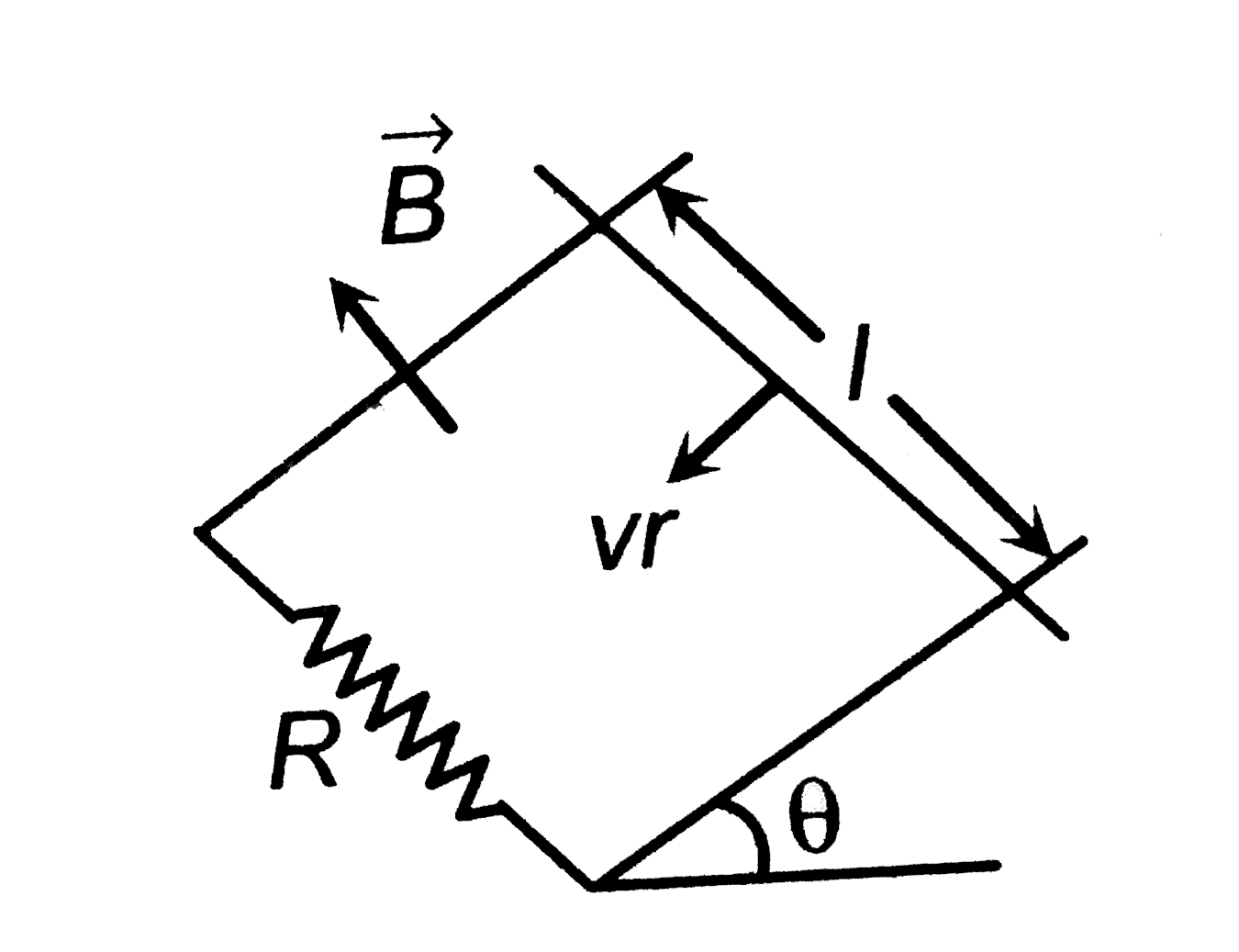
A conducting rod with resistance r per unit length is moving inside a vertical magnetic field B with spee v on two smooth horizontal parallel ideal conducting rails. The end of the rails are connected to a resistor R. the separation between the rails is d. The rod maintains a tilted angle θ to the rail. Find the external force F required to keep the rod moving
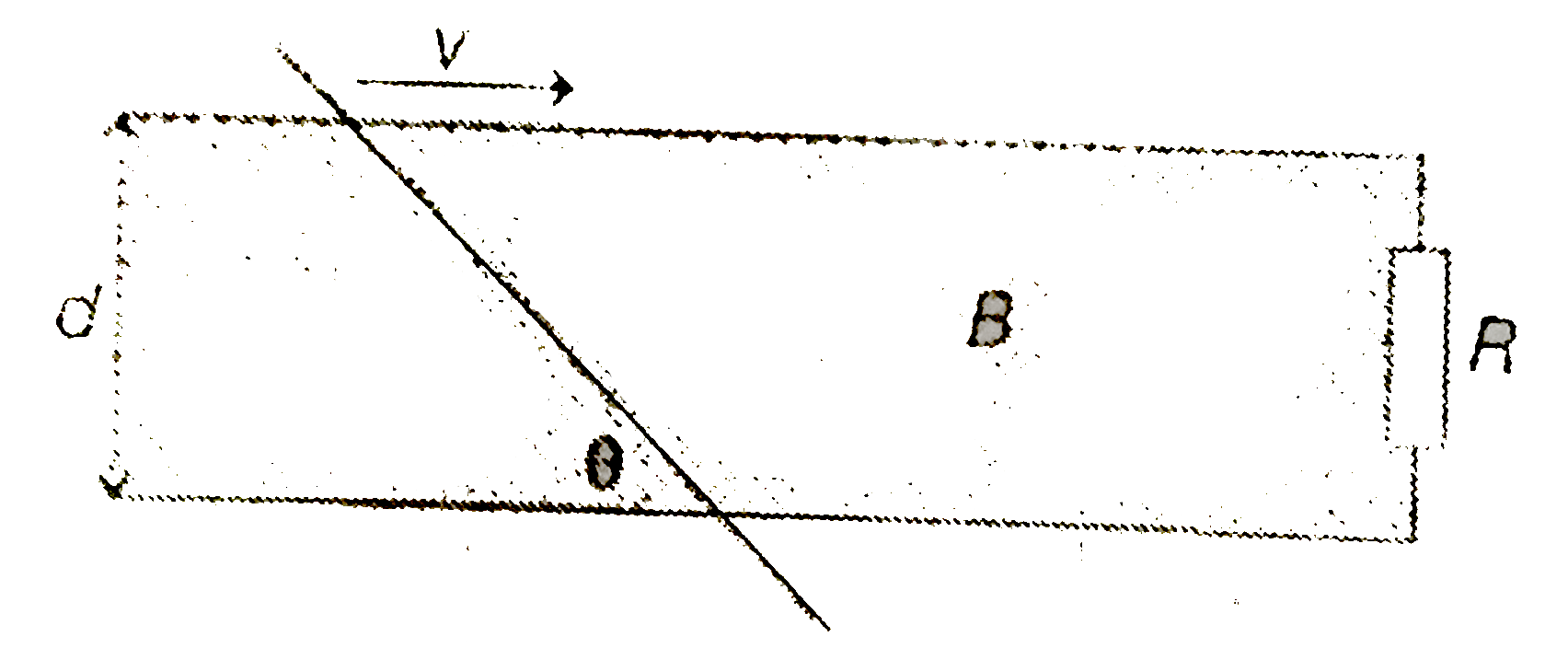
F=B2d2vR+dr
F=B2d2v(R+dr)/sinθ
F=B2d2vsinθ(R+dr)/sinθ
F=B2d2vcosθ(R+dr)/cosθ
The correct Answer is:C
Similar Questions
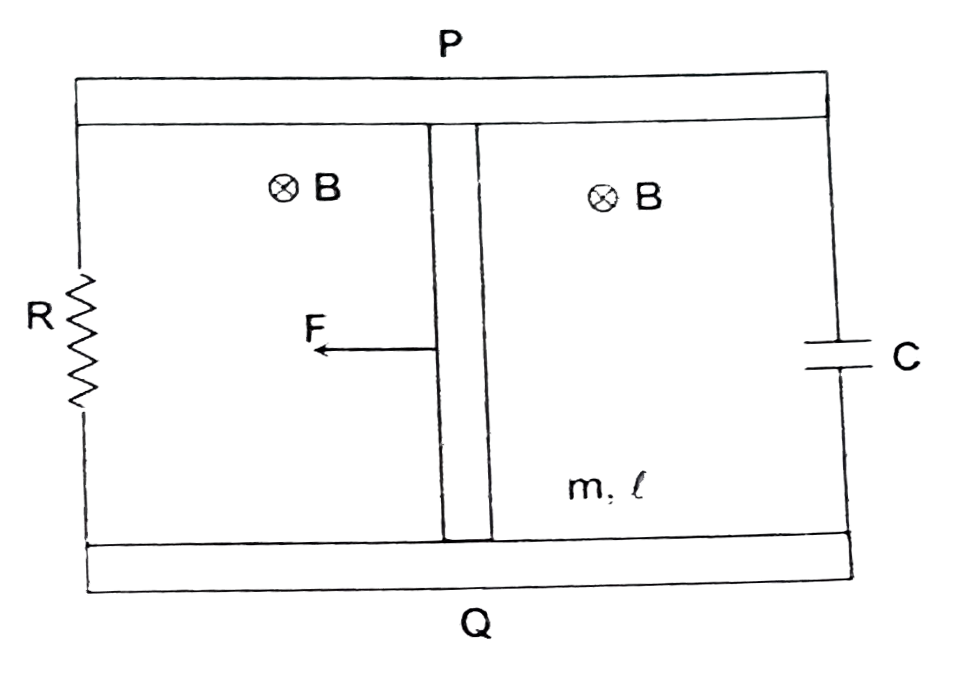
Two smooth horizontal parallel conducting rails are connected with a capacitor and a resistor at the two ends as shown. A uniform conducting rod PQ of mass 'm' and length 'l' is dragged with a constant horizontal force 'F'. The terminal velocity aquired by the conducting rod is
 View Solution
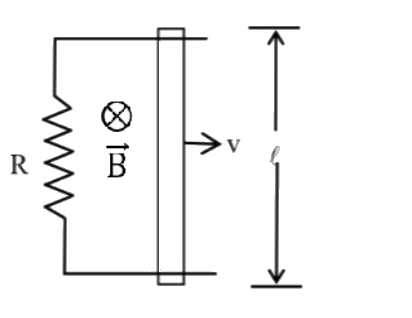
View SolutionA conducting rod of resistance r moves uniformly with a constant speed v in a uniform magnetic field. If the rod keeps moving uniformly, then the amount of force required is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two smooth horizontal parallel conducting rails are connected with a capacitor and a resistor at the two ends as shown. A uniform conducting rod PQ of mass 'm' and length 'l' is dragged with a constant horizontal force 'F'. The terminal velocity aquired by the conducting rod is
 A4FRB2l2B2FRB2l2CFRB2l2DFR2B2l2
A4FRB2l2B2FRB2l2CFRB2l2DFR2B2l2 - Question 2 - Select One
A pair of long, smooth, parallel, horizontal, conducting rails are joined to a cell at one end. There are no external electric or magnetic fields. A metal rod is placed on the rails. The rod will
Aremain stationaryBmove towards the cellCmove away from the cellDoscillate - Question 3 - Select One or More
A conducting rod of length is moved at constant velocity v0 on two parallel, conducting, smooth, fixed rails, that are placed in a uniform constant magnetic field B perpendicular to the plane for the rails as shown in Fig. A resistance R is connected between the two ends of the rails.
Then which of the following is/are correct:
 ATh thermal power dissipated in the resistor is equal to rate of work done by externl person pulling the rod.BIf applied external force is doubled then a part of external power increases the velocity of rod.CLenz's Law is not satisfied if the rod is accelerated by external forceDIf resistance R is doubled then power required to maintain the constant velocity v0 becomes half.
ATh thermal power dissipated in the resistor is equal to rate of work done by externl person pulling the rod.BIf applied external force is doubled then a part of external power increases the velocity of rod.CLenz's Law is not satisfied if the rod is accelerated by external forceDIf resistance R is doubled then power required to maintain the constant velocity v0 becomes half.
A conducting rod of resistance r moves uniformly with a constant speed v in a uniform magnetic field. If the rod keeps moving uniformly, then the amount of force required is
 View Solution
View SolutionA wire of mass m and length I can freely slide on a pair of parallel, smooth, horizontal rails placed in a vertical magnetic field B . The rails are connected by a capacitor of capacitance C. The electric resistance of the rails and the wire is zero. If a constant force F acts on the wire as shown in the figure, find the acceleration of the wire.
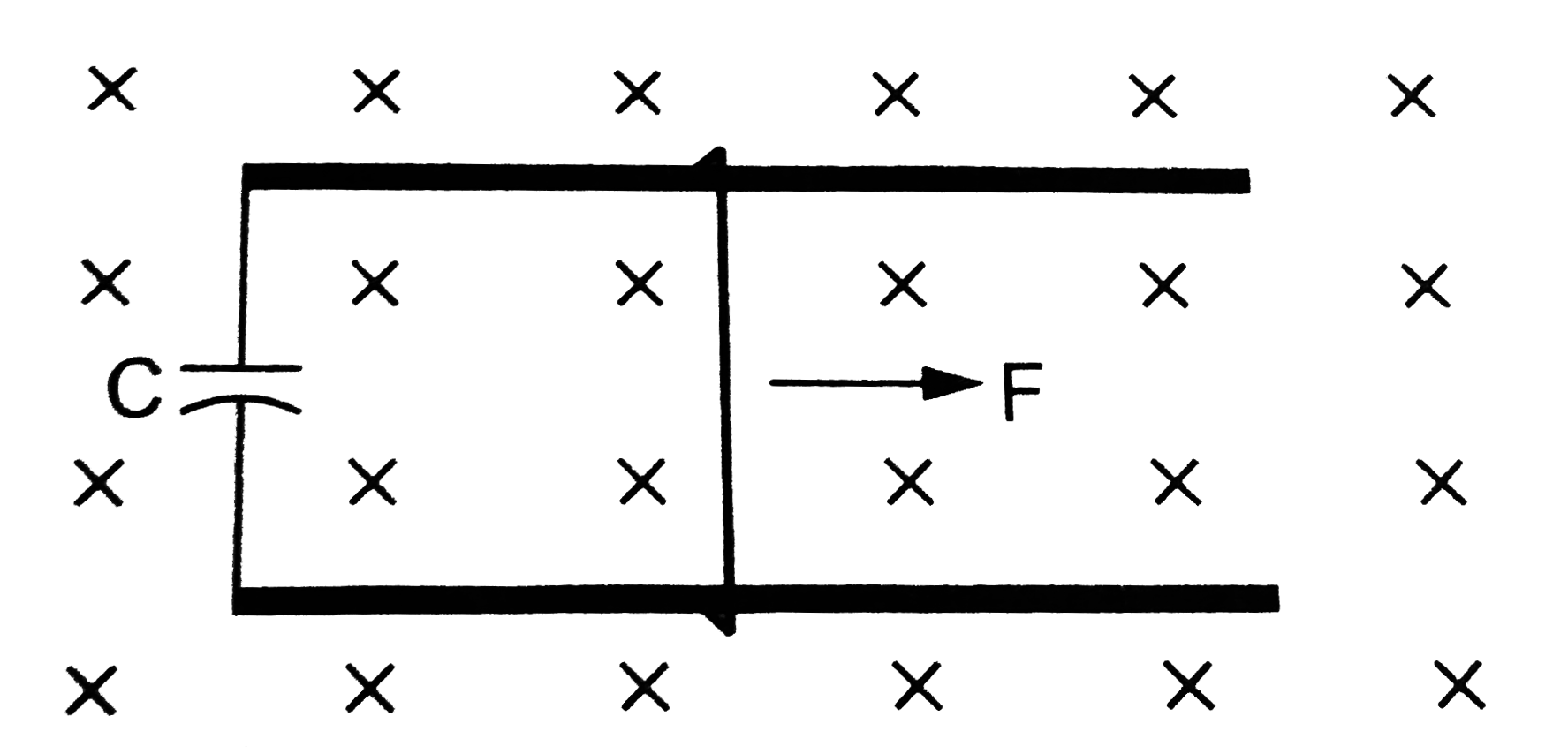 View Solution
View SolutionA conducting rod of length is moved at constant velocity v0 on two parallel, conducting, smooth, fixed rails, that are placed in a uniform constant magnetic field B perpendicular to the plane for the rails as shown in Fig. A resistance R is connected between the two ends of the rails.
Then which of the following is/are correct:
 View Solution
View SolutionA conducting rod of length is moved at constant velocity v0 on two parallel, conducting, smooth, fixed rails, that are placed in a uniform constant magnetic field B perpendicular to the plane for the rails as shown in Fig. A resistance R is connected between the two ends of the rails.
Then which of the following is/are correct:
 View Solution
View SolutionA copper rod of mass m slides under gravity on two smooth parallel rails l distance apart set at an angle θ to the horizontal. At the bottom, the rails are joined by a resistance R.
There is a uniform magnetic field perpendicular to the plane of the rails. the terminal valocity of the rod isView Solution

