On the basis of consumption function: C = 120 + 0.40 Y, answer the following questions:
(i) Derive the saving function.
(ii) Determine the saving at the income level of ₹ 500 crores.
(iii) At what level of income, saving becomes zero ?
(i) We know, Consumption function is expressed as: C= ¯c + b(Y)
It means: 120 is the autonomous consumption (¯c) and 0.40 indicates MPC or b
Saving Function is given as: S = - ¯c + (1+b)Y
MPS or (1-b) = 1 - MPC = 1 -0.40 = 0.60
Putting the values of (1-b) or MPS and - ¯c, we get : S = -120+0.60(Y)
(ii) For saving at income (Y) of ₹ 500 crores, putting the values of (1-b), ¯c and Y in the saving function, we get : S= -120 + 0.60 × 500 = ₹ 180 crores
(iii) Saving will become zero at break- even poit, i.e. when Y=C.
Replacing C with Y in the consumption function to determine the break-even point.
Y = 120 + 0.40 Y
0.6 Y = 120
Y = 200
Saving will become zero at income level of ₹ 200 crores
Topper's Solved these Questions
- BOOK - SANDEEP GARGCHAPTER - AGGREGATE DEMAND AND RELATED CONCEPTS EXERCISE - TRUE AND FALSE15videos
AGGREGATE DEMAND AND RELATED CONCEPTS
- BOOK - SANDEEP GARGCHAPTER - AGGREGATE DEMAND AND RELATED CONCEPTS EXERCISE - GUIDELINES TO NCERT QUESTIONS2videos
AGGREGATE DEMAND AND RELATED CONCEPTS
- BOOK - SANDEEP GARGCHAPTER - FOREIGN EXCHANGE RATE EXERCISE - REVISION EXERCISE12videos
FOREIGN EXCHANGE RATE
- BOOK - SANDEEP GARGCHAPTER - BALANCE OF PAYMENTSEXERCISE - LONG ANSWER TYPE QUESTIONS6videos
BALANCE OF PAYMENTS
Similar Questions
If APC of an Economy is 0.6 , saving at the income level of Rs. 1,000 crore will be
View SolutionIf APC of an economy is 0.8, what should be saving at an income level of ₹ 2,000 crores ?
View SolutionThe consumption function is given as C = 40+0.6Y. Derive saving function.
View SolutionThe saving function for an economy is given as: S = -120 + 0.4(Y). Determine: (i) Consumption function, (ii) Consumption at income level of ₹ 600 crores, (iii) Break-even level of income.
View SolutionThe consumption function of an economy is given as: C = 40+0.7Y. Calculate the saving at the income level of ₹ 2,200 crores
View SolutionOn the basis of given diagram, answer the following questions:
(i) What is the value of autonomous consumption ?
What is the break-even level of income or at what level of income saving is zero ?
(iii) How much is APC corresponding to point E ?
(iv) If MPC = 0.6, determine the consumption and saving function.
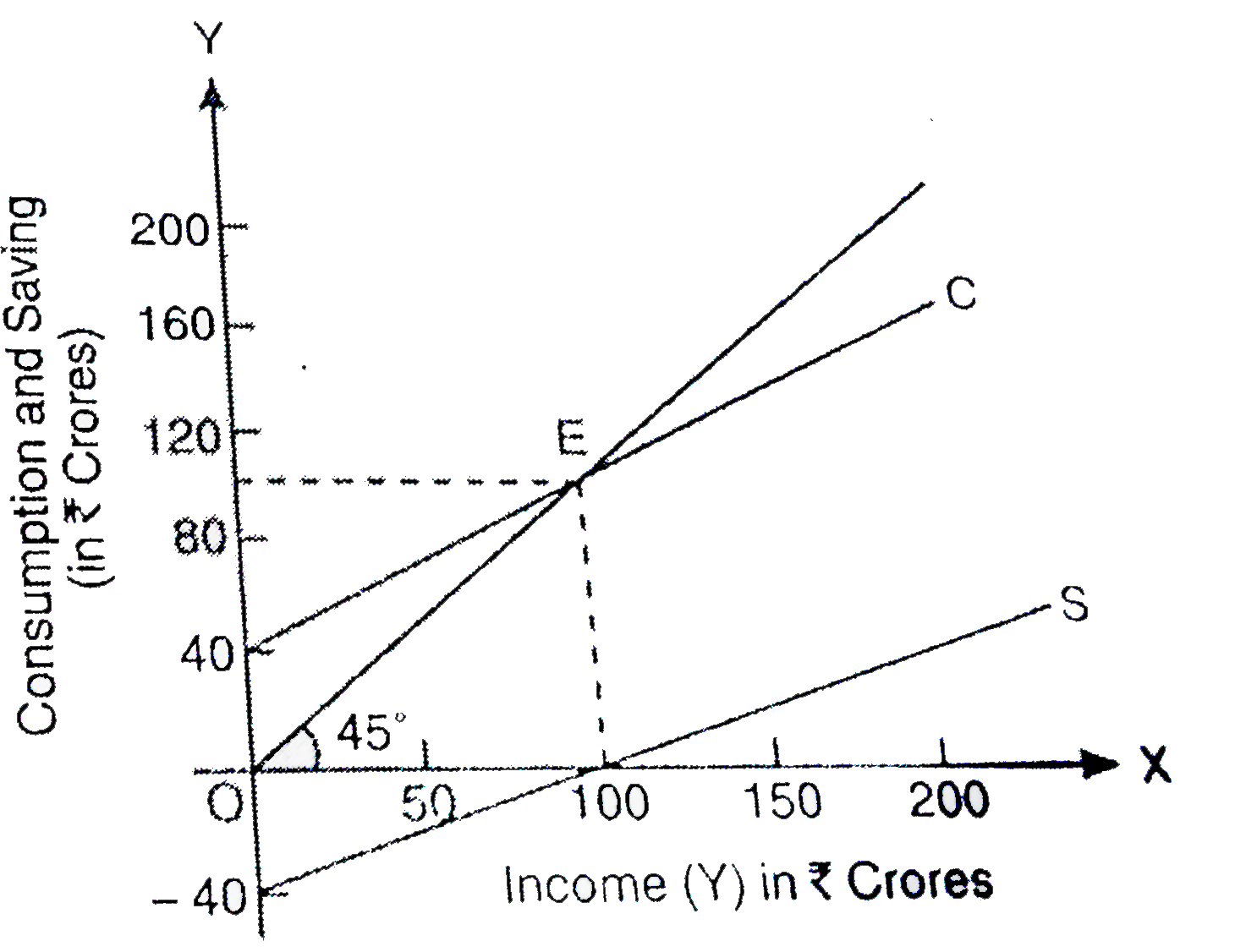 View Solution
View SolutionWith the help of saving function: S = -20+ 0.3 (Y), calculate consumption expenditure at the income level of ₹1,000 crores.
View SolutionFind saving level if income level is 500 crore and S=-50+0.60Y.
View SolutionWhat is the saving function ? Derive it from consumption function.
View SolutionAt income level of ₹ 5,000 crores, total saving is ₹ 1,000 crores. Calculate APC.
View Solution

