In the given figure, one side of the 30∘ inscribed angle is the diameter of a semicircle of radius 3 cm. What is the perimeter of the shaded region bounded by the inscribed angle and its intercepted arc
Step by step video & image solution for In the given figure, one side of the 30^@ inscribed angle is the diameter of a semicircle of radius 3 cm. What is the perimeter of the shaded region bounded by the inscribed angle and its intercepted arc by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
In the given figure, one side of the 30∘ inscribed angle is the diameter of a semicircle of radius 3 cm. What is the perimeter of the shaded region bounded by the inscribed angle and its intercepted arc
View SolutionIn the figure given below, the diameter of bigger semicircle is 108 cm. What is the area of the shaded region ?
View Solution
Knowledge Check
If the perimeter of a semicircle is 18 cm, what will be its diameter ?
A9 cmB28 cmC14 cmD7 cmIn the given figure, what is the radius of the inscribed semicircle having base on AB ?
 A3/2B5/2C7/5Dnone of these
A3/2B5/2C7/5Dnone of these
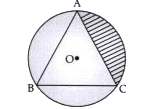
In the given figure, ABC is an equilateral triangle inscribed in a circle of radius 4 cm with centre O, then the area of the shaded region is :
View SolutionThe given figure shows a circle inscribed in a square of side 14 cm.

What is the area of the shaded region?
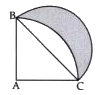
View SolutionIn the given figure, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region.
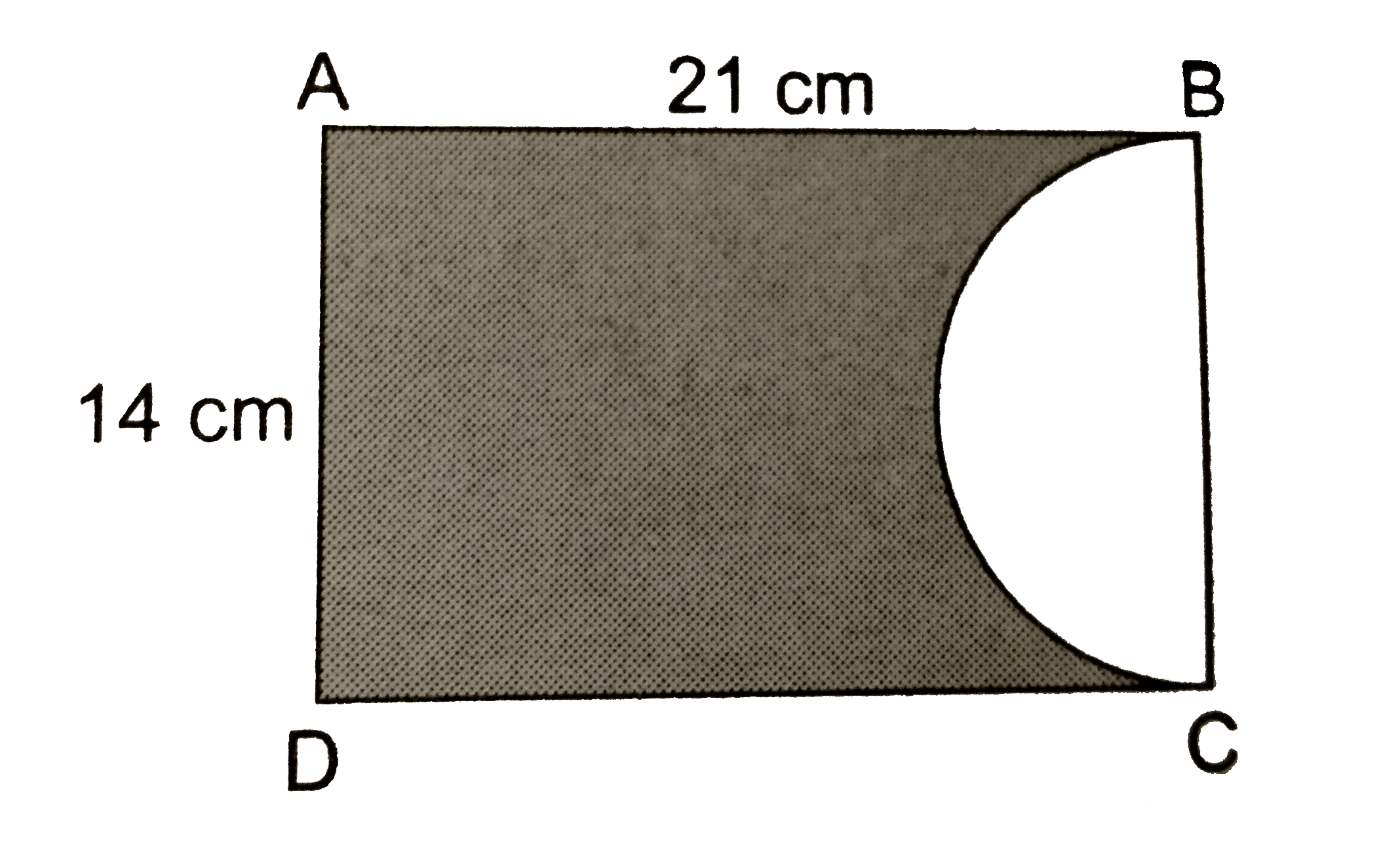
View SolutionIn the given figure ABCD is a rectangle of dimensions 21cm×14cm. A semicircle is drawn with BC as diameter. Find the area and the perimeter of the shaded region in the figure.
View SolutionIn the adjoining figure, PQR is an equilateral triangle inscribed in a circle of radius 7cm. Find the area of the shaded region.
View SolutionIn Figure, ABC is an equilateral triangle inscribed in a circle of radius 4 cm with centre O . Find the area of the shaded region.
View Solution
