A block of mass M placed on a frictionless horizontal table is pulled by another block of mass m hanging vertically by a massless string passing over a frictionless pulley. The tension in the string is :
mM+mg
MM+mg
M+mmng
MnM+ng
The correct Answer is:D
To find the tension in the string connecting the two blocks, we can follow these steps:
Step 1: Identify the forces acting on each block
- For the hanging block of mass m:
- The downward force due to gravity is mg (where g is the acceleration due to gravity).
- The upward force due to tension in the string is T.
- For the block of mass M on the table:
- The only horizontal force acting on it is the tension T.
Step 2: Write the equations of motion
- For the hanging block (mass m):
mg−T=ma(1)
Here, a is the acceleration of the system.
- For the block on the table (mass M):
T=Ma(2)
Step 3: Solve the equations simultaneously
- From equation (2), we can express a:
a=TM(3)
- Substitute equation (3) into equation (1):
mg−T=m(TM)
Step 4: Rearrange the equation
- Rearranging gives:
mg−T=mTM
mg=T+mTM
mg=T(1+mM)
Step 5: Solve for tension T
- Now, isolate T:
T=mg1+mM
T=mgM+mM=mgMM+m
Final Result
The tension in the string is:
T=mgMM+m
---
- For the hanging block of mass m:
- The downward force due to gravity is mg (where g is the acceleration due to gravity).
- The upward force due to tension in the string is T.
- The only horizontal force acting on it is the tension T.
- For the hanging block (mass m):
mg−T=ma(1)
Here, a is the acceleration of the system.
T=Ma(2)
- From equation (2), we can express a:
a=TM(3)
mg−T=m(TM)
- Rearranging gives:
mg−T=mTM
mg=T+mTM
mg=T(1+mM)
- Now, isolate T:
T=mg1+mM
T=mgM+mM=mgMM+m
The tension in the string is:
T=mgMM+m
Similar Questions
A block A of 10 kg rests on a rouch, horizontal table and is connected to another block of mass 5 kg by a string passing over frictionless pulley. This mass hangs vertically (a) Determinem the minimum weight of a third block C which can be placed on A to prevent it from sliding if μs=0.2 (b) The block C is suddenly lifted, then what is the acceleration of the block A ? (μk=0.18)
View SolutionA block of mass m1=2 kg is kept on a smooth horizontal table. Another block of mass m2=1 kg is connected to m1 by a light inextensible string passing over a smooth pulley as shown in the figure. If the blocks are released, what will be the (a) acceleration of each block (b) tension in the string?
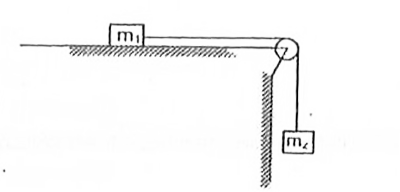
View Solution
Knowledge Check
Two bodies of massless string passing over a frictionless pulley. The acceleration of the system is (g=9.8m/s2)
A4.9m/s2B2.45m/s2C1.4m/s2D9.5m/s2Two masses ,each equal to m ,are arrached to one another by a massless string passing over a smooth pulley the tension in the string is :
AmgB2mgCmg/2DzeroTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A block of mass M is placed on a rough horizontal surface. It is connected by means of a light inextensible string passing over a smooth pulley . The other end of string is connected to a block of mass m. There is no friction between vertical surface and block m
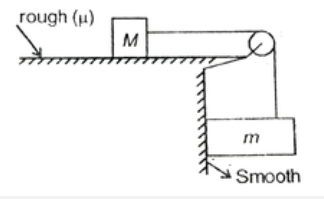
The minimum value of coefficient of friction μ such that system remains at rest , is
View SolutionA block of mass M is placed on a rough horizontal surface. It is connected by means of a light inextensible string passing over a smooth pulley . The other end of string passing over a smooth pulley. The other end of string is connected to a block of mass m. There is no friction between vertical surface and block m
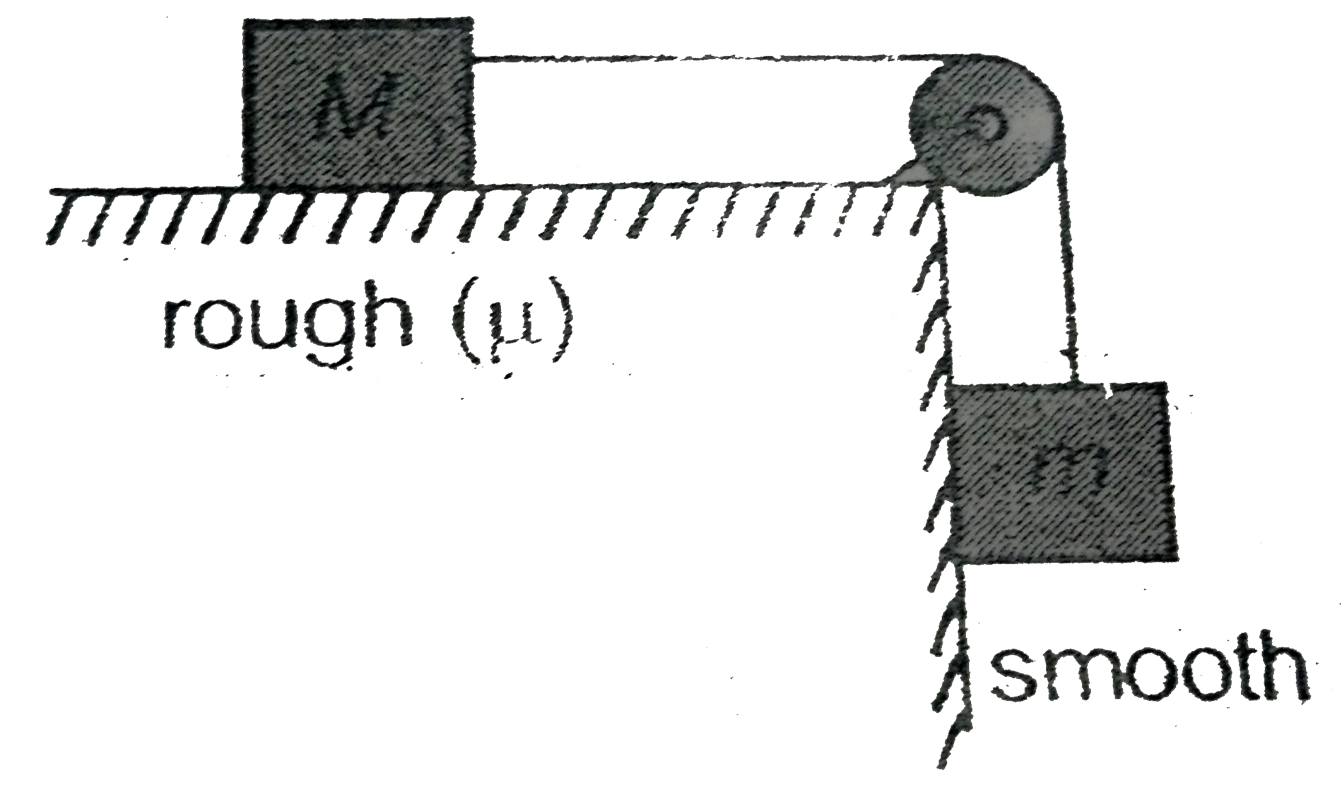
If the whole system ( horizontal surface + blocks ) accelerates towards right the value of maximum on the horizontal surface is
View SolutionTwo masses are hanging vertically over frictionless pulley. The acceleraton of the two masses is -
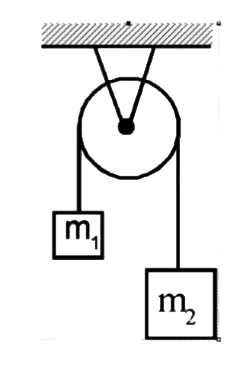
View SolutionTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.

View SolutionTwo blocks of masses 3 kg and 6 kg are connected by a string as shown in the figure over a frictionless pulley. The acceleration of the system is

View Solution