A point mass is subjected to two simultaneous sinusoidal displacement in x-direction, x1(t)=Asinωt and x2(t)=Asin(ωt+2π/3). Adding a third sinusoidal displacement x3(t)=Bsin(ωt+ϕ) brings the mass to a complete rest. The values of B and ϕ are :
√2A,3π4
A,4π3
√3A,5π6
A,π3
The correct Answer is:B
To solve the problem, we need to find the values of B and ϕ such that the resultant displacement of the point mass is zero when subjected to the three sinusoidal displacements.
1. Identify the Given Displacements:
We have two initial displacements:
x1(t)=Asin(ωt)
x2(t)=Asin(ωt+2π3)
2. Convert to Phasor Representation:
The first displacement can be represented as a phasor at an angle of 0∘ (or 0 radians):
- x1 has an amplitude A and angle 0.
The second displacement can be represented as a phasor at an angle of 2π3 (or 120∘):
- x2 has an amplitude A and angle 2π3.
3. Calculate the Resultant of x1 and x2:
To find the resultant displacement R of x1 and x2, we use the formula for the resultant of two vectors:
R=√A2+A2+2A⋅A⋅cos(2π3)
Since cos(2π3)=−12:
R=√A2+A2−A2=√A2=A
4. Determine the Angle of the Resultant:
The angle θ of the resultant can be calculated using the sine rule or by vector addition:
tan(θ)=Asin(0)+Asin(2π3)Acos(0)+Acos(2π3)
Since sin(2π3)=√32 and cos(2π3)=−12:
tan(θ)=0+A⋅√32A−A⋅12=√3212=√3
Thus, θ=π3 (or 60∘).
5. Determine the Third Displacement x3:
To bring the mass to rest, the third displacement x3 must be equal in magnitude and opposite in direction to the resultant of x1 and x2:
x3(t)=Bsin(ωt+ϕ)
The angle ϕ must be:
ϕ=θ+π=π3+π=4π3
The amplitude B must be equal to the magnitude of the resultant:
B=A
Final Values:
Thus, the values of B and ϕ are:
B=A,ϕ=4π3
We have two initial displacements:
x1(t)=Asin(ωt)
x2(t)=Asin(ωt+2π3)
The first displacement can be represented as a phasor at an angle of 0∘ (or 0 radians):
- x1 has an amplitude A and angle 0.
- x2 has an amplitude A and angle 2π3.
To find the resultant displacement R of x1 and x2, we use the formula for the resultant of two vectors:
R=√A2+A2+2A⋅A⋅cos(2π3)
Since cos(2π3)=−12:
R=√A2+A2−A2=√A2=A
The angle θ of the resultant can be calculated using the sine rule or by vector addition:
tan(θ)=Asin(0)+Asin(2π3)Acos(0)+Acos(2π3)
Since sin(2π3)=√32 and cos(2π3)=−12:
tan(θ)=0+A⋅√32A−A⋅12=√3212=√3
Thus, θ=π3 (or 60∘).
To bring the mass to rest, the third displacement x3 must be equal in magnitude and opposite in direction to the resultant of x1 and x2:
x3(t)=Bsin(ωt+ϕ)
The angle ϕ must be:
ϕ=θ+π=π3+π=4π3
The amplitude B must be equal to the magnitude of the resultant:
B=A
Thus, the values of B and ϕ are:
B=A,ϕ=4π3
Similar Questions
A point mass is subjected to two simultaneous sinusoidal displacement in x -direction, x1(t)=Asinωt and x2(t)=Asin(ωt+π2). Adding a third sinusoidal displacement x3t=Bsin(ωt+ϕ) brings the mass to a complete rest. The value of ϕ is nπ4. Then n is.
View SolutionA particle is subjected to two simple harmonic motions
x1=A1sinωt
andx2=A2sin(ωt+π3)
Find a the displacement at t=0, b. the maxmum speed of the particle and c. the maximum acceleration of the particle
View Solution
Knowledge Check
A point mass is subjected to two simultaneous sinusoidal displacement in x−direction, x1(t)=Asinωt and x2(t)=Asin(ω+2π3). Adding a third sinusoidal displacement x3(t)=Bsin(ωt+ϕ) brings the mass to complete rest. The value of B and ϕ are
A√2A,3π4BA,4π3C√3A,5π6DA,π3A point mass is subjected to two simultaneous sinusoidal displacements in x−direction,x1(t)=Asin(ω)tandx2(t)=Asin((ωt+2π3). Adding a third sinusoidal displacement x3(t)=Bsin(ωt+ϕ) brings the mas to a complete rest. The values of (B) and (phi) are.
A√2A,3π4BA,4π3C√3A,5π3DA,π3x and y displacements of a particle are given as x(t)=−asinωt and y(t)=asin2ωt. Its trajectory will look like.
A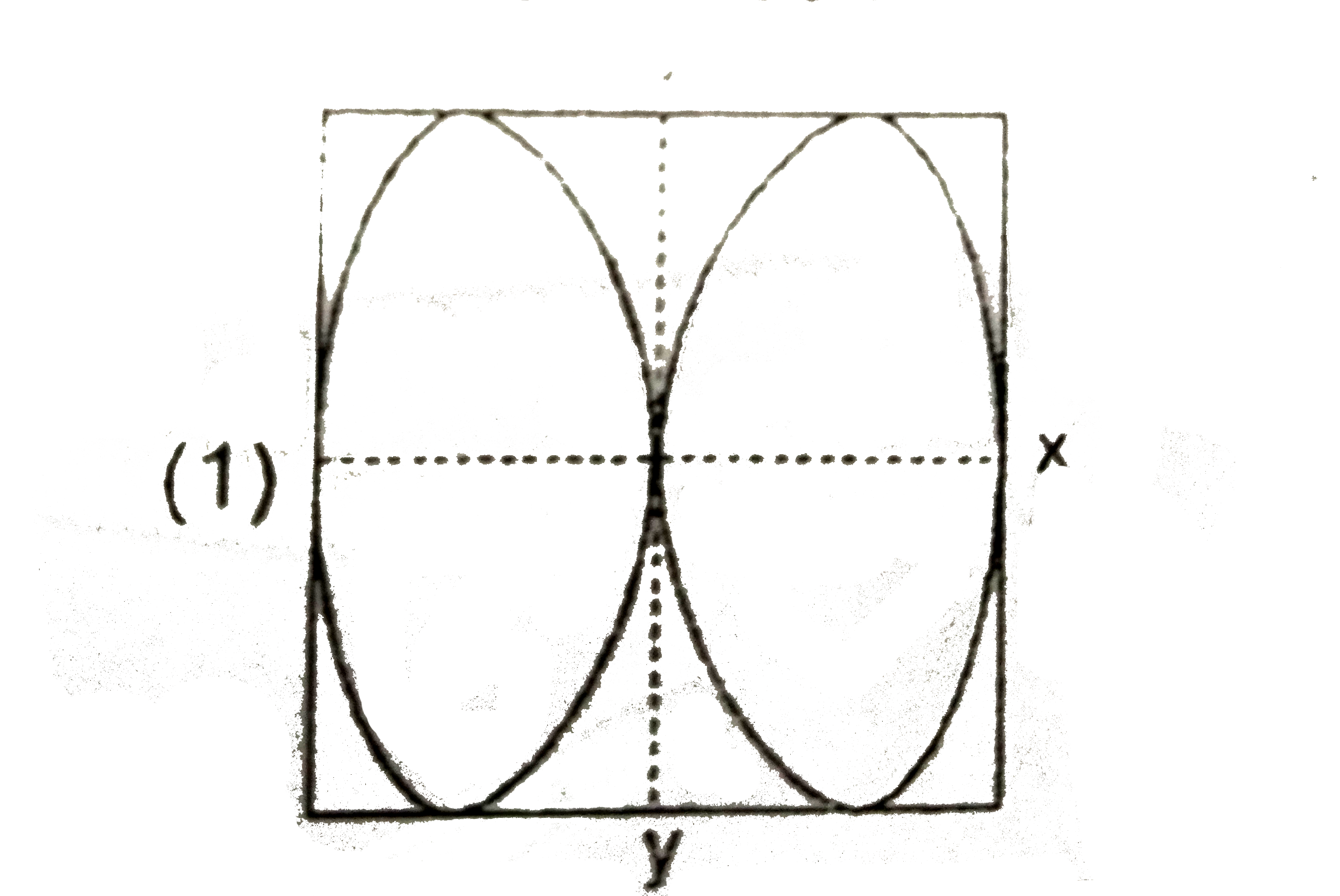 B
B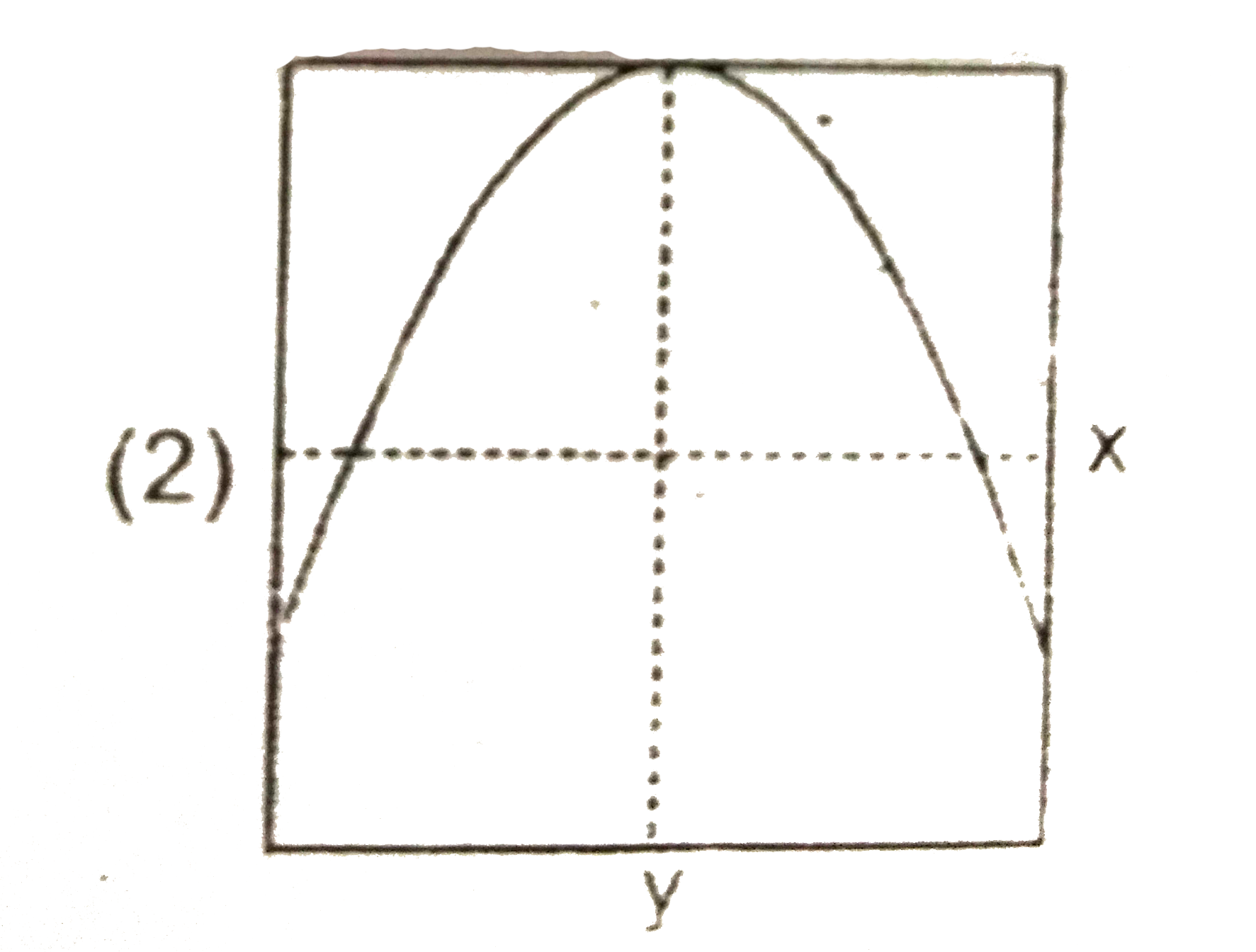 C
C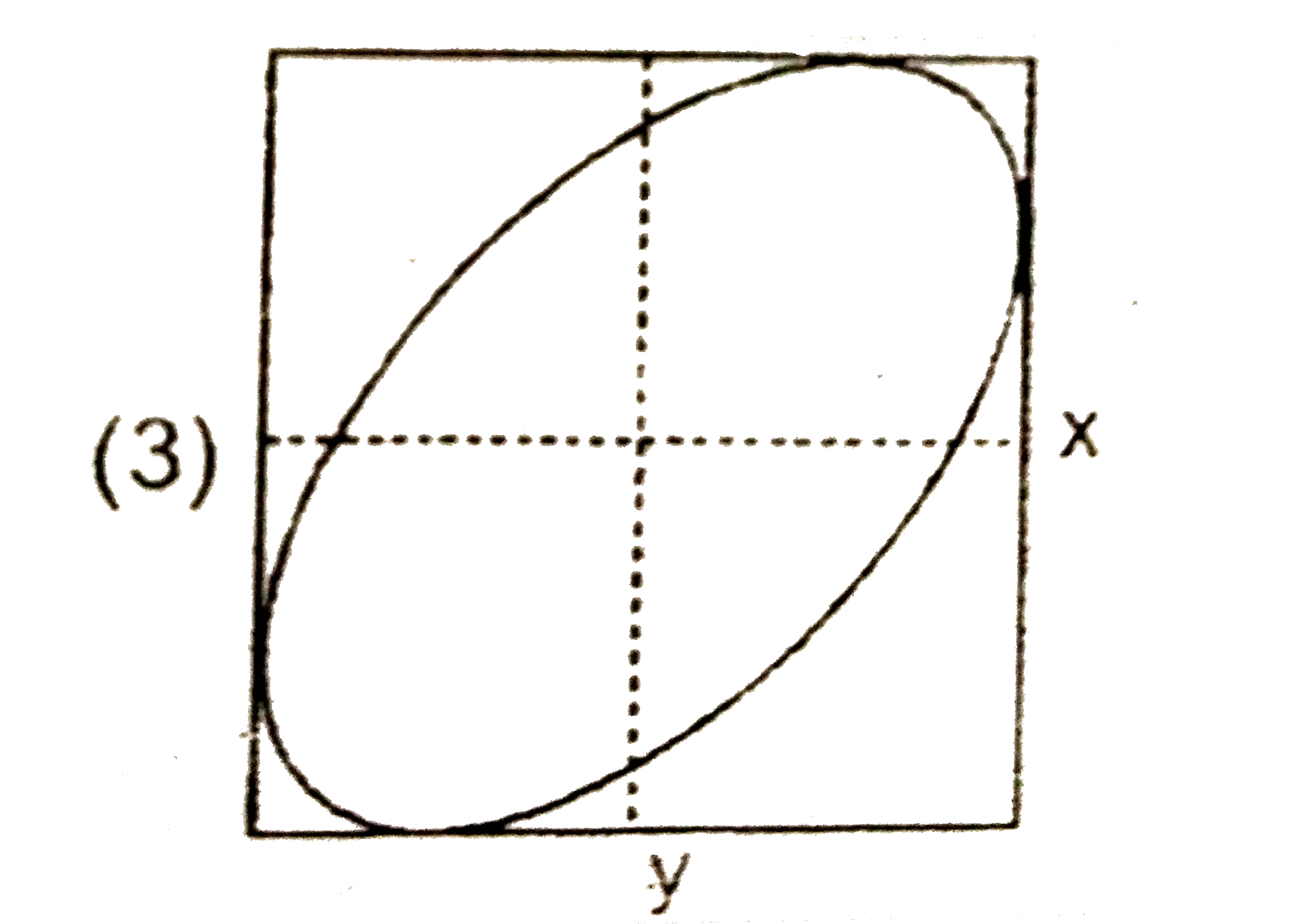 D
D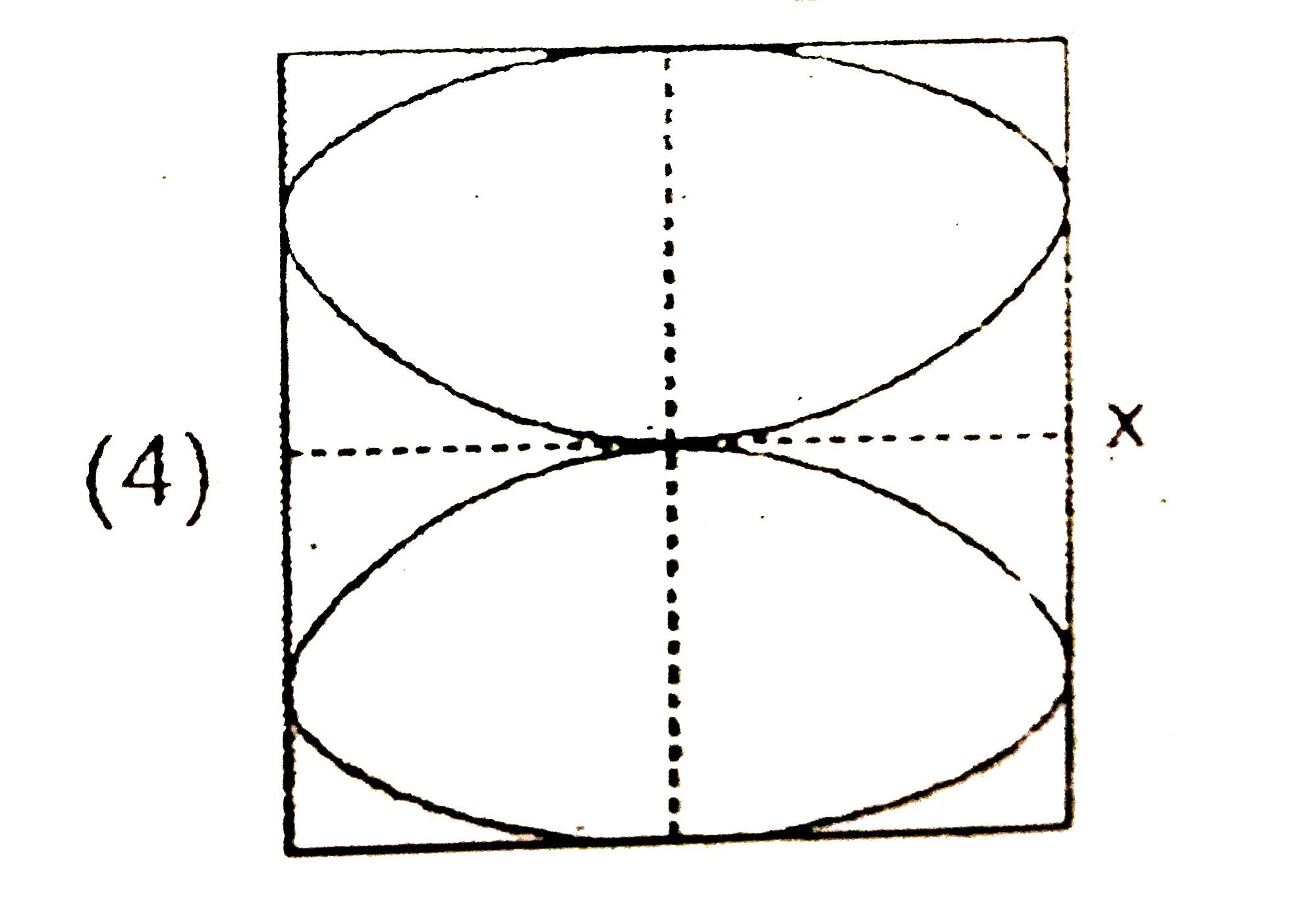
A particle is subjected to two simple harmonic motions.
x1=4.0sin(100πt) and x2=3.0sin(100πt+π3)
Find
(a) the displacement at t=0
(b) the maximum speed of the particle and
(c ) the maximum acceleration of the particle.
View Solutionx1=Asin(ωt−0.1x) and x2=Asin(ωt−0.1x−ϕ2) Resultant amplitude of combined wave is
View SolutionFour simple harmonic vibrations
x1=8sin(ωt),x2=6sin(ωt+π2),
x3=4sin(ωt+π) and x4=2sin(ωt+3π2) are superimposed on each other. The resulting amplitude is……units.
View SolutionWhat is the phase difference between two simple harmonic motions represented by x1=Asin(ωt+π6) and x2=Acos(ωt)?
View SolutionFour simple harmonic motions,c1=8sinωt,x2=6sin(ωt=π/2),x3=4sin(ωt+π)andx4=2sin(ωt+3π/2) are superimposed on each other. The resuslting amplitude and its phase difference with x1 are respectively
View Solution
