A ball of mass 2 kg is thrown from a tall building with velocity,
v=(20m/s)ˆi+(24m/s)ˆj at time t=0 s. Change in the potential energy of the ball after, t=8 s is (The ball is assumed to be in air buring its motion between 0 s and 8 s, ˆi is along the horizontal and ˆj is along the vertical direction. (Take g=10m/s2)Solution in Telugu
−2.56 kJ
0.52 kJ
1.76 kJ
−2.44 kJ
The correct Answer is:A
Given, velocity of the ball, v=(20ˆi+24ˆj) m/s and mass of the ball, m=2 kg
From the equation of motion, when a ball is throuwn,
v=u−gt ...(i)
Substituting the given values in Eq. (i), we get
v=(20ˆi+24ˆj)−(10ˆj)8
=20ˆi+24ˆj−80ˆj=20ˆi−56ˆj ...(ii)
From the law of conservation of energy, charge in potential energy of the ball = change in kinetic energy of the ball
⇒ΔPE=12mu2−12mv2
=12m(u2−v2)
=22(u.u−v.v)
=[(20ˆi+24ˆj).(20ˆj+24ˆj)]−[(20ˆi−56ˆj).(20ˆi−56ˆj)]
=(20)2+(24)2−(20)2−(56)2
=(24)2−(56)2=576−3136
=−2560=−2.56 kJ
Hence, change in potential energy of the ball after t=85 s is −2.56 kJ.
Topper's Solved these Questions
Similar Questions
A tokamak fusion test reactor works on
View SolutionWhich of the following represents fundamental forces of nature ?
View SolutionA projectile is given an initial velocity of ( hat(i) + 2 hat (j) ) m//s , where hat(i) is along the ground and hat (j) is along the vertical . If g = 10 m//s^(2) , the equation of its trajectory is :
View SolutionA sphere of mass 1 kg rests at one corner of a cube. The cube is moved with a velocity v =(8 t hat(i) - 2t^(2)) hat(j) , where t is time in second . The force by sphere on the cube at t = 1 s is (g = 10 m//s^(-2)) [figure shown vertical plane of the cube]
View SolutionA particle is moving with speed 6m/s along the direction of →A=2ˆi+2ˆj−ˆk, then its velocity is :
View SolutionAn observer O_(1) standing on ground finds that momentum of a projectile of mass 2 kg changes with time as vec(P)_(01) =(4that (i) + 20 t hat(k))kg m//s Acceleration due to gravity is vec(g)=(10 hat(k))m//s^(2) and there is a wind blowing in horizontal direction. Another observer O_(2) driving a car observes that momentum of the same projectile changes with time as - vec(P)_(02)=(8t hat(i)-16 hat(t) hat(j)+20 t hat(k))kg m//s . Find the acceleration of the car at t = (1)/(8) s
View SolutionThe velocity and acceleration vectors of a particle undergoing circular motion are v=2ˆim/sanda=2ˆi+4ˆjm/s2 respectively at some instant of time. The radius of the circle is
View SolutionA particle is moving in a circular path. The acceleration and momentum of the particle at a certain moment are a=(4 hat(i)+3hat(j))m//s^(2) and p=(8 hat(i)-6hat(j))"kg-m/s" . The motion of the particle is
View SolutionA particle of mass 2kg is projected with a velocity (6ˆi+8ˆj) m/s. Find the magnitude of change in momentum (in kg m/s) when it just touches ground again.
View SolutionA ball of mass 2 kg is thrown form a tall building with velocity →V =(20 m/s) ˆi + (24 m/s) ˆj at time t=0 s . Change in the potential energy of the ball after t=8 s is (The ball is assumed to be in air during its motion between 0 s and 8 s, ˆi is along the horizontal and ˆj is along the vertical direction , let g=10m/s2 )
View SolutionA particle of mass 0.020 kg moves along a curve with velocity 5.0ˆi+18ˆkm/s. After some time, the velocity changes to 9.0ˆi+22ˆj m/s due to action of a single force. Find the work done on the particle during this interval of time.
View SolutionAt t=0 a 1.0 kg ball is thrown from a tall tower with vec(v)=(18 m//s) hat(i)+(24 m//s) hat(j) . What is DeltaU of the ball-Earth system between t=0 and t=6.0s ( still free fall ) ?
View SolutionA 0.8 kg mass object whose initial velocity (3 hat i-4 hat j)m//s And final velocity (-6 hat j+2 hat k)m//s Find the change in energy.
View SolutionA projectile is given an initial velocity of (ˆi+2ˆj)m/s, where ˆi is along the ground and ˆj is along the vertical . If g=10m/s2, the equation of its trajectory is :
View SolutionA sphere of mass 1kg rests at one corner of a cube. The cube is moved with a velocity v=(8tˆi−2t2)ˆj, where tis time in second . The force by sphere on the cube at t=1s is (g=10m/s−2) [figure shown vertical plane of the cube]
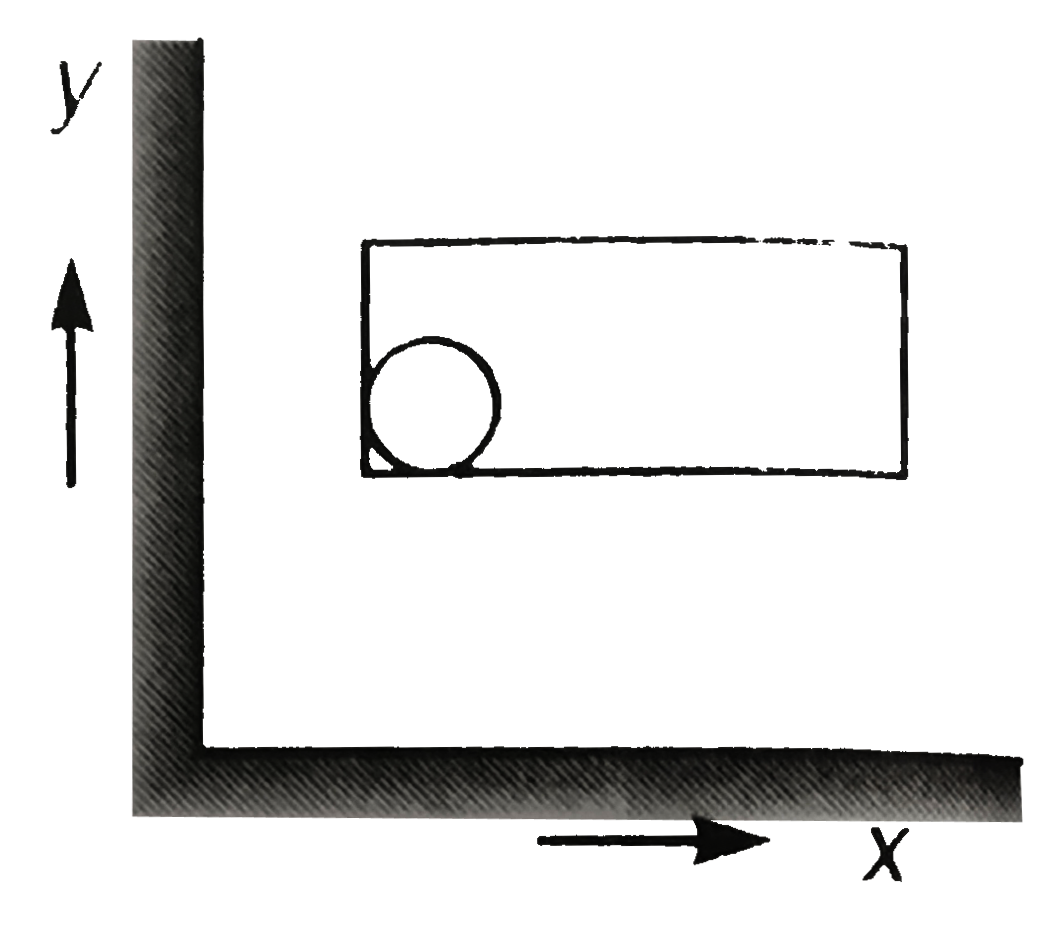
View Solution