A boy runs on a horizontal road with a speed of 4 m/s while it is raining.
He sees that the rain is making an angle θ with the vertical while running from west to east.
However , when he runs from east to west, the angle is α.
The rain is pouring down at an angle 45∘ with the vertical normal and at a speed of 8 m/s as shown in the figure. the ratio tanθtanα is
 Solution in Telugu
Solution in Telugu
(1−√2)2
(1+√2)2
(1+√2)
(√2−1)
The correct Answer is:B
A relative motion between rain and boy is shown in the figure below.
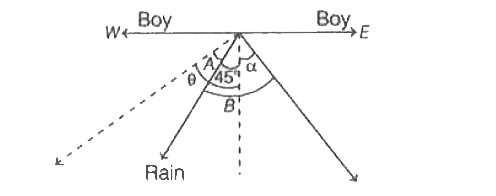
Given , speed of the boy on horizontal road is v= 4m/s
The boy is runs from east to west , the angle is = α the rain is pouring down at an angle = 45∘
Now, tanA=4sin45∘8−4cos45∘=4(1√2)8−4(1√2)=12√2−1
Similarly , tanB=4sin(90∘+45∘)8−4cos(90∘+45∘)=12√2+1
As from figure,
tanθ=tan(A+45∘)=tanA+tan45∘1−tanAtan45∘
⇒tanθ=1+2√2−12√2−12√2−1−12√2−1=2√22√2−2=√2√2−1
Similarly, tanα=tan(β−45∘)=−2√22+2√2=−√2(1+√2)
Hence, tanθtanα=(1+√21−√2)×(1+√21+√2)=(1+√2)2
So, the correct option is b
Topper's Solved these Questions
Similar Questions
Match the following fundamental forces of nature with their relative strength.
List-IList-II(A)Strong nuclear force(i)10−2(B)Weak nuclear force(ii)1(C)Elextromagnetic force(iii)10−39(D)Gravitational force(iv)10−13
The correct match is
View SolutionIdentify the correct option.
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionRain, pouring down at an angle alpha with the vertical has a speed of 10ms^(-1) . A girl runs against the rain with a speed of 8ms//^(-1) and sees that the rain makes an angle beta with the vertical, then relation between alpha and beta is
View SolutionRain is falling vertically with a speed of 30 m/s. from East to West direction. At what the angle with the vertical, he sees the rain falling?
View SolutionA man running at a speed of 5 kmph finds that the rain falls vertically. When he stops running, he finds that the rain is falling at an angle of 60° with the horizontal. The velocity of rain with respect to running man is
View SolutionA boy is running on a horizontal road with a velocity of 5 m/s. At what angle should he hold his umbrella in order to protect him self from the rain if the rain is falling vertically with a velocity of 10 m/s?
View SolutionRain, pouring down at an angle alpha with the vertical has a speed of 10 m/s. A girl runs against the rain with a speed of 8 m/s and sees that the rain makes an angle beta with the vertical, then relation between alpha and beta is
View SolutionRain, pouring down at an angle a with the vertical has a speed of 10 m/s. A girl runs against the rain with a speed of 8 m/s and sees that the rain makes an angle β with the vertical, then relation between αandβ is
View SolutionRain, pouring down at an angle α with the vertical has a speed of 10 m/s. A girl runs against the rain with a speed of 8 m/s and sees that the rain makes an angle β with the vertical, then relation between α and β
View SolutionRain, pouring down at an angle alpha with the vertical has a speed of 10 m/s. A girl runs against the rain with a speed of 8 m/s and sees that the rain makes an angle beta with the vertical, then relation between alpha and beta
View SolutionA man running on a horizontal road at 8kmph finds that rain is falling vertically. He increases the speed to 12kmphr and find that the drops make angle 30∘ with the vertical.
View SolutionA man standing still finds rain pouring at an angle 30∘with vertical , when he starts walking forward at a speed of 4 kmph it appears to him that rain pours vertically down . When he runs at riple the speed, what is the angle at which rain appears to pour?
View SolutionRain, pouring down at an angle alpha with the vertical has a speed of 10 m/s. A girl runs against the rain with a speed of 8 m/s and sees that the rain makes an angle beta with the vartical, then relation between alpha and beta is
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View Solution
