Two circular loops L1andL2 of wire carrying equal and opposite currents are placed to each other with a common axis. The radius of loop L1 is R1 and that of L2 is R2. The distance between the centres of the loops is √3R. The magnetic field at the centre of L2 shall be zero if
R2=4R1
R2=2R1
R2=√2R1
R2=8R1
The correct Answer is:A
Net magnetic field at centre, Bnet=B1−B2
For magnetic field Bnet to be zero, B1=B2
Magnetic field at the axis of coil 1 at a distance of √3R1,
B1=μ0I2πR214π(R21+(3R1)2)3/2
B1=μ0i16R1 ...(1)
Magnetic field at the centre of coil 2,B2=μ0i2R2 ...(2)
On equationg (1) and (2),
μ0i16R1=μ0i2R2
⇒R2=8R1
Topper's Solved these Questions
- EAMCET - 2018 (TS) SHIFT - 1BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 1EXERCISE - EXERCISE - Chemistry
8 Videos
- EAMCET - 2018 (TS) SHIFT - 1BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 1EXERCISE - EXERCISE - Chemistry
8 Videos
- DIFFERENTIATION BOOK - SIA PUBLICATIONCHAPTER - DIFFERENTIATION EXERCISE - Problems
50 Videos
- EAMCET - 2018 (TS) SHIFT - 2BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 2EXERCISE - Chemistry
18 Videos
Similar Questions
Knowledge Check
A square loop of edge 'a' carries a current l. The magnetic field at the centre of loop is
Aμ0l2√2πaB2√2μ0lπaCμ0l√2πaDμ0l√2πaAn are of a circle of a radius R subtends an angle π2 at the centre .it carries a current l. The magnetic field at the centre will be
Aμ0I2RBμ0I8RCμ0I4RDμ0I5RA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
Aindependent of LBproportional LCinversely proportional to LDlinearly proportional to L
Two coaxial circular loops L1 and L2 of radii 3cm and 4cm are placed as shown in figure. What should be the magnitude and direction of the current in the loop L2 so that the net magnetic field at the point O be zero?
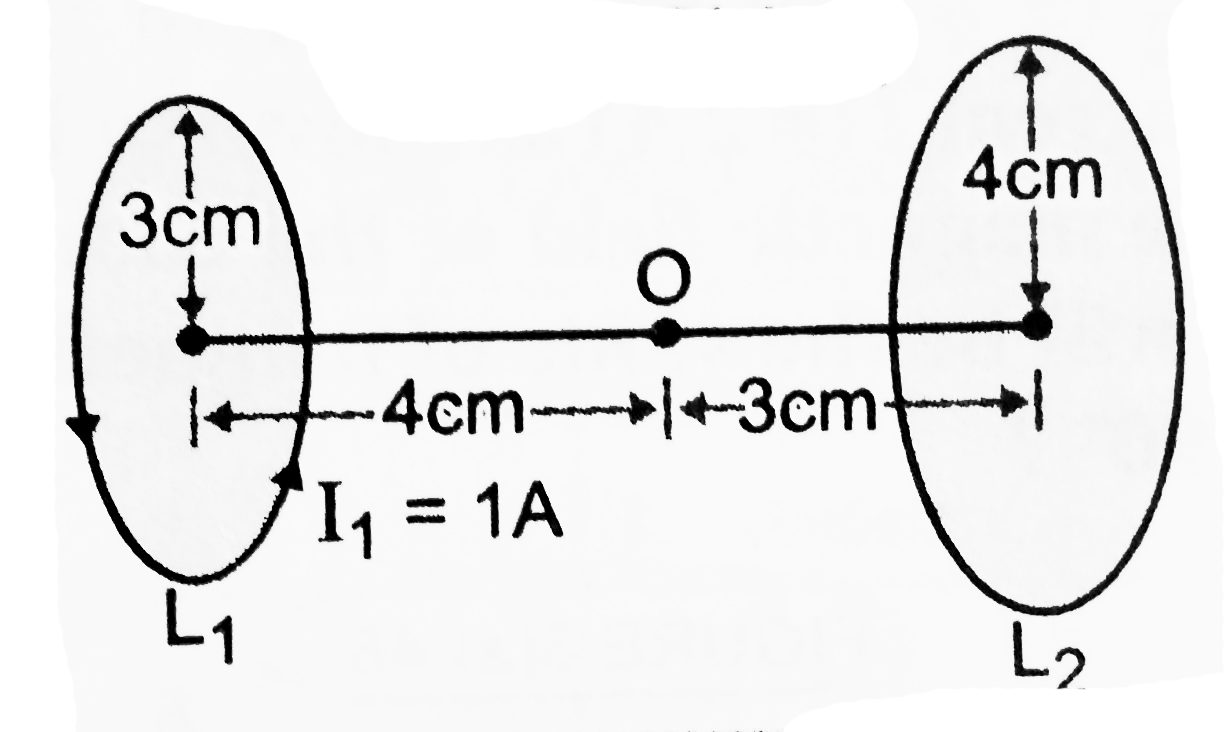
View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionConsidering magnetic field along the axis of a circular loop of radius R, at what distance from the centre of the loop is the field one eighth of its value at the centre ?
View SolutionA star shaped loop ( with l= length of each section ) carries current i. Magnetic field at the centroid of the loop is
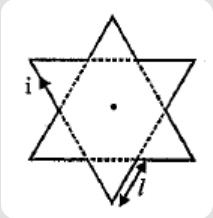
View Solution
