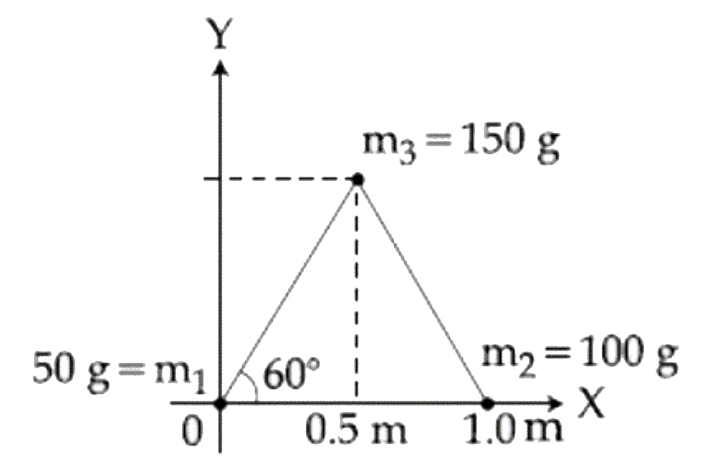
Three balls A,B and C of masses 50 g , 100 g and 150 g respectively are placed at the verticles of an equilateral triangle . The length of each side is 1 m . If A is placed at (0,0) and B is placed at (1,0) m , find the coordinates , (x,y) for the centres of mass of this system of balls
(712,√34)m
(518,√14) m
(712,√32) m
(518,√34)m
The correct Answer is:A
The diagram representing the given situation is shown in figure,
AP=0.5 m (∵ AC=1m ⇒AP12=AC=0.5)
From △le ACP
AC2=AP2+CP2
12=0.52+CP2
CP2=1−0.52
CP2=34
CP=√32
Coordinates of C =(0.5,√32)
The centroid of a triangle is given by,
(x,y)=(m1x1+m2x2+m3x3m1+m2+m3,m1y1+m2y2+m3y3m1+m2+m3)
Here,
m1=50 g (x1,y1) = (0,0)
m2 = 100 g (x2,y2) = (1,0)
m3 = 150 g (x3,y3)=(0.5,√32)
⇒(x,y)=⎛⎜⎝50×0+100×1+150×0.550×100+150,50×0+100×0+150×√3250+100+150⎞⎟⎠
=(0+100+75300,0+0+75√3300)
=(175300,75√3300)
=(712,√34) m
Topper's Solved these Questions
- EAMCET-2019 (TS) SHIFT-1BOOK - SIA PUBLICATIONCHAPTER - EAMCET-2019 (TS) SHIFT-1EXERCISE - CHEMISTRY
10 Videos
- EAMCET-2019 (TS) SHIFT-1BOOK - SIA PUBLICATIONCHAPTER - EAMCET-2019 (TS) SHIFT-1EXERCISE - CHEMISTRY
10 Videos
- EAMCET QUESTION PAPER 2017BOOK - SIA PUBLICATIONCHAPTER - EAMCET QUESTION PAPER 2017EXERCISE - CHEMISTRY
6 Videos
- FUNCTIONS AND MATHEMATICAL INDUCTION BOOK - SIA PUBLICATIONCHAPTER - FUNCTIONS AND MATHEMATICAL INDUCTION EXERCISE - Problems
58 Videos
Similar Questions
Three particles of massses 50 g, 100 g and 150 g are placed at the vertices of an equilateral triangle of side 1m ( as shown in the figure ) . The ( x, y) coordinates of the centre of mass will be :
View SolutionThree particles A(50g),B(100g) and C(150g) are placed at vertices of an equilateral triangle as shown in figure. Then the coordinates of center of mass of system is :
_2019_CBT8_E01_014_Q01.png)
View Solution
Knowledge Check
Three particles of massses 50 g, 100 g and 150 g are placed at the vertices of an equilateral triangle of side 1m ( as shown in the figure ) . The ( x, y) coordinates of the centre of mass will be :
A(712m,√34m)B(712m,√38m)C(√34m,512m)D(√38m,712m)Three charges each20μC are placed at the corners of an equilateral triangle of side 0.4m The potential energy of the system is
A18×10−6JB9JC9×10−6JD27JThree particles A(50g),B(100g) and C(150g) are placed at vertices of an equilateral triangle as shown in figure. Then the coordinates of center of mass of system is :
A(712√34)cmB(√34,712)cmC(716√38)cmD(√38,716)cm
Three particles A(50g),B(100g) and C(150g) are placed at vertices of an equilateral triangle as shown in figure. Then the coordinates of center of mass of system is :
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View SolutionThree particles of masses 0.50 kg, 1.0 kg and are placed at the corners of a right angle triangle, as shown in fig. Locate the centre of mass of the system.
View SolutionThree particles of masses 0.50 kg, 1.0 kg and are placed at the corners of a right angle triangle, as shown in fig. Locate the centre of mass of the system.
View SolutionTwo balls of mass m each are placed at the two vertices of an equilateral triangle. Another ball of mass 2m is placed at the third vertex of the triangle. Locate the centre of mass of the system.
View Solution