A block of mass m=25 kg sliding on a smooth horizontal mg surface with a velocity v = 3 ms' meets the spring of 4d spring constant k = 100 Nm' fixed at one end as shown in figure. The maximum compression of the spring and 23. Consider the following statements A and B and identify velocity of block as it returns to the original position the correct answer respectively are

The correct Answer is:a
The kinetic energy of a block when strikes the spring converts into potenial energy, ie,.
(x-compression in spring)
when the body returns to its original position potential energy is converted back into Kinectic energy. Therefore velocity of the block is same as before but nin opposite direction.
Topper's Solved these Questions
Similar Questions
A block of 10 g slides on smooth horizontal surface with 20 m/s towards a spring of spring constant 100 N/m placed horizontally (as shown in figure). The maximum compression in spring is

View SolutionA block of mass m sliding n a smooth horizontal surface with velocity →v meets a long horizontal spring fixed ast one end and having spring constant k as shown n figure. Find the maximum compression of the spring. Will the velocity of the block be the same as v whenit comes back to the original position shown?

View Solution
Knowledge Check
A block of mass m=25kg on a smooth horizontal surface with a velocity →v=3ms−1 meets the spring of spring constant k=100N/m fixed at one end as shown in figure. The maximum compression of the spring and velocity of block as it returns to the original position respectively are.
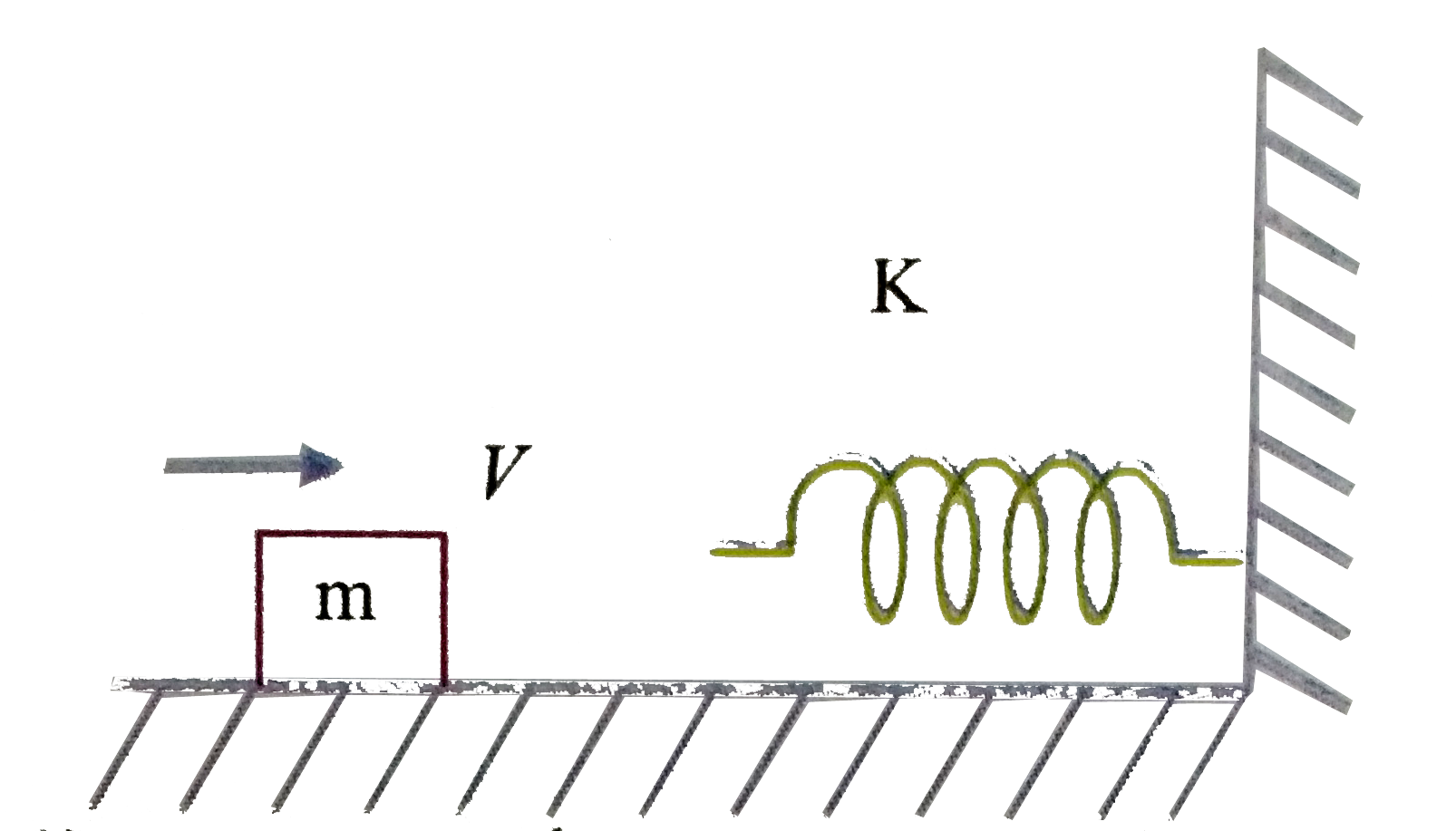 .A1.5m,−3ms−1B1.5m,0ms−1C1.0m,3ms−1D0.5m,2ms−1
.A1.5m,−3ms−1B1.5m,0ms−1C1.0m,3ms−1D0.5m,2ms−1A block of 10 g slides on smooth horizontal surface with 20 m/s towards a spring of spring constant 100 N/m placed horizontally (as shown in figure). The maximum compression in spring is
 A2 mB0.2 mC20 mD43m
A2 mB0.2 mC20 mD43mA solid cylinder of mass 3kg is rolling on a horizontal surface with velocity 4ms−1. It collides with a horizontal spring of force constant 200Nm−1. The maximum compression producec in the spring will be :
A0.5mB0.6mC0.7mD0.2m
A block of mass m sliding n a smooth horizontal surface with velocity →v meets a long horizontal spring fixed ast one end and having spring constant k as shown n figure. Find the maximum compression of the spring. Will the velocity of the block be the same as v whenit comes back to the original position shown?

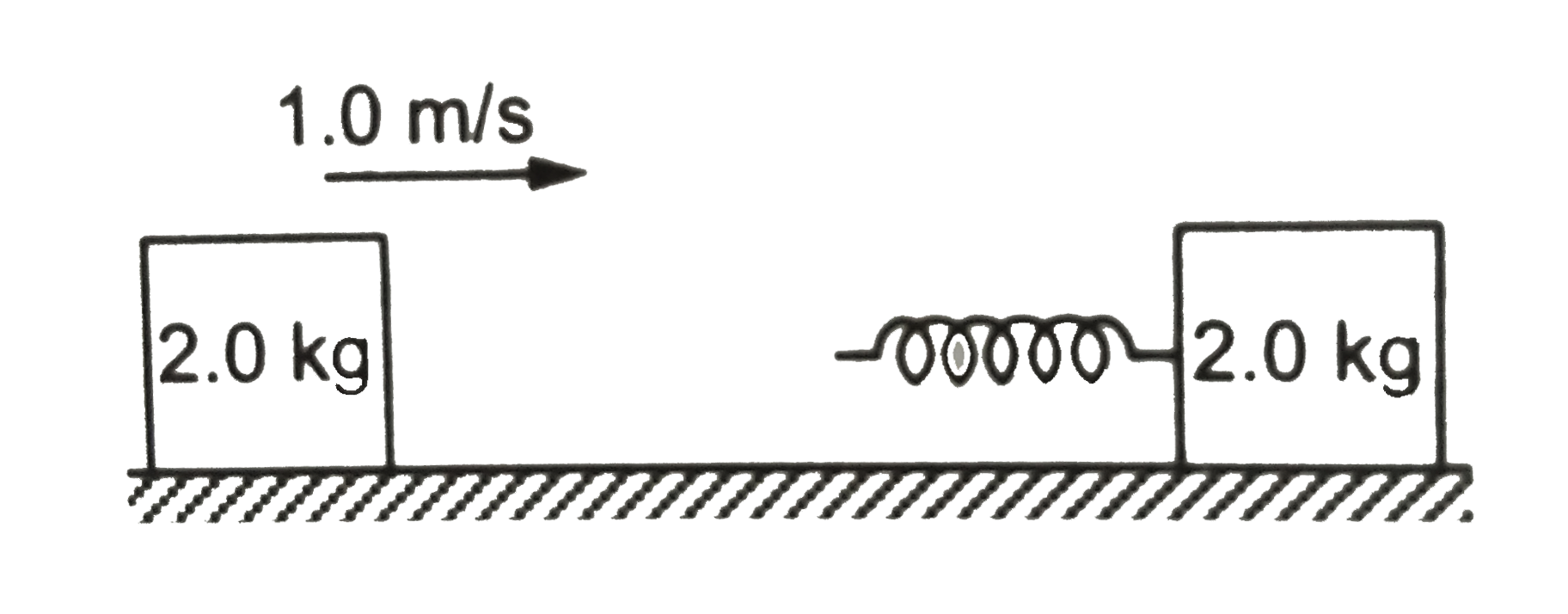
View SolutionA block of mass 2 kg is moving on a frictionless horizontal surface with a velocity of 1m/s towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100N/m. Find the rnaximum compression of the spring
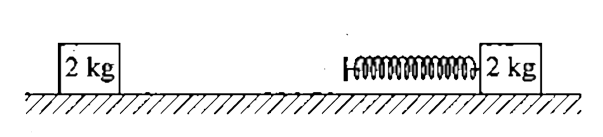
View SolutionA block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s figure towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 N/m. Find the maximum compression of the spring.
View SolutionA solid cylinder of mass 3kg is rolling on a horizontal surface with velocity 4ms−1. It collides with a horizontal spring of force constant 200Nm−1. The maximum compression produced in the spring will be :
View SolutionA block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. The maximum compression in spring is

View Solution
