If X is a discete random variable, then show that E(X−¯¯¯X)=0.Solution in Bengali
Step by step video & image solution for If X is a discete random variable, then show that E(X-barX)=0. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER 2017EXERCISE - UNIT -101videos
QUESTION PAPER 2017
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER 2017EXERCISE - UNIT -111videos
QUESTION PAPER 2017
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER 2017EXERCISE - UNIT -81videos
QUESTION PAPER 2017
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER -2018EXERCISE - WBJEE45videos
QUESTION PAPER -2018
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTIONS PAPER -2019EXERCISE - WBJEE 201945videos
QUESTIONS PAPER -2019
Similar Questions
A man is known to speak the truth 3 out of 4 times. He throws an unbaised die and reports that it is a six. Find the probability that it is a actually six.
01:12View SolutionIf the sum of the mean and variance of a binomial distribution for 5 random trials is 1.8, then find the binomal distribution.
01:25View SolutionIf P(A)=23,P(B)=12 and P(A∩B)=12,then find the value of P(A∩Bc).
03:02View SolutionThe approximate value of sin31∘ is
01:27View Solutionlimx→0+(xnlnx),n>0
01:46View SolutionFor a random variable X, E(X)=3 and E(X2)=11 Then, variance of X is
02:24View SolutionFor a random variable X, if E(X) = 5 and V(X) = 6, then E(X2) is equal to
03:01View SolutionA random variable X has the following probability distribution:
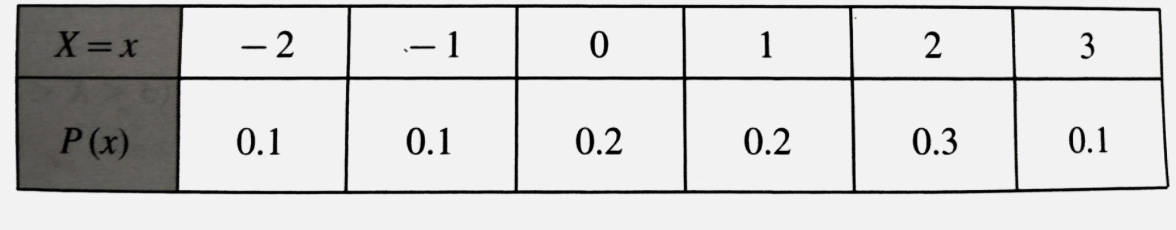
Then E(X) = …….05:12View SolutionFor a random variable X, if E(X2) = 31 , Var(X) = 6, then E(X)=.........
01:50View SolutionLet the p.m.f. of a random variable X be -
P(x)=3−x10 for x=−1,0,1,2
= 0 otherwise
Then E(X) is ………… .01:33View SolutionLet X be the random variable of the number of points obtained in single throw of an unbiased die. Then, the value of ¯¯¯X is -
03:14View SolutionIf random variable x satisfying binomial distribution and its probability distribution f(x), thenf(x)=nCxpxqn−x(x=0,1,2,......,n) and ¯x=E(x)
Statement I: E(x2)=n(n−1)p2+np
Statement II: E(x)=np.04:54View SolutionIf x is a discrete random variable and 'a' is a constant show that
(i) E(a)=a
(ii) E(ax)=aE(x)
(iii) E(x−¯¯¯X)=002:02View Solutionযদি X একটি বিচ্ছিন্ন সমসম্ভব চলক ও a একটি ধ্রুবক রাশি হয়, তবে দেখাও যে E(x−¯¯¯X)=0
01:51View Solutionসম্পর্ক প্রমাণ করো: E(x−¯x)=0, যেখানে ¯x=x চলকের মধ্যক।
03:10View Solution(x−¯x)2 -এর প্রত্যাশিত মান দ্বারা কোনো চলক x -এর ভেদমানের সংজ্ঞা দেওয়া হয়, যেখানে¯x হল x -এর মধ্যক। প্রমাণ করো যে x -এর ভেদমান = E(x2)−{E(x)}2
01:16View Solution

