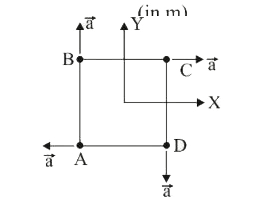
Four particles A, B, C and D with masses mA=m,mB=2m,mC=3mandmD=4m are at the corners of a square. They have accelerations of equal magnitude with directions as shown. The acceleration of the centre of mass of the particles is:
a5(ˆi−ˆj)
a(ˆi+ˆj)
a5(ˆi+ˆj)
zero
The correct Answer is:A
¯aCM=m1¯a1+m3¯a2+m3¯a3+m4¯a4m1+m2+m3+m4=m(−aˆi)+2m(aˆj)+3m(aˆi)+4m(−aˆj)m+2m+3m+4m=2aˆi−2aˆj10=a5(ˆi−ˆj)
Similar Questions
Four particles A,B,CandD of masses m,2m,3mand4m respectively are placed at corners of a square of side x as shown in Fig.
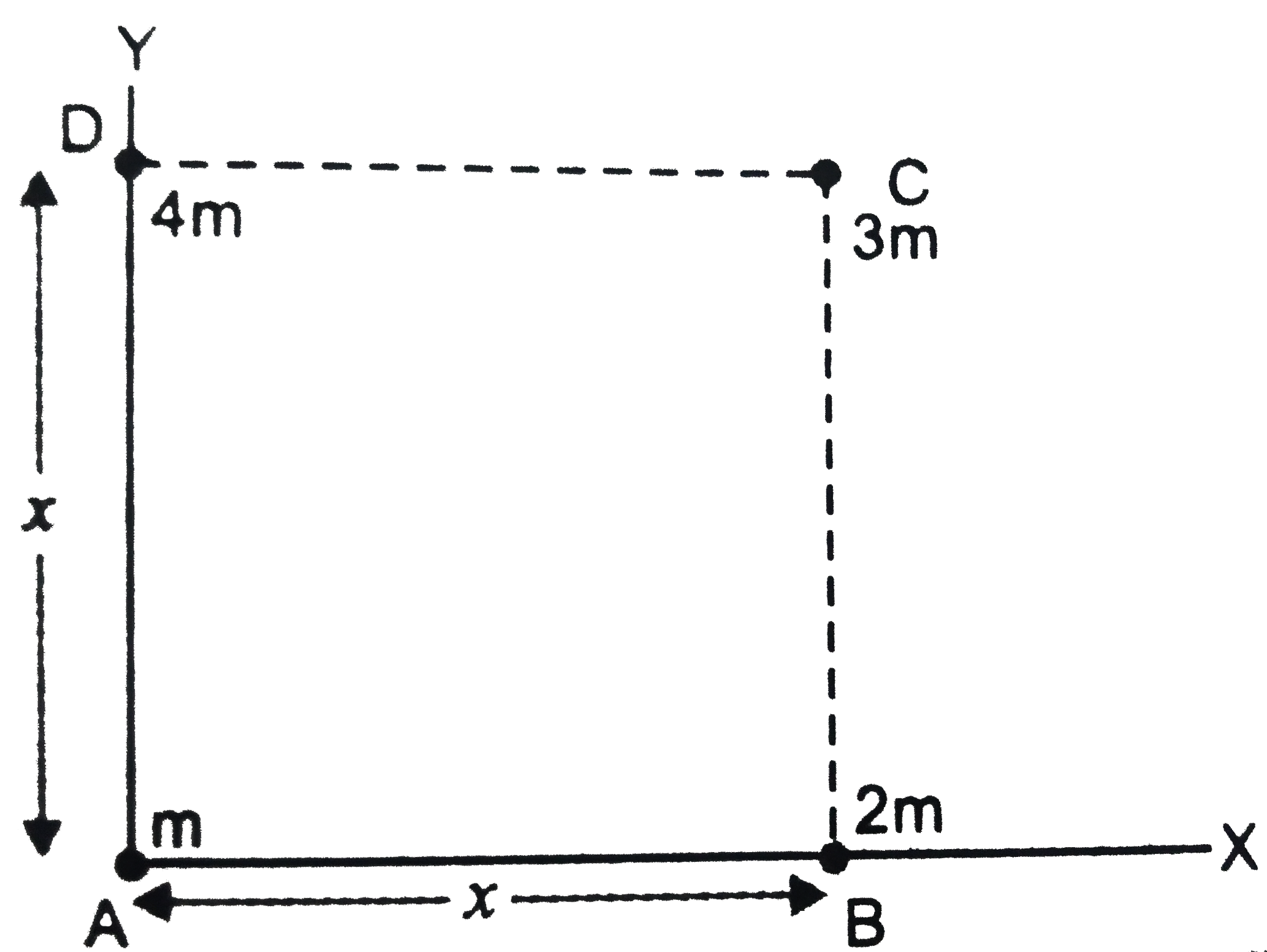
Locate the centre of mass.
View SolutionFour particles of masses m, m, 2m and 2m are placed at the corners of a square of side a, as shown in fig. Locate the centre of mass.
View Solution
Knowledge Check
Four particle of masses m1=2m,m2=4m,m3=mandm4 are placed at four corners of a square. What should be the value of m4 so that the centres of mass of all the four particle are exactly at the centre of the square ?
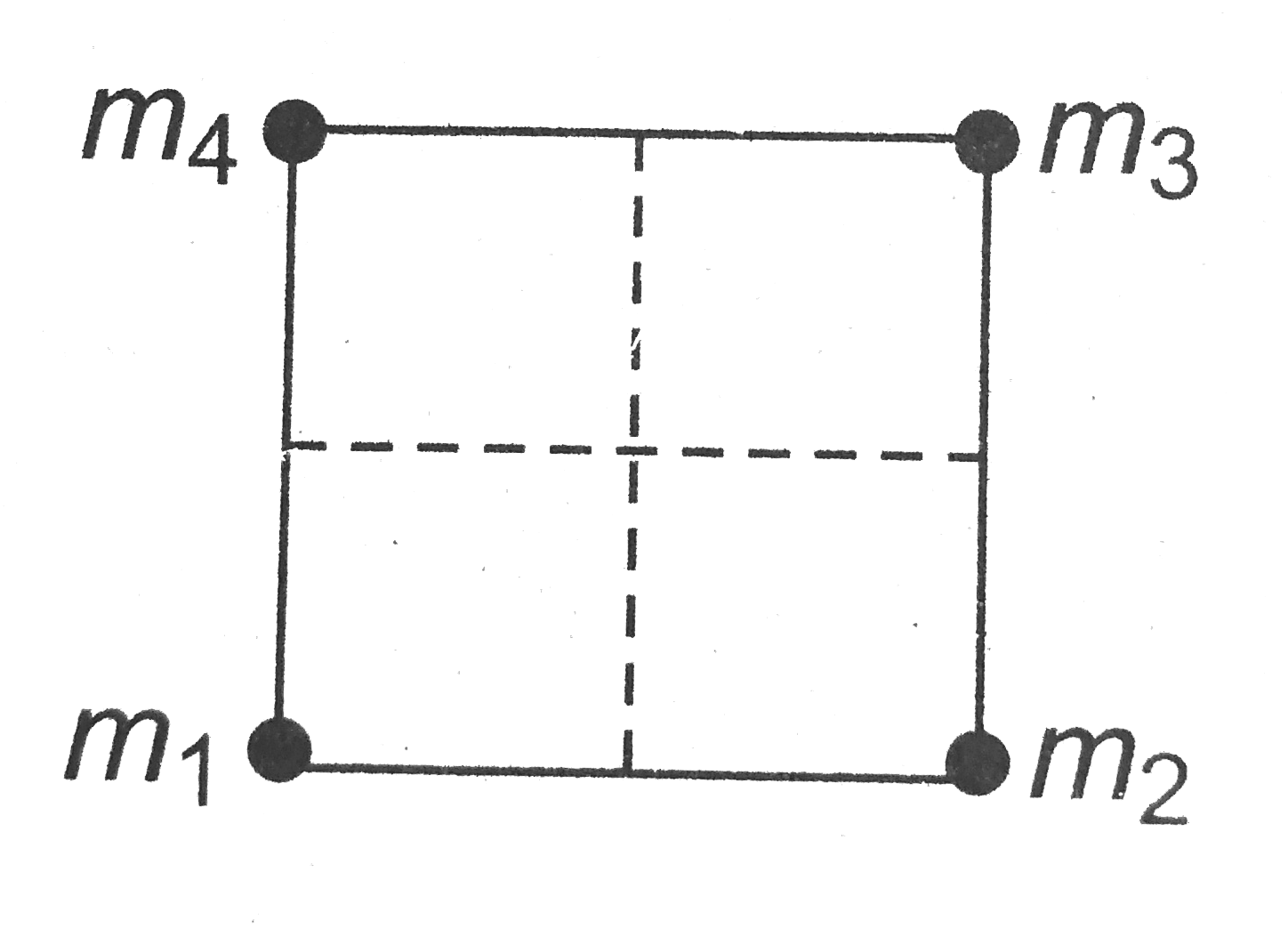 A3 mB5 mC8 mDnone of these
A3 mB5 mC8 mDnone of theseIn the figure shown, the acceleration of block of mass m2 is 2m/s2. The acceleration of m1 :
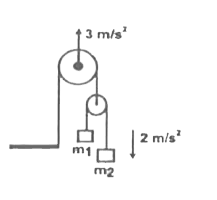 Awill be 8m/s2 upwardBwill be 4m/s2 upwardCwill be 14m/s2 upwardDThe value depends upon actual values of m1 and m2
Awill be 8m/s2 upwardBwill be 4m/s2 upwardCwill be 14m/s2 upwardDThe value depends upon actual values of m1 and m2Four particles of masses m, 2m, 3m and 4m are arranged at the corners of a parallelogram with each side equal to a and one of the angle between two adjacent sides as 60∘ The parallelogram lies in the x-y plane with mass m at the origin and 4m on the r-axis. The centre of mass of the arrangement will be located at
A(√32a,0.95a)B(0.95a,√34a)C(3a4,a2)D(a2,3a4)
If four different masses m1,m2,m3 and m4 are placed at the four corners of a square of side a, the resultant gravitational force on a mass m kept at the centre is
View SolutionIf two particles of masses m1 and m2 are projected vertically upwards with speed v1 and v2, then the acceleration of the centre of mass of the system is
View SolutionFour particles of masses m, m, 2m and 2m are placed at the four corners of square of side a. Find the centre of mass of the system.
View SolutionFour particles of masses m, 2m, 3m and 4m are arranged at the corners of a parallelogram with each side equal to a and one of the angle between two adjacent sides is 60∘. The parallelogram lies in the x-y plane with mass m at the origin and 4 m on the x-axis. The centre of mass of the arrangement will be located at
View SolutionTwo particles of mass M and 2M are at a distance D apart. Under their mutual force of attraction they start moving towards each other. The acceleration of their centre of mass when they are D/2 apart is :
View Solution
