A cylindrical tank of height H is completely filled with water. On its vertical side there are two tiny holes, one above the middle at a height h1 and the other below the middle at a depth h2 If the jets of water from the holes meet at the same point at the horizontal plane through the bottom of the tank then the ratio h1/h2 is :
1
2
3
4
The correct Answer is:A
To solve the problem, we need to analyze the situation involving the cylindrical tank with two holes at different heights. The goal is to find the ratio h1h2 given that the jets of water from the holes meet at the same point on the horizontal plane through the bottom of the tank.
1. Understanding the Setup:
- The cylindrical tank has a height H and is filled with water.
- There are two holes: one at height h1 above the middle of the tank and the other at depth h2 below the middle.
2. Identifying Heights:
- The middle of the tank is at height H2.
- The height of the first hole from the bottom is H2+h1.
- The height of the second hole from the bottom is H2−h2.
3. Using Torricelli’s Law:
- The velocity of water flowing out of a hole can be derived from Torricelli's theorem, which states that the speed v of efflux of a fluid under gravity through an orifice is given by:
v=√2gh
- For the first hole (at height h1):
v1=√2g(H2+h1)
- For the second hole (at depth h2):
v2=√2g(H2−h2)
4. Calculating the Range:
- The horizontal range R for a projectile is given by:
R=v⋅t
- The time t taken for the water to fall from height h is given by:
t=√2hg
5. Setting Up the Equations:
- For the first hole, the range R1 can be expressed as:
R1=v1⋅t1=√2g(H2+h1)⋅
⎷2(H2+h1)g=2(H2+h1)
- For the second hole, the range R2 is:
R2=v2⋅t2=√2g(H2−h2)⋅
⎷2(H2−h2)g=2(H2−h2)
6. Equating the Ranges:
- Since the jets meet at the same point:
R1=R2
- This gives us:
2(H2+h1)=2(H2−h2)
- Simplifying this equation:
H2+h1=H2−h2
- Rearranging gives:
h1+h2=0⇒h1=h2
7. Finding the Ratio:
- Therefore, the ratio h1h2 is:
h1h2=1
Final Answer:
The ratio h1h2 is 1.
- The cylindrical tank has a height H and is filled with water.
- There are two holes: one at height h1 above the middle of the tank and the other at depth h2 below the middle.
- The middle of the tank is at height H2.
- The height of the first hole from the bottom is H2+h1.
- The height of the second hole from the bottom is H2−h2.
- The velocity of water flowing out of a hole can be derived from Torricelli's theorem, which states that the speed v of efflux of a fluid under gravity through an orifice is given by:
v=√2gh
- For the first hole (at height h1):
v1=√2g(H2+h1)
- For the second hole (at depth h2):
v2=√2g(H2−h2)
- The horizontal range R for a projectile is given by:
R=v⋅t
- The time t taken for the water to fall from height h is given by:
t=√2hg
- For the first hole, the range R1 can be expressed as:
R1=v1⋅t1=√2g(H2+h1)⋅
⎷2(H2+h1)g=2(H2+h1)
- For the second hole, the range R2 is:
R2=v2⋅t2=√2g(H2−h2)⋅
⎷2(H2−h2)g=2(H2−h2)
- Since the jets meet at the same point:
R1=R2
- This gives us:
2(H2+h1)=2(H2−h2)
- Simplifying this equation:
H2+h1=H2−h2
- Rearranging gives:
h1+h2=0⇒h1=h2
- Therefore, the ratio h1h2 is:
h1h2=1
The ratio h1h2 is 1.
Similar Questions
A jet of water issunig from a hole mear the bottom of water tank is an…………………………. .
View SolutionA water tank placed on the floor has two small holes, pinched in the vertical wall, one above the other. The holes are 3.3cm and 4.7cm above the floor. If the jets of water issuing out from the holes hit the floor at the same point on the floor, then the height of water in the tank is
View Solution
Knowledge Check
- Question 1 - Select One
A water tank of height H, completely filled with water is placed on a level ground. It has two holes one at a depth h from top and the other at height h form its base. The water ejecting from
Aboth the holes will fall at the same spotBupper hole will fall farther than that form the lower holeCupper hole will fall closer than that form the lower holeDmore information is required. - Question 2 - Select One
A water tank of height 10m, completely filled with water is placed on a level ground. It has two holes one at 3m and the other at 7m form its base. The water ejecting from
Aboth the holes will fall at the same spotBupper hole will fall farther than that form the lower holeCupper hole will fall closer than that form the lower holeDmore information is required. - Question 3 - Select One
A water tank standing on the floor has two small holes punched in the vertical wall one above the other. The holes are 2.4 cm and 7.6 cm above the floor. If the jest of water from the holes hit the floor at the same point, then the height of water in the tank is
A10 cmB5 cmC20 cmD4.8 cm
Two holes are made in the side of the tank such that the jets of water flowing out of them meet at the same point on the ground. If one hole is at a height of 3cm above the bottom, then the distance of the other holes from the top surface of water is
View SolutionA tank of height H is fully filled with water. If the water rushing from a hole made in the tank below the free surface , strikes the floor at a maximum horizontal distance , then the depth of the hole from the free surface must be
View SolutionA cylindrical tank of height H is open at the top end and it has a radius r. Water is filled in it up to a height of h. The time taken to empty the tank through a hole of radius r' in its bottom is
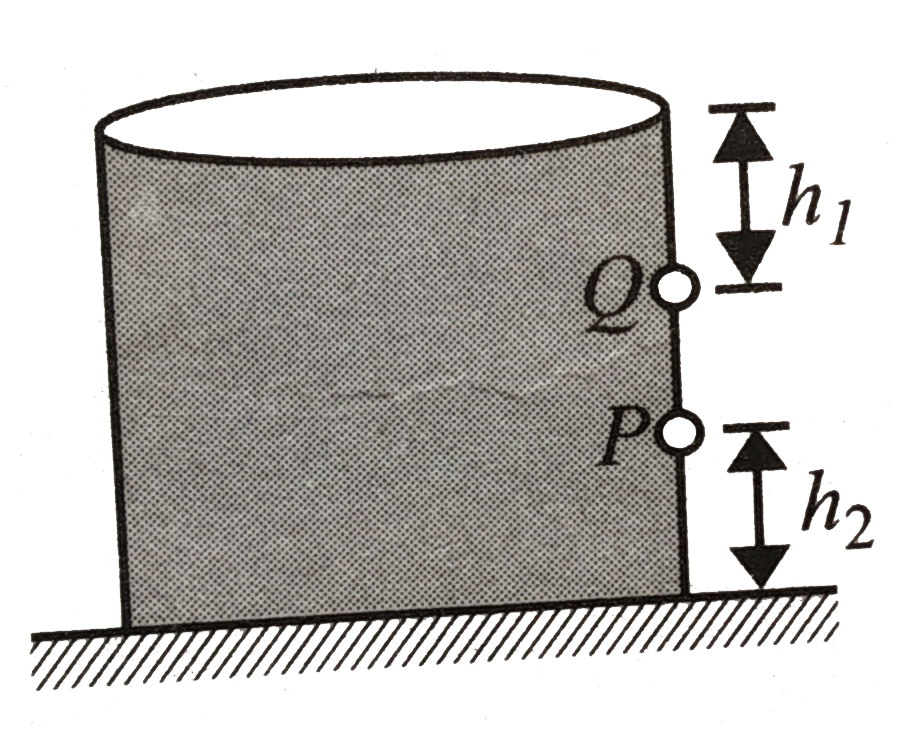
View SolutionIn a cylindrical water tank there are two small holes Q and P on the wall at a depth of h1 from the upper level of water and at a height of h2 from the lower end of the tank, respectively, as shown in the figure. Water coming out from both the holes strike the ground at the same point. The ratio of h1 and h2 is
 View Solution
View SolutionThe level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
View Solution

