A parallel plate capacitor each with plate area A and separation ‘d’ is charged to a potential difference V. The battery used to charge it is then disconnected. A dielectric slab of thickness d and dielectric constant K is now placed between the plates. What change if any, will take place in
(i) charge on the plates
(ii) electric field intensity between the plates,
(iii) capacitance of the capacitor
Justify your answer in each case
To solve the problem step by step, we will analyze the situation of a parallel plate capacitor before and after inserting a dielectric slab.
Given:
- Plate area of the capacitor = A
- Separation between plates = d
- Initial potential difference = V
- Dielectric slab thickness = d
- Dielectric constant of the slab = K
Step 1: Initial Charge on the Capacitor Plates
The initial capacitance C0 of the capacitor without the dielectric is given by the formula:
C0=ε0Ad
where ε0 is the permittivity of free space.
The charge Q on the plates when connected to the battery is:
Q=C0V=ε0AdV
Step 2: Charge After Disconnecting the Battery
Once the battery is disconnected, the charge on the plates remains constant. Therefore, the charge Q on the plates after disconnecting the battery is still:
Q=ε0AdV
Answer for (i): The charge on the plates remains the same.
Step 3: Electric Field Intensity Between the Plates
The electric field E between the plates without the dielectric is given by:
E=Qε0A
Substituting for Q:
E=ε0AdVε0A=Vd
When the dielectric slab is inserted, the electric field E′ becomes:
E′=QKε0A
Substituting for Q:
E′=ε0AdVKε0A=VKd
Answer for (ii): The electric field intensity decreases by a factor of K.
Step 4: Capacitance of the Capacitor with Dielectric
The capacitance with the dielectric slab C′ is given by:
C′=KC0=Kε0Ad
Answer for (iii): The capacitance increases by a factor of K.
Summary of Changes:
1. Charge on the plates: Remains the same.
2. Electric field intensity: Decreases by a factor of K.
3. Capacitance: Increases by a factor of K.
- Plate area of the capacitor = A
- Separation between plates = d
- Initial potential difference = V
- Dielectric slab thickness = d
- Dielectric constant of the slab = K
The initial capacitance C0 of the capacitor without the dielectric is given by the formula:
C0=ε0Ad
where ε0 is the permittivity of free space.
Q=C0V=ε0AdV
Once the battery is disconnected, the charge on the plates remains constant. Therefore, the charge Q on the plates after disconnecting the battery is still:
Q=ε0AdV
Answer for (i): The charge on the plates remains the same.
The electric field E between the plates without the dielectric is given by:
E=Qε0A
Substituting for Q:
E=ε0AdVε0A=Vd
E′=QKε0A
Substituting for Q:
E′=ε0AdVKε0A=VKd
Answer for (ii): The electric field intensity decreases by a factor of K.
The capacitance with the dielectric slab C′ is given by:
C′=KC0=Kε0Ad
Answer for (iii): The capacitance increases by a factor of K.
1. Charge on the plates: Remains the same.
2. Electric field intensity: Decreases by a factor of K.
3. Capacitance: Increases by a factor of K.
Similar Questions
A parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
charge on plates ?
Justify your answerView SolutionA parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
electric filed intensity between the plates ? Justify your answer .View Solution
Knowledge Check
- Question 1 - Select One
A capacitor with plate separation d is charged to V volts. The battery is disconnected and a dielectric slab of thickness d2 and dielectric constant '2' is inserted between the plates. The potential difference across its terminals becomes
AVB2 VC4V3D3V4 - Question 2 - Select One
Figure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
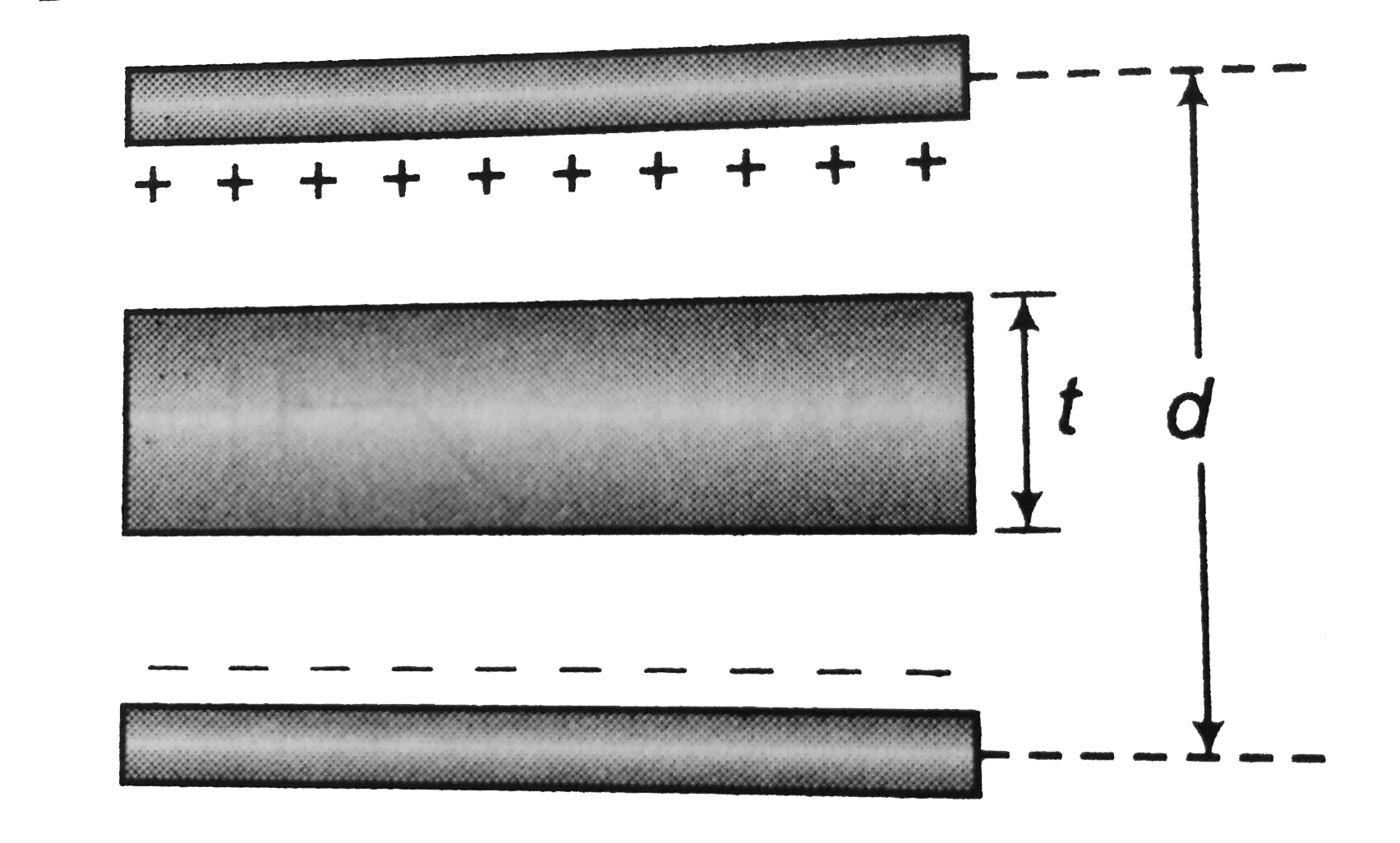
The electric field in the gaps between the plates and the electric slab will beAε0AVdBVdCKVdDVd−t - Question 3 - Select One
Figure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dielectric constant K is placed in between the plates of the capacitor as shown.
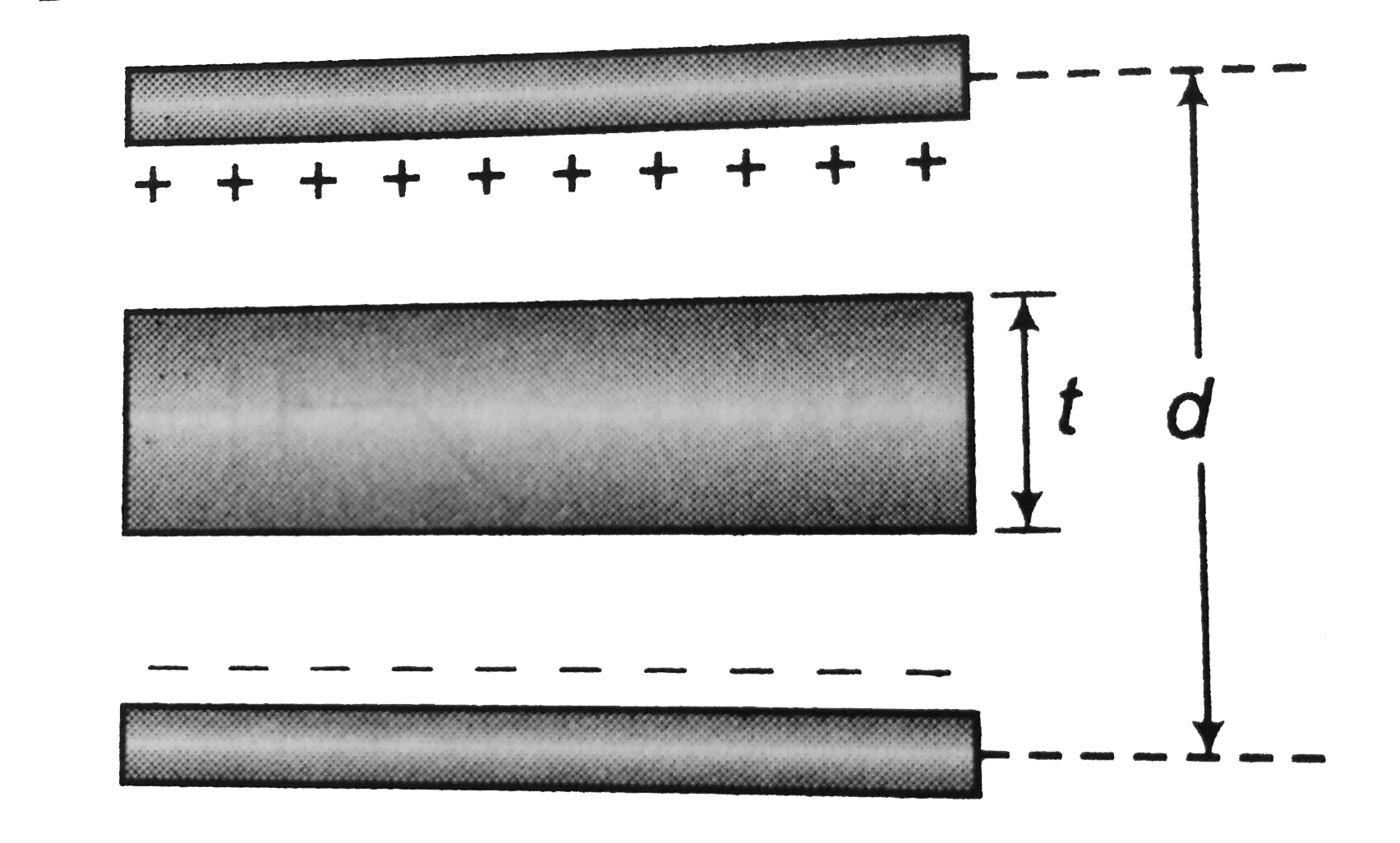
The electric field in the dielectric slab isAVKdBKVdCVdDKVt
A parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
capacitance of the capacitor ? Justify your answer .View SolutionA parallel plate capacitor having plate area A and separation between the plates d is charged to potential V.The energy stored in capacitor is ....???
View SolutionA parallel - plate capacitor of plate area Aand plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View SolutionA parallel plate capacitor is charged to a certain potential difference and the battery used to charged it is disconnected. Now a dielectric slab of dimensions equal to spacing between plates is introduced between the plates. What will be the changes, if any, in the charge, potential difference, capacitance, electric field strength and the energy stored in the capacitor?
View SolutionA parallel plate capacitor has plates with area A and separation d. A battery charges the plates to a potential difference V0. The battery is then disconnected and a dielectric slab of thikness d is introduced. The ratio of the enrgy stored in the capacitor before and after the slab is introduced, is
View Solution

