A and B two points on the curve xy=a2. Let N be the mid-point of AB. The line through A and B meets. x-axis at P and y-axis at Q, then:
N bisects PQ
ON is perpendicular to AB (where O is origin)
AP = BQ
AQ = BP
The correct Answer is:A, C, D
Similar Questions
Let A be a fixed point (0,6) and B be a moving point (2t,0). Let M be the mid-point of AB and the perpendicular bisector of AB meets the y-axis at C. The locus of the mid-point P of MC is :
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
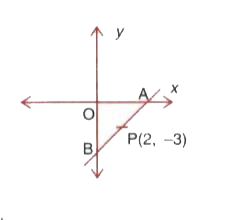
co-ordinates of A and B.
View Solution
Knowledge Check
Let P be the point (1,0) and Q a point on the locus y2=8x. The locus of mid-point of PQ is :
Ax2+4y+2=0Bx2−4y+6=0Cy2−4x+2=0Dy2+4x+2=0
A and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
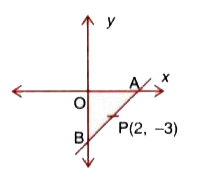
equation of line AB.
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
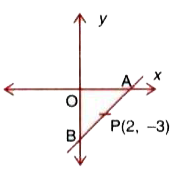
slope of line AB
View SolutionA variable line through the point (p,q) cuts the xandy axes at AandB respectively. The lines through AandB parallel to y-axis and x-axis respectively meet at P. If the locus of P is 3x+2y−xy=0, then (A) p=2,q=3 (B) p=3,q=2 (C) p=2,q=−3 (D) p=−3,q=−2
View SolutionA variable line through the point (p,q) cuts the xandy axes at AandB respectively. The lines through AandB parallel to y-axis and x-axis respectively meet at P. If the locus of P is 3x+2y−xy=0, then (A) p=2,q=3 (B) p=3,q=2 (C) p=2,q=−3 (D) p=−3,q=−2
View SolutionFind the equation of a line passing through the points (7,−3)and(2,−2). If this line meets x-axis at point P and y-axis at point Q, find the co-ordinates of points P and Q.
View SolutionP is a point on positive x-axis, Q is a point on the positive y-axis and 'O' is the origin. If the line passing through P and Q is tangent to the curve y=3−x2 then find the minimum area of the triangle OPQ , is 3 (b) 4 (c) 5 (c) 6
View SolutionThe tangent at a point 'P' of a curve meets the axis of 'y' in N, the parallel through 'P' to the axis of 'y' meets the axis of X at M, O is the origin of the area of ΔMON is constant then the curve is (A) circle C) ellipse (D) hyperbola (B) parabola
View Solution
