Question

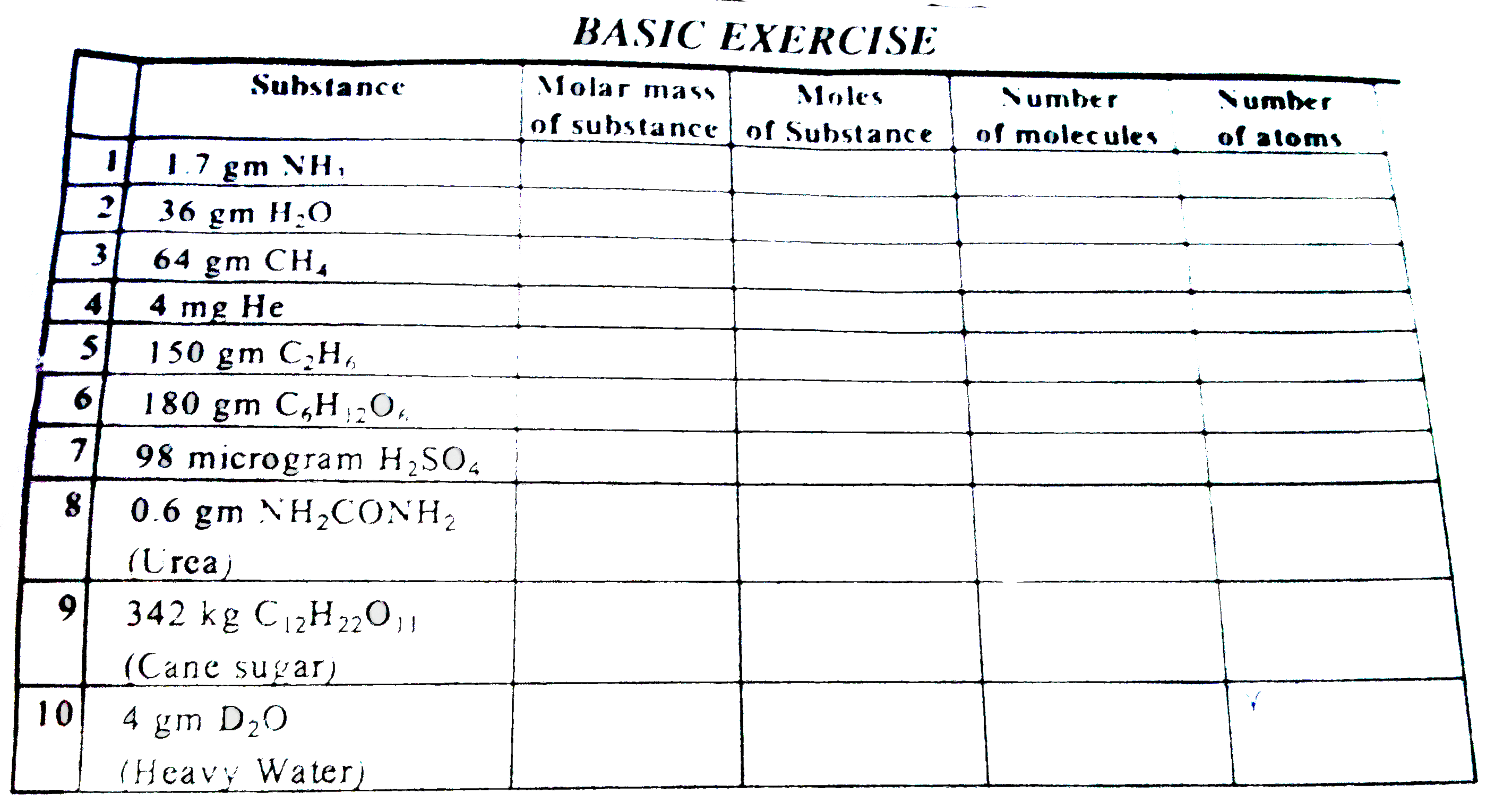
Two containers A and B have the same volume. Container A contains 5 moles of $O_2$ gas. Container B contains 3 moles of He and 2 moles of $N_2$. Both the containers are separately kept in vacuum at the same temperature. Both the containers have very small orifices of the same area through which the gases leak out. Compare the rate of effusion of $O_2$ with that of He gas mixture.

Explanation

To solve the problem of comparing the rate of effusion of $$O_2$$ gas in container A with the helium gas mixture in container B, we will follow these steps:

Step 1: Identify the Moles and Molecular Weights
- Container A: Contains \( 5 \) moles of \( O_2 \).
- Container B: Contains \( 3 \) moles of Helium (\( He \)) and \( 2 \) moles of Nitrogen (\( N_2 \)).
- Molecular weights:
- \( O_2 \): \( 32 \, g/mol \)
- \( He \): \( 4 \, g/mol \)
- \( N_2 \): \( 28 \, g/mol \)

Step 2: Calculate the Rate of Effusion of Gases in Container B
The rate of effusion is given by Graham's law, which states that the rate of effusion of a gas is inversely proportional to the square root of its molar mass.

For the gases in container B:
- Rate of effusion of \( He \) to \( N_2 \):
\( \frac{\text{Rate of } He}{\text{Rate of } N_2} = \frac{\text{moles of } He}{\text{moles of } N_2} \times \sqrt{\frac{\text{Molar mass of } N_2}{\text{Molar mass of } He}} \)
Substituting the values:
\( \frac{3}{2} \times \sqrt{\frac{28}{4}} = \frac{3}{2} \times \sqrt{7} \approx \frac{3}{2} \times 2.64575 \approx 3.96 \)

Step 3: Determine the Mole Fractions
Let \( x \) be the moles of \( N_2 \):
- Moles of \( He = 3.96x \)
- Total moles in container B = \( x + 3.96x = 4.96x \)

Now, calculate the mole fractions:
- Mole fraction of \( N_2 \):
\( \text{Mole fraction of } N_2 = \frac{x}{4.96x} = \frac{1}{4.96} \approx 0.202 \)
- Mole fraction of \( He \):
\( \text{Mole fraction of } He = \frac{3.96x}{4.96x} = \frac{3.96}{4.96} \approx 0.798 \)

Step 4: Calculate the Average Molecular Weight of the Mixture
Using the mole fractions and molecular weights:
\( \text{Molecular weight of mixture} = (0.202 \times 28) + (0.798 \times 4) \)
Calculating:
\( = 5.656 + 3.192 = 8.848 \, g/mol \approx 8.85 \, g/mol \)

Step 5: Calculate the Rate of Effusion of \( O_2 \) Compared to the Mixture
Using Graham's law again:
\( \frac{\text{Rate of } O_2}{\text{Rate of mixture}} = \sqrt{\frac{\text{Molar mass of mixture}}{\text{Molar mass of } O_2}} = \sqrt{\frac{8.85}{32}} \)
Calculating:
\( = \sqrt{0.2765625} \approx 0.525 \)

Final Result
The rate of effusion of \( O_2 \) is approximately \( 0.525 \) times that of the helium gas mixture.

---