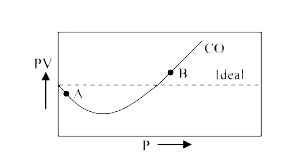
In the above Question, near the point B, compressibility factor Z is about :
(1−PbRT)
1
(1+PbRT)
(1−aRTV)
The correct Answer is:C
To determine the compressibility factor Z near point B for carbon monoxide, we can follow these steps:
Step 1: Understand the Compressibility Factor
The compressibility factor Z is defined as the ratio of the molar volume of a real gas to the molar volume of an ideal gas at the same temperature and pressure. It indicates how much a real gas deviates from ideal gas behavior. The formula is given by:
Z=PVnRT
Step 2: Use the Van der Waals Equation
The Van der Waals equation for real gases is:
(P+an2V2)(V−nb)=nRT
For our case, we consider n=1 (1 mole of carbon monoxide), which simplifies the equation to:
(P+aV2)(V−b)=RT
Step 3: Analyze Conditions at High Pressure
At high pressures (near point B), the term aV2 becomes negligible compared to P. Thus, we can ignore the pressure correction term:
P(V−b)=RT
Step 4: Rearrange the Equation
Rearranging the equation gives us:
PV−Pb=RT
This can be rewritten as:
PV=RT+Pb
Step 5: Divide by RT
Now, divide the entire equation by RT:
PVRT=1+PbRT
Step 6: Identify the Compressibility Factor
From the above equation, we can identify the compressibility factor Z:
Z=PVRT=1+PbRT
Conclusion
Thus, near point B, the compressibility factor Z is given by:
Z=1+PbRT
The compressibility factor Z is defined as the ratio of the molar volume of a real gas to the molar volume of an ideal gas at the same temperature and pressure. It indicates how much a real gas deviates from ideal gas behavior. The formula is given by:
Z=PVnRT
The Van der Waals equation for real gases is:
(P+an2V2)(V−nb)=nRT
For our case, we consider n=1 (1 mole of carbon monoxide), which simplifies the equation to:
(P+aV2)(V−b)=RT
At high pressures (near point B), the term aV2 becomes negligible compared to P. Thus, we can ignore the pressure correction term:
P(V−b)=RT
Rearranging the equation gives us:
PV−Pb=RT
This can be rewritten as:
PV=RT+Pb
Now, divide the entire equation by RT:
PVRT=1+PbRT
From the above equation, we can identify the compressibility factor Z:
Z=PVRT=1+PbRT
Thus, near point B, the compressibility factor Z is given by:
Z=1+PbRT
Similar Questions
Knowledge Check
- Question 1 - Select One
For Co, isotherm is of the type as shown. Near point A, compressibility factor Z is:
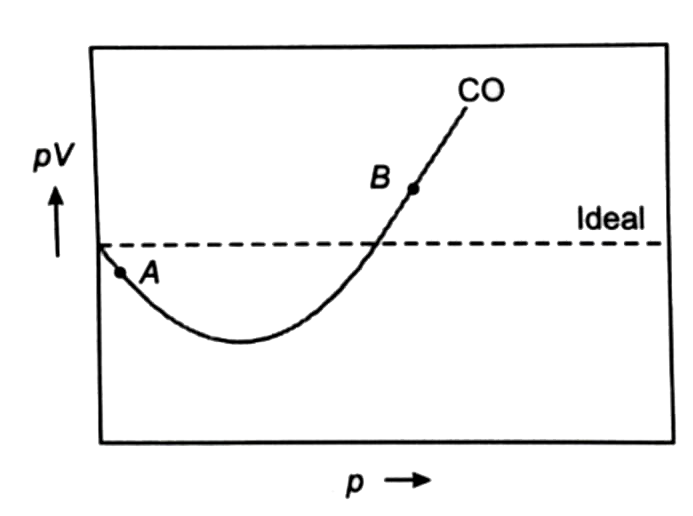 A(1+bV)B(1−bV)C(1+aRTV)D(1−aRTV)
A(1+bV)B(1−bV)C(1+aRTV)D(1−aRTV) - Question 2 - Select One
The compressibility factor for an ideal gas is
A1.5B1.0C2.0D∞ - Question 3 - Select One
Compressibility factor (Z) for H2(g) at STP is
AZ=1B[Z=1+ a/(VmRT)]C[Z=1− a/(VmRT)]D[Z=1 + Pb/Rt]
The compressibility factor for an ideal gas is
View SolutionThe compressibility factor for an ideal gas is
View SolutionThe compressibility factor for an ideal gas is .
View SolutionThe compressibility factor Z for an ideal gas will be .........
View SolutionThe compressibility factor for an ideal gas is .
View Solution