Quantity AQuantity B√30×√512
The correct Answer is:Quantity A is greater.
To solve the problem comparing Quantity A and Quantity B, we will follow these steps:
Step 1: Identify the quantities
- Quantity A = √30×√5
- Quantity B = 12
Step 2: Simplify Quantity A
We can simplify Quantity A using the property of square roots:
√30×√5=√30×5
Calculating 30×5:
30×5=150
Thus, Quantity A becomes:
√150
Step 3: Further simplify √150
We can factor 150 to simplify it further:
150=25×6=52×6
Using the property of square roots:
√150=√25×6=√25×√6=5√6
Step 4: Approximate √6
Now we need to approximate √6. The approximate value of √6 is about 2.449.
Thus:
5√6≈5×2.449=12.245
Step 5: Compare Quantity A and Quantity B
Now we compare:
- Quantity A ≈12.245
- Quantity B = 12
Since 12.245>12, we conclude that:
Quantity A is greater than Quantity B.
Final Conclusion
Thus, the final answer is that Quantity A is greater than Quantity B.
---
- Quantity A = √30×√5
- Quantity B = 12
We can simplify Quantity A using the property of square roots:
√30×√5=√30×5
Calculating 30×5:
30×5=150
Thus, Quantity A becomes:
√150
We can factor 150 to simplify it further:
150=25×6=52×6
Using the property of square roots:
√150=√25×6=√25×√6=5√6
Now we need to approximate √6. The approximate value of √6 is about 2.449.
Thus:
5√6≈5×2.449=12.245
Now we compare:
- Quantity A ≈12.245
- Quantity B = 12
Quantity A is greater than Quantity B.
Thus, the final answer is that Quantity A is greater than Quantity B.
Similar Questions
Knowledge Check
Quantity AQuantity Bx3x−4
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.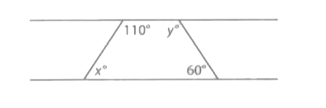
Quantity AQuantity By−x50AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.