A small ball having charge +q and mass m is suspended at one end of a light string of length and can rotate in vertical plane.A uniform electric field E exists in the region in vertically upward direction (mg>qE) .The minimum velocity required to be imparted to the ball at the lowest position in order to successfully negotiate the circular loop is √x(g−qEmy) then find x+y .x&y are lowest possible integer.
Step by step video & image solution for A small ball having charge +q and mass m is suspended at one end of a light string of length and can rotate in vertical plane.A uniform electric field E exists in the region in vertically upward direction (mg>qE) .The minimum velocity required to be imparted to the ball at the lowest position in order to successfully negotiate the circular loop is sqrt(x(g-(qE)/(my))) then find x+y .x & y are lowest possible integer. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A ball having charge q and mass m is suspended from a string of length L between two parallel plates where a vertical electric field E is established . Find the time period of simple pendulum if electric field is directed (a) downward and (b) upward.
View SolutionA small ball of mass m having charge q is suspended by a string of length L. Another identical ball having the same charge is kept at the point of suspension. Determine the minimum horizontal velocity which should be imparted to ball at lowest point so that it can make complete revolution.
View Solution
Knowledge Check
- Question 1 - Select One
A small ball of masss m and charge +q tied with a string of length l, rotating in a veticle circle under gravity and a uniform horizontal electric field E as shown. The tension in the string will be minimum for
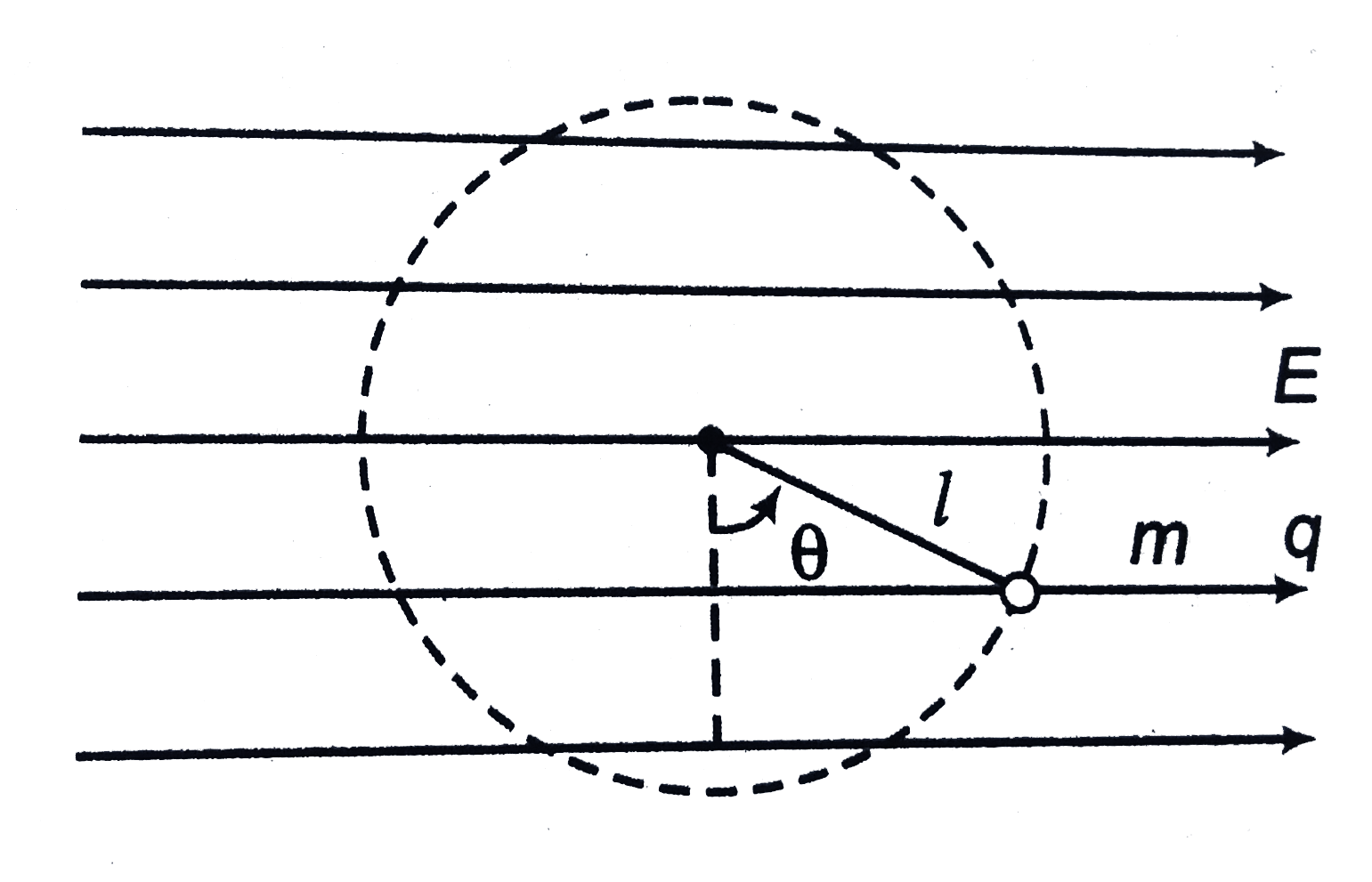 Aθ=tan−1(qEmg)Bθ=πCθ=0∘Dθ=π+tan−1(qEmg)
Aθ=tan−1(qEmg)Bθ=πCθ=0∘Dθ=π+tan−1(qEmg) - Question 2 - Select One
A pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθ - Question 3 - Select One
A positively charged particle of charge q and mass m is suspended from a point by a string of length l. In the space a uniform horizontal electric field E exists . The particle is drawn aside so that it is projected horizontally with velocity v such that the particle starts to move along a circle with same constant speed v. Find the speed v.
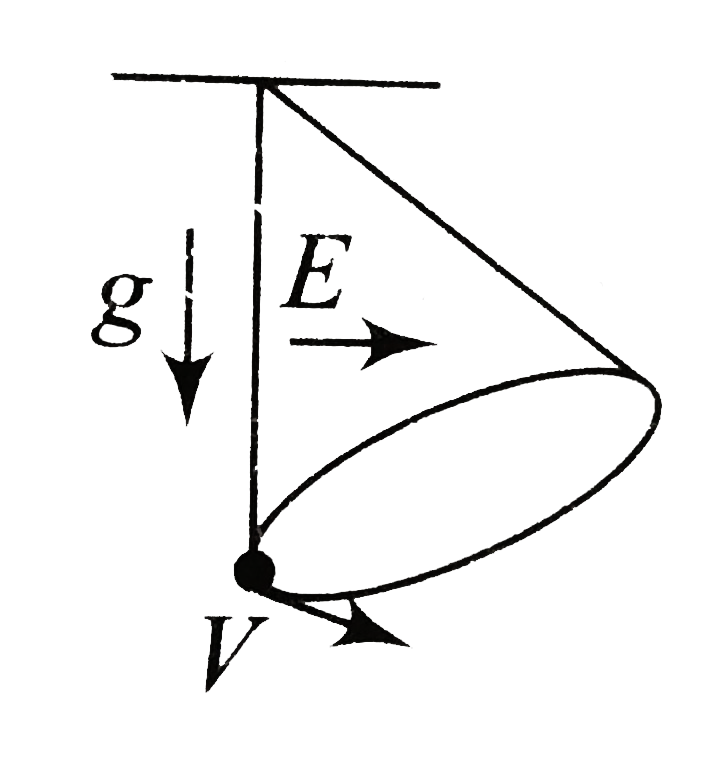 A2qEm(√lg)BqEm(√lg)CqEm(√2lg)DqE2m(√lg)
A2qEm(√lg)BqEm(√lg)CqEm(√2lg)DqE2m(√lg)
A point mass m connected to one end of inextensible string of length l and other end of string is fixed at peg. String is free to rotate in vertical plane. Find the minimum velocity given to the mass in horizontal direction so that it hits the peg in its subsequent motion.
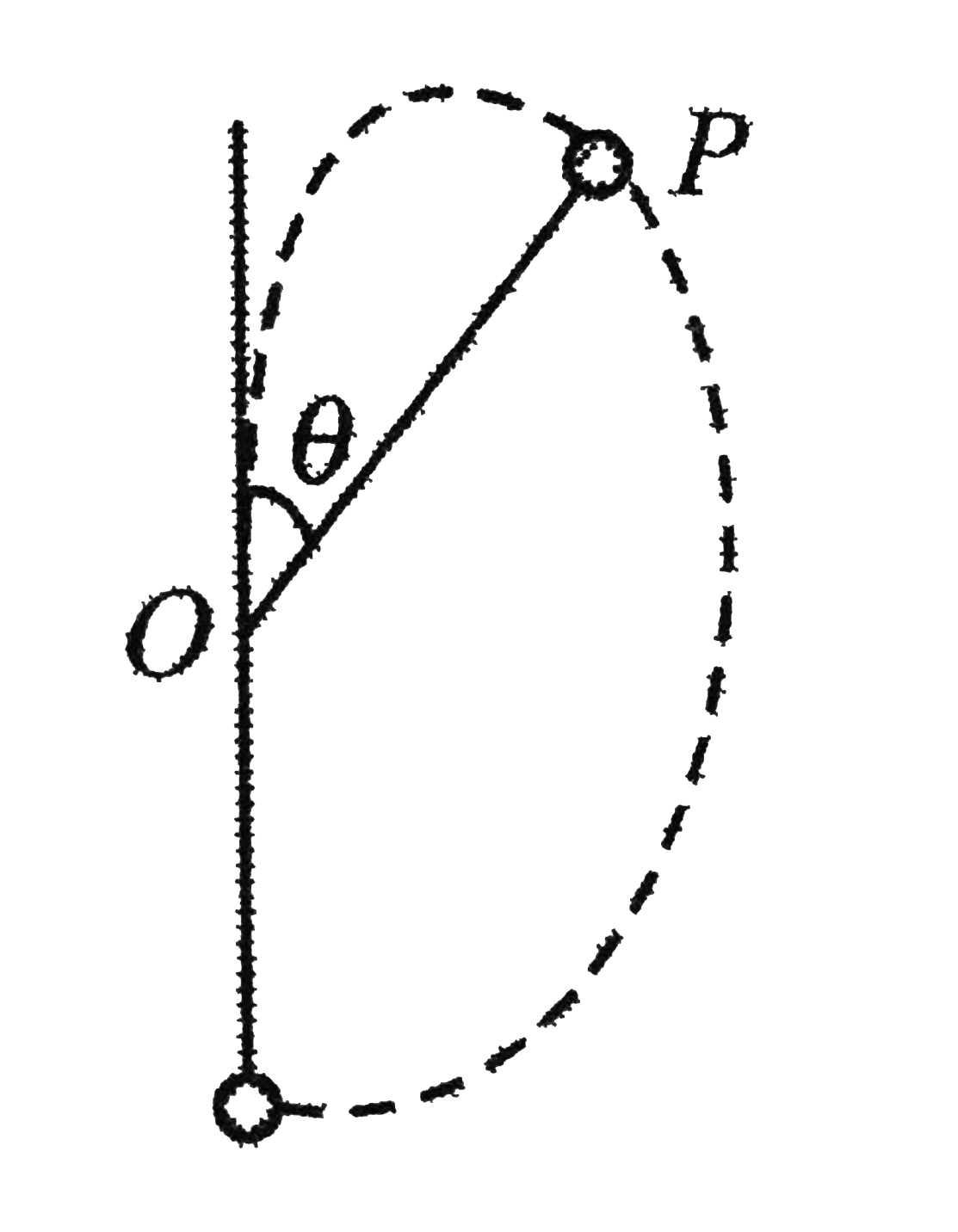 View Solution
View SolutionA ball of mass m with a charge q can rotate in a vertical plane at the end of a string of length l in a uniform electrostatic field whose lines of force are directed upwards. What horizontal velocity must be imparted to the ball in the upper position so that the tension in the string in the lower position of the ball is 1.5 times than the weight of the ball?
View SolutionA charged ball with mass m and charge q is dropped from a height h over a non-conducting smooth horizontal plane. There exists a uniform electric field E0 in vertically downward direction and the coefficient of restitution between the ball and the plane is e. Find the maximum height attained by the ball after nth collision.
View SolutionA charged cork ball of mass m is suspended on a light sting in the presence of a uniform electric field as shown in fig. When E=(Aˆi+Bˆj)NC−1, Where A and B positive numbers, the ball is in equilibirum at θ. Find (a) the charge on the ball and (b) the tension in the string.
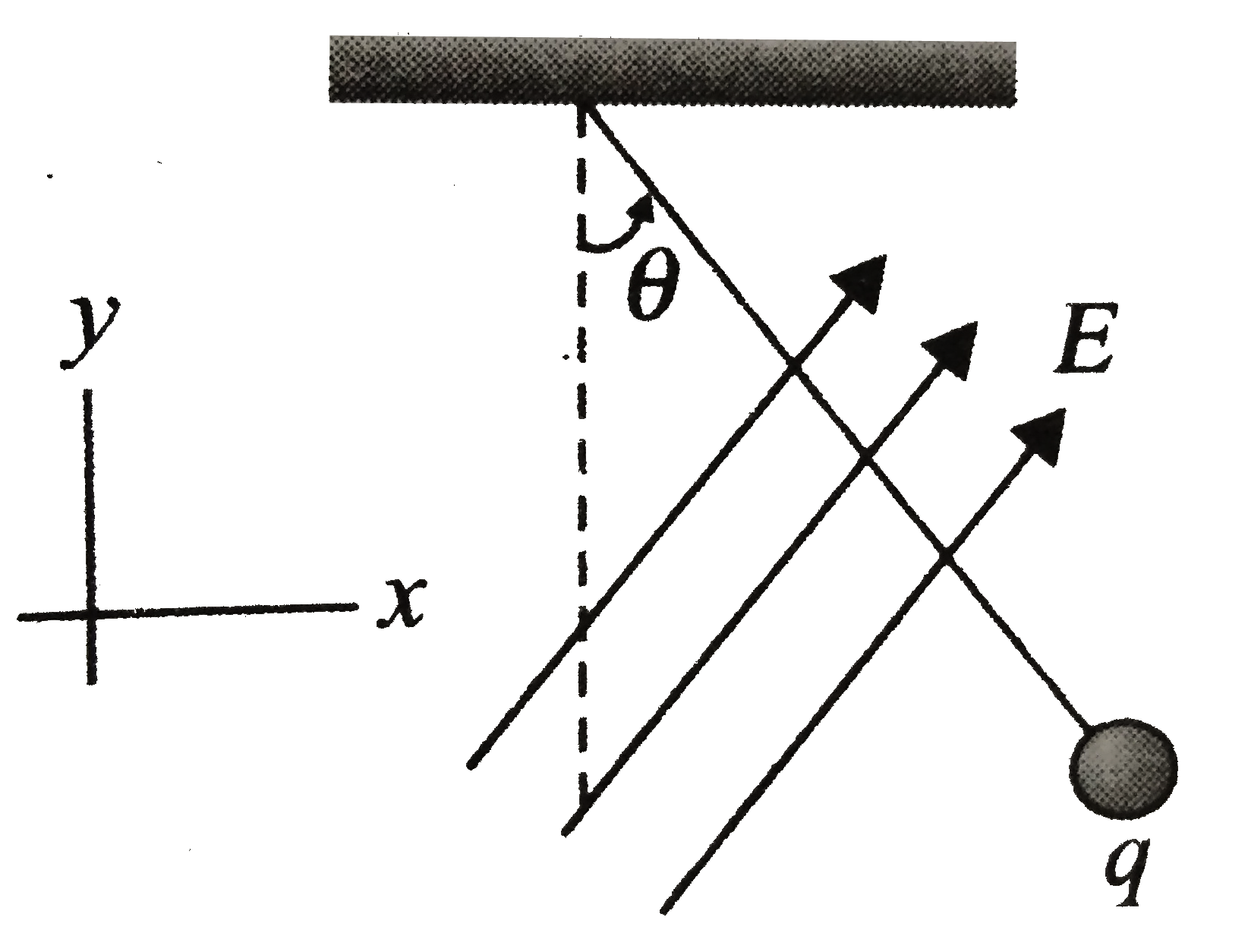 View Solution
View SolutionA mass m carrying a charge q si suspended form a string and placed in a uniform horizontal electric field of intensity E.The angel made by the string with the vertical in the equilibrium position is
View Solution
