Let $h_{A}, h_{B}, h_{C}$ be the altitudes of triangle $A B C$ from vertices $A, B, C$ respectively. With usual notations, $(\frac{1}{h_{A}}) cos A + (\frac{1}{h_{B}}) cos B + (\frac{1}{h_{C}}) cos C =$

Video Solution

Answer

Step by step video & image solution for Let h_(A),h_(B),h_(C) be the altitudes of triangle ABC from vertices A,B,C respectively. With usual notations,(1/h_(A))cos A+(1/h_(B))cos B+(1/h_(C))cosC= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Knowledge Check

Question 1 - Select One
In a $Δ A B C$ with usual notations, if $\frac{a}{cos A} = \frac{b}{cos B} = \frac{c}{cos C}$ , then the triangle is :
Aright angled
Bisosceles
Cobtuse angled
Dequilateral
Question 2 - Select One
If in a $! A B C$ , the altitudes from the vertices A , B , C on the opposite sides are in H.P., then sin A, sin B , sin C are in
AH.P.
BAGP
CA.P.
DG.P.

If in a $Δ A B C$ , the altitudes from the vertices A, B, C on opposite sides are in H.P, then sin A, sin B, sin C are in
View Solution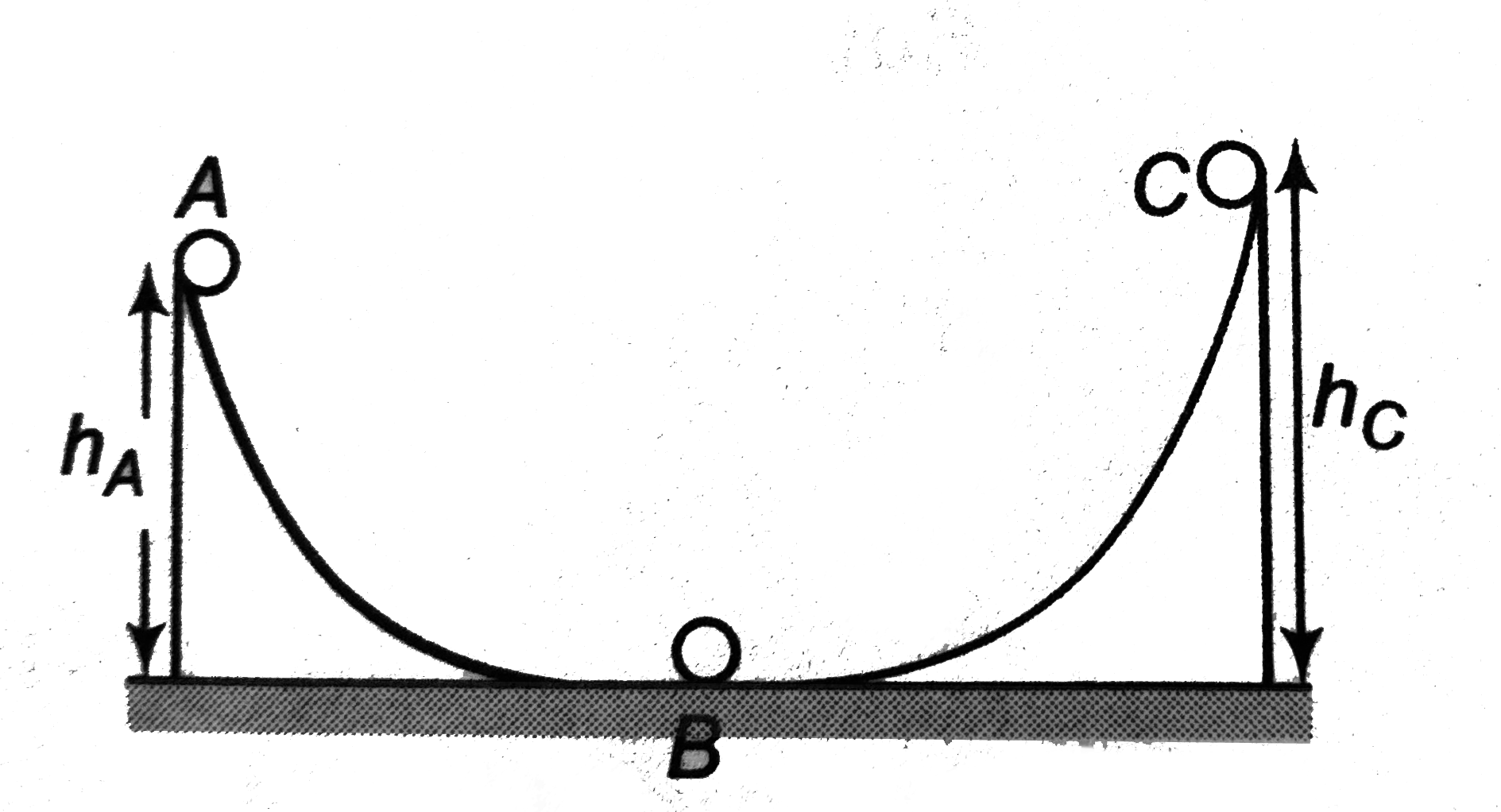
A ball moves over a fixed track as shown in thre figure. From A to B the ball rolls without slipping. If surface BC is frictionless and $K_{A}, K_{B}$ and $K_{C}$ are kinetic energies of the ball at A, B and C respectively then
(a). $h_{A} > h_{C}, K_{B} > K_{C}$
(b). $h_{A} > h_{C}, K_{C} > K_{A}$
(c). $h_{A} = h_{C}, K_{B} = K_{C}$
(d). $h_{A} < h_{C}, K_{B} > K_{C}$
View Solution
$Let A \equiv (- 2, 0), B \equiv (4, 0), C \equiv (h, h) .If perimeter of triangle A B C is minimum,then$
View Solution
Let $f, g and h$ be the lengths of the perpendiculars from the circumcenter of $Δ A B C$ on the sides a, b, and c, respectively. Prove that $\frac{a}{f} + \frac{b}{g} + \frac{c}{h} = \frac{1}{4} \frac{a b c}{f g h}$
View Solution
With usual notations, in a triangle ABC, a cos(B - C)+b cos(C-A)+ c cos(A-B) is equal to
View Solution
Let $f$ , $g$ , $h$ be the lengths of the perpendiculars from the circumcenter of the $Δ A B C$ on the sides $B C$ , $C A$ and $A B$ respectively. If $\frac{a}{f} + \frac{b}{g} + \frac{c}{h} = λ \frac{a b c}{f g h}$ , then the value of ' $λ$ ' is
(A) $\frac{1}{4}$
(B) $\frac{1}{2}$
(C) $1$
(D) $2$
View Solution

Let $h_{A}, h_{B}, h_{C}$ be the altitudes of triangle $A B C$ from vertices $A, B, C$ respectively. With usual notations, $(\frac{1}{h_{A}}) cos A + (\frac{1}{h_{B}}) cos B + (\frac{1}{h_{C}}) cos C =$

Similar Questions

Knowledge Check

In a $Δ A B C$ with usual notations, if $\frac{a}{cos A} = \frac{b}{cos B} = \frac{c}{cos C}$ , then the triangle is :

If in a $! A B C$ , the altitudes from the vertices A , B , C on the opposite sides are in H.P., then sin A, sin B , sin C are in

Recommended Questions

Let hA,hB,hC be the altitudes of triangle ABC from vertices A,B,C respectively. With usual notations,(1hA)cosA+(1hB)cosB+(1hC)cosC=

Similar Questions

Knowledge Check

In a ΔABC with usual notations, if acosA=bcosB=ccosC, then the triangle is :

If in a !ABC , the altitudes from the vertices A , B , C on the opposite sides are in H.P., then sin A, sin B , sin C are in

Recommended Questions

Let $h_{A}, h_{B}, h_{C}$ be the altitudes of triangle $A B C$ from vertices $A, B, C$ respectively. With usual notations, $(\frac{1}{h_{A}}) cos A + (\frac{1}{h_{B}}) cos B + (\frac{1}{h_{C}}) cos C =$

In a $Δ A B C$ with usual notations, if $\frac{a}{cos A} = \frac{b}{cos B} = \frac{c}{cos C}$ , then the triangle is :

If in a $! A B C$ , the altitudes from the vertices A , B , C on the opposite sides are in H.P., then sin A, sin B , sin C are in