" A charge of "10^(-10)C" is placed at the origin.The electric field at "(1,1)cm" due to it (in "NC^(-1)" ) is "
Step by step video & image solution for " A charge of "10^(-10)C" is placed at the origin.The electric field at "(1,1)cm" due to it (in "NC^(-1)" ) is " by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
The electric potential on the surface of a sphere of radius R and charge 3×10−6 C is 500 V The intensity of electric field on the surface of the sphere ( inNC−1) is
A<10B> 20CBetween 10 and 20D<5 - Question 2 - Select One
The electric potential on the surface of a sphere of radius R due to a charge 3×10−6C is 500V. The intensity of electric field on the surface of the sphere is (NC−1) is
A<10B>20Cbetween 10 and 20D<5 - Question 3 - Select One
A point dipole is located at the origin in some orientation. The electric field at the point (10 cm, 10 cm) on the x-y plane is measured to have a magnitude 1.0×10−3V/m. What will be the magnitude of the electric field at the point (20 cm, 20 cm)?
A5.0×10−4V/mB2.5×10−4V/mCIt will depend on the orientation of the dipole.D1.25×10−4V/m
A point charge of 10mC is placed at origin.The value of electric potential at point (3,4)m is.........
View SolutionA small particle of mass 1.8×10−30 kg and charge −3.5×10−19 C is dropped in a region of electric field of strength 2.8×105NC−1, directed in the upward direction. Calculate the time taken by the ball to cover a distance of 2 cm.
View SolutionA charge of 0.5 nC is placed at the centre of an equilateral triangle of side 10 cm, calculate the work done in bringing a charge of 0.1 nC from infinity to any vertices of the triangle.
View SolutionTwo point charges q1andq2, of magnitude +10–8Cand–10–8C, respectively, are placed 0.1 m apart. Calculate the electric fields at points A, B and C shown in Fig. 1.14.
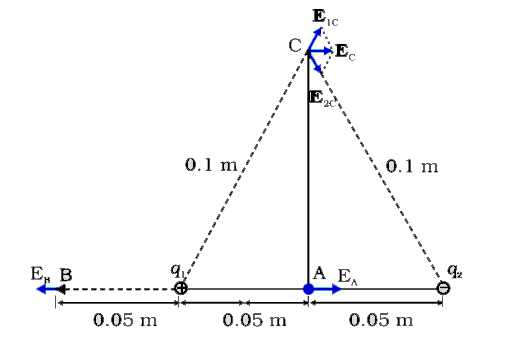 View Solution
View Solution4×1010 electrons are removed from a neutral metal sphere of diameter 20 cm placed in air. The magnitude of the electric field (in NC−1) at a distance of 20 cm from its centre is
View Solution
