A circular platform is free to rotate in a horizontal plane about a vertical axis passing through its centre. A tortoise is sitting at the edge of the platform. Now, the platform is given an angular velocity ω0 . When the tortoise moves along a chord of the platform with a constant velocity (with respect to the platform), the angular velocity of the platform will vary with time t as




The correct Answer is:B
In absence of external torque, the angular momentum of the system remains conserved.
Let the tortoise move along the chord PQ. When the tortoise moves from P to M its distance from axis point O decreases and so the moment of inertia decrease as I=mr2, where r = distance of tortoise from O. When the tortoise moves from M to Q the distance r increases and so I also increases.
IP=mR2+MR22..........(i)
IN=mr2+MR22.......(ii)

By geometry, r2=d2+[√R2−d2−vt]2........(iii)
Angular momentum is conserved
Ipω0=INω(t) or ω(t)=Ipω0IN.........(iv)
ω(t) depends on IN',IN depends on r and r depends on time t.
The function of t is non-linear.
Hence, ω(t) is a non-linear function of t.
ω increases when tortoise from P to M and ω decreases when tortoise travels from M to Q.
First increases and then decrease of ω is revealed in graph (b) and (d). But ω(t) is a non-linear function of t, hence graph (b) represents the variation of ω(t) w.r.t. time.
Similar Questions
A uniform horizontal circular platform of mass 200kg is rotating at 5 rpm about vertical axis passing through its centre. A boy of mass 80kg is standing at its edge. if boy moves to centre of platform, find out final angular speed
View SolutionA uniform horizontal circular platform of mass 200kg is rotating at 5 rpm about vertical axis passing through its centre. A boy of mass 80kg is standing at its edge. if boy moves to centre of platform, find out final angular speed
View Solution
Knowledge Check
A circular platform is free to rotate in a horizontal plane about a vertical axis passing through its centre. A tortoise is sitting at the edge of the platform. Now the platform is given an angular velocity ω0. When the tortoise move along a chord of the platform with a constant velocity (with respect to the platform),
A B
B C
C D
D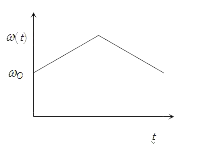
A circular plarform is free to rotate in a horizontal plane about a vertical axis passing through its centre. A tortoise is sitting at the edge of the platform. Now, the platform is given an angular velocity ω0. When the tortoise move along a chord of the platform with a constant velocity (with respect to the platform), the angular velocity of the platform ω(t) will vary with time t as
A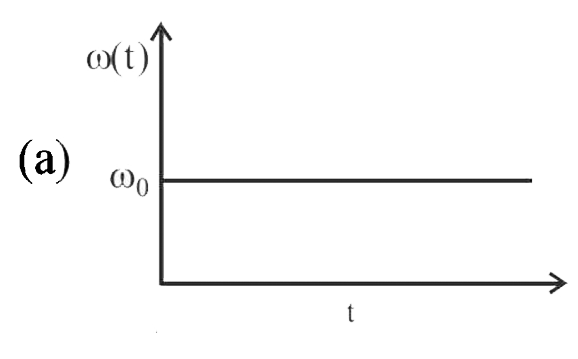 B
B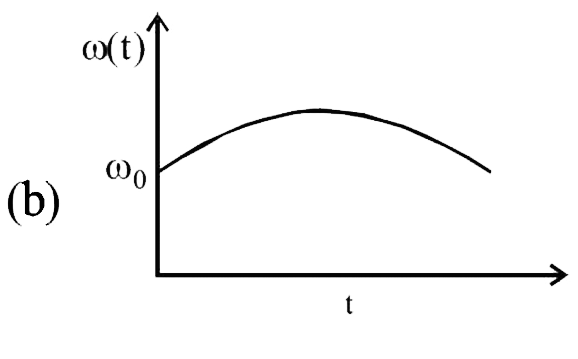 C
C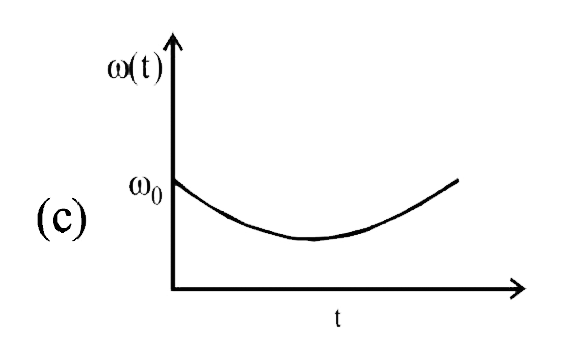 D
D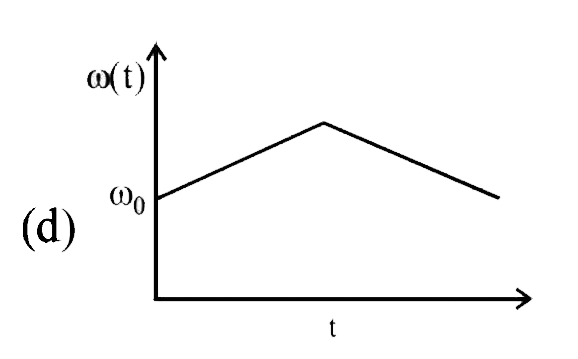
A circular platform is free to rotate in a horizontal plane about a vertical axis passing through its center. A tortoise is sitting at the edge of the platform. Now, the platform is given an angular velocity c.o0. When the tortoise moves along the diameter of the platform with a constant velocity (with respect to the platform), the angular velocity of the platform ω(t) will vary with time t as
A B
B C
C D
D
A person of mass m stands at the edge of a circular platform of radius R and moment of inertia. A platform is at rest initially. But the platform rotate when the person jumps off from the platform tangentially with velocity u with respect to platfrom. Determine the angular velocity of the platform.
View SolutionA horizontal platform with a mass of 100kg rotates at 10(r±) around a vertical axis passing through its centre. A man of mass 60kg is standing on its edge. The platform begins to rotaté with an angular velocity ω(r±), if the man "moves from the edge of the platform to its centre. Regard the platform as a circular homogeneous disk and the man as a point mass. Find the value of 3ω11.
View SolutionA man of mass m1 is standing on a platform of mass m2 kept on a smooth horizontal surface. The man starts moving on the platform with a velocity vr relative to the platform. Find the recoil velocity of platform.
View SolutionConsider the situation of previous example. If the platform is rotating intially with angular velocity ω0 and then person jumps off tangentially. Determine the new angular velocity of the platform.
View SolutionA uniform horizontal circular platform of mass 200kg is rotating at 10 rpm about a vertical axis passing through its center. A boy of mass 50kg is standing at its edge. If the boy moves to the center of the platform, the frequency of rotation would become
View Solution
