For a reaction of reversible nature, net rate is (dxdt)=k1[A][B]−k2[C] hence, given reaction is :
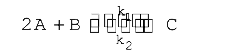
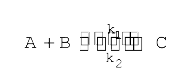
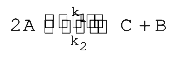
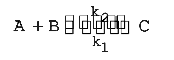
The correct Answer is:B
rf=k1[A][B]⇒rb=k2[C] Net rate =k1[A][B]−k2[C]
Similar Questions
Knowledge Check
For a reaction of reversible nature , net rate is (dxdt)=k1[A]2[B]1−k2[C], hence , given reactionis:
A2A+1B⇔CB2A+B⇔CC2A⇔C+B−1Dnone of theseFor a reaction,net rate is (dxdt)=k[A]2−k'[C][B]2 then ,Select the correct Statement:
A−d[A]2dt=d[b]2dt=d[C]dt is the relation amongB2A⇔2B+C is the required reactionCBoth are correctDnone is correctRate expression of a chemical change is −dxdt=k[A]2[B]1[C]0 The order of reaction is :
A3B2C1Dzero
The instantaneous rate of an elementary chemical reaction
aA+bB(⇔)cC+dD can be given by:
rate =kf[A]a[B]b−kb[C]c[D]d
where kfandkb are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]a[B]b where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is ab times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae−(Ea/RT) whereas the ratio kfkb is expressed in terms of van't Hoff isochore: KfKb=Ae−ΔH/RT where Ea and ΔH are energy of activation and enthalpy of reaction respectively
For a chemical reaction :aA→bB<br>log[(d[A])/(dt)]=log [(d[B])/(dt)+0.3
Then the ratio of a and b is approximately:
View SolutionThe instantaneous rate of an elementary chemical reaction
aA+bB(⇔)cC+dD can be given by:
rate =kf[A]a[B]b−kb[C]c[D]d
where kfandkb are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]a[B]b where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is ab times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae−(Ea/RT) whereas the ratio kfkb is expressed in terms of van't Hoff isochore: KfKb=Ae−ΔH/RT where Ea and ΔH are energy of activation and enthalpy of reaction respectively
For a gaseous phase Ist order reaction
A(g)→B(g)+2C(g)
(rate constant K=10−2time−1)
in a closed vessel of 2 litre containing 5 mole of A(g) at 27∘ C which of the following is incorrect?
View SolutionThe instantaneous rate of an elementary chemical reaction
aA+bB(⇔)cC+dD can be given by:
rate =kf[A]a[B]b−kb[C]c[D]d
where kfandkb are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]a[B]b where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is ab times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae−(Ea/RT) whereas the ratio kfkb is expressed in terms of van't Hoff isochore: KfKb=Ae−ΔH/RT where Ea and ΔH are energy of activation and enthalpy of reaction respectively
For an elementary reaction aA→ product the graph plotted between log [−d[A]dt] vs. concentration gives a straight line with intercept equal to 0.6 and showing an angle of 45∘ with origin, then:
View SolutionThe rate law expression for a hypothetic reaction:
2A+3B→2C is
dxdt=k[A][B]2
The order of the reaction is
View SolutionFor a reaction, A+2B→C, rate is given by +d[C]dt=k[A][B], hence, the order of the reaction is
View Solution