Question

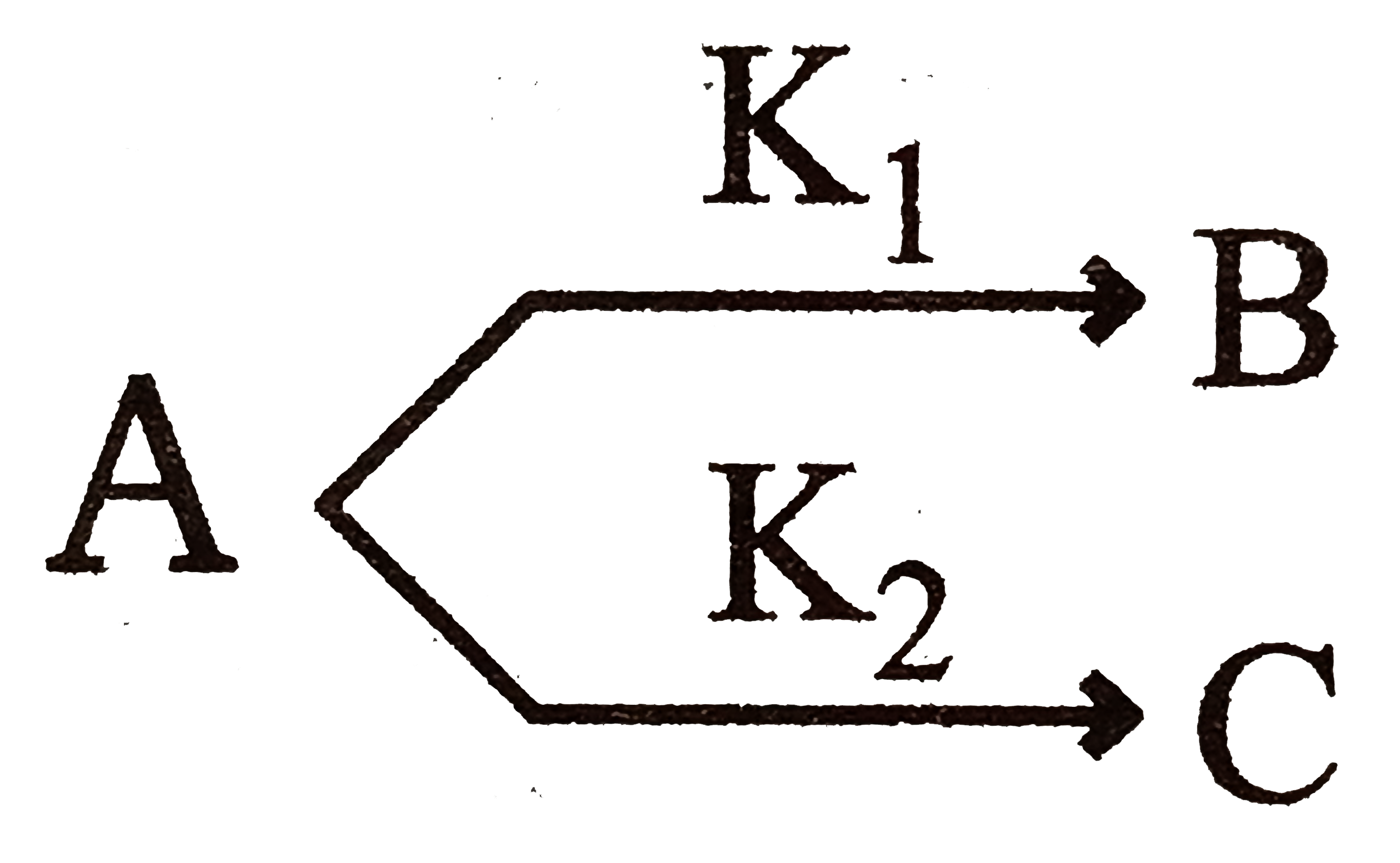
For the first order reaction,

Options

A. the degree of dissociation is equal to `(1-e^(-kt))`
B. a plot of reciprocal concentration of the reactant vs time gives a straight line
C. the time taken for the completion of 75% reaction is thrice the `(1)/(2)` of the reaction
D. the pre-exponential factor in the Arrhenius equation has dimension of time, `T^(-1)`

Answer

A::D

Explanation

To solve the question regarding a first-order reaction, we will break down the steps to find the degree of dissociation (α) and analyze the options provided.

1. Understanding Degree of Dissociation (α):
- For a reaction, the degree of dissociation (α) is defined as the fraction of the initial amount of reactant that has dissociated. If we start with 1 mole of A, and x moles dissociate, then:
\( \alpha = \frac{x}{1} = x \)

2. Relating α to Concentrations:
- If we denote the initial concentration of A as \( C_0 \) and the concentration at time t as \( C_t \), then:
\( C_t = C_0 e^{-kt} \)
- The amount dissociated (x) can be expressed as:
\( x = C_0 - C_t \)
- Substituting for \( C_t \):
\( x = C_0 - C_0 e^{-kt} = C_0(1 - e^{-kt}) \)

3. Finding α:
- Now, substituting x into the expression for α:
\( \alpha = \frac{x}{C_0} = \frac{C_0(1 - e^{-kt})}{C_0} = 1 - e^{-kt} \)
- Thus, the degree of dissociation for a first-order reaction is:
\( \alpha = 1 - e^{-kt} \)

4. Analyzing the Options:
- Option 1: The expression \( \alpha = 1 - e^{-kt} \) is correct.
- Option 2: A plot of reciprocal concentration (1/C) versus time does not yield a straight line for a first-order reaction, so this option is incorrect.
- Option 3: The time taken for 75% completion is not thrice the half-life; it is actually twice the half-life. Therefore, this option is incorrect.
- Option 4: The pre-exponential factor (A) in the Arrhenius equation has the same dimensions as the rate constant (k) for a first-order reaction, which is time inverse (s⁻¹). This option is correct.

5. Final Conclusion:
- The correct answers are Options 1 and 4.