Draw all the possible stereoisomers of 3-pentene - 2-ol.
The correct Answer is:(##MOTCONJ∃CHEC21E03006_A01##)
To find all possible stereoisomers of 3-pentene-2-ol, we will follow these steps:
Step 1: Determine the Structure of 3-Pentene-2-ol
3-Pentene-2-ol has the following structure:
- The main chain is a pentane (5 carbon atoms).
- There is a double bond between the 3rd and 4th carbon atoms (3-pentene).
- There is an alcohol (-OH) group on the 2nd carbon atom.
The structural formula can be represented as:
CH3−CH(OH)−CH=CH−CH3
Step 2: Identify Chiral Centers
A chiral center is a carbon atom that has four different groups attached to it. In 3-pentene-2-ol, the carbon atom at position 2 (C2) is a chiral center because it has:
- Hydroxyl group (-OH)
- Methyl group (CH3)
- Hydrogen (H)
- A carbon chain (C3)
Step 3: Determine Geometric Isomers
Since there is a double bond between C3 and C4, we can have geometric (cis-trans) isomers. The two possible configurations for the double bond are:
- Cis Isomer: The higher priority groups (C2 -OH and C4 -CH3) are on the same side of the double bond.
- Trans Isomer: The higher priority groups are on opposite sides of the double bond.
Step 4: Draw the Geometric Isomers
1. Cis-3-pentene-2-ol:
CH3|CH3−CH(OH)−C=C|H
2. Trans-3-pentene-2-ol:
CH3|CH3−CH(OH)−C=C|H
Step 5: Draw the Optical Isomers
For each geometric isomer, we can draw the optical isomers by switching the positions of the groups around the chiral center (C2).
1. Cis-3-pentene-2-ol Optical Isomers:
- R-configuration:
CH3|CH3−CH(OH)−C=C|H
- S-configuration:
CH3|CH3−CH(OH)−C=C|H
2. Trans-3-pentene-2-ol Optical Isomers:
- R-configuration:
CH3|CH3−CH(OH)−C=C|H
- S-configuration:
CH3|CH3−CH(OH)−C=C|H
Final Summary
In total, we have:
- 2 geometric isomers (cis and trans)
- Each geometric isomer has 2 optical isomers (R and S)
Thus, the total number of stereoisomers for 3-pentene-2-ol is 4.
3-Pentene-2-ol has the following structure:
- The main chain is a pentane (5 carbon atoms).
- There is a double bond between the 3rd and 4th carbon atoms (3-pentene).
- There is an alcohol (-OH) group on the 2nd carbon atom.
CH3−CH(OH)−CH=CH−CH3
A chiral center is a carbon atom that has four different groups attached to it. In 3-pentene-2-ol, the carbon atom at position 2 (C2) is a chiral center because it has:
- Hydroxyl group (-OH)
- Methyl group (CH3)
- Hydrogen (H)
- A carbon chain (C3)
Since there is a double bond between C3 and C4, we can have geometric (cis-trans) isomers. The two possible configurations for the double bond are:
- Cis Isomer: The higher priority groups (C2 -OH and C4 -CH3) are on the same side of the double bond.
- Trans Isomer: The higher priority groups are on opposite sides of the double bond.
1. Cis-3-pentene-2-ol:
CH3|CH3−CH(OH)−C=C|H
CH3|CH3−CH(OH)−C=C|H
For each geometric isomer, we can draw the optical isomers by switching the positions of the groups around the chiral center (C2).
- R-configuration:
CH3|CH3−CH(OH)−C=C|H
- S-configuration:
CH3|CH3−CH(OH)−C=C|H
- R-configuration:
CH3|CH3−CH(OH)−C=C|H
- S-configuration:
CH3|CH3−CH(OH)−C=C|H
In total, we have:
- 2 geometric isomers (cis and trans)
- Each geometric isomer has 2 optical isomers (R and S)
Topper's Solved these Questions
- ISOMERISMBOOK - MOTIONCHAPTER - ISOMERISMEXERCISE - Exercise 4
26 Videos
- ISOMERISMBOOK - MOTIONCHAPTER - ISOMERISMEXERCISE - Exercise 2
50 Videos
- IONIC EQUILIBRUIMBOOK - MOTIONCHAPTER - IONIC EQUILIBRUIMEXERCISE - Exercise -4 (Level -II)
14 Videos
- LIQUID SOLUTIONBOOK - MOTIONCHAPTER - LIQUID SOLUTIONEXERCISE - EXERCISE-4 (Level-II) PREVIOUS YEAR JEE ADVANCED
19 Videos
Similar Questions
Knowledge Check
Two possible stereoisomers for
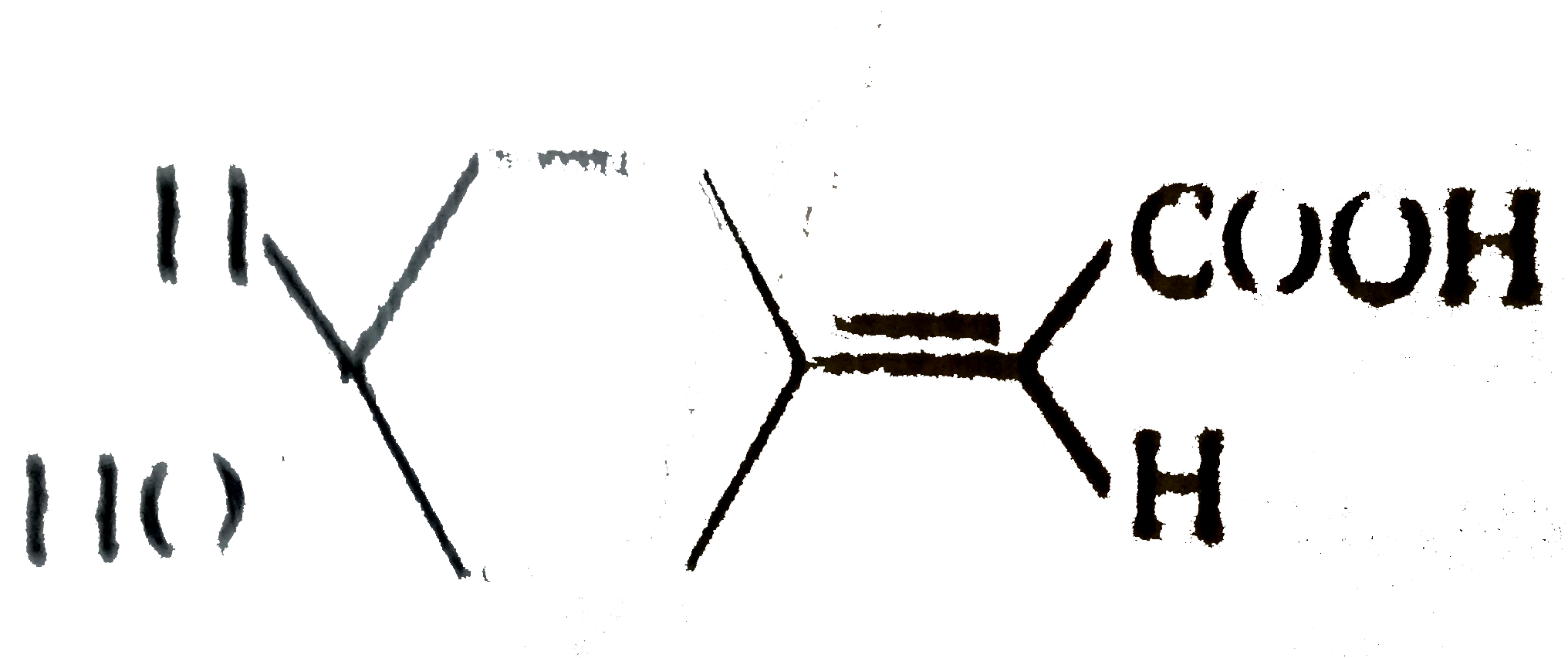 AenantiomersBdiastereomersCconformersDrotamers
AenantiomersBdiastereomersCconformersDrotamersTwo possible stereoisomers for
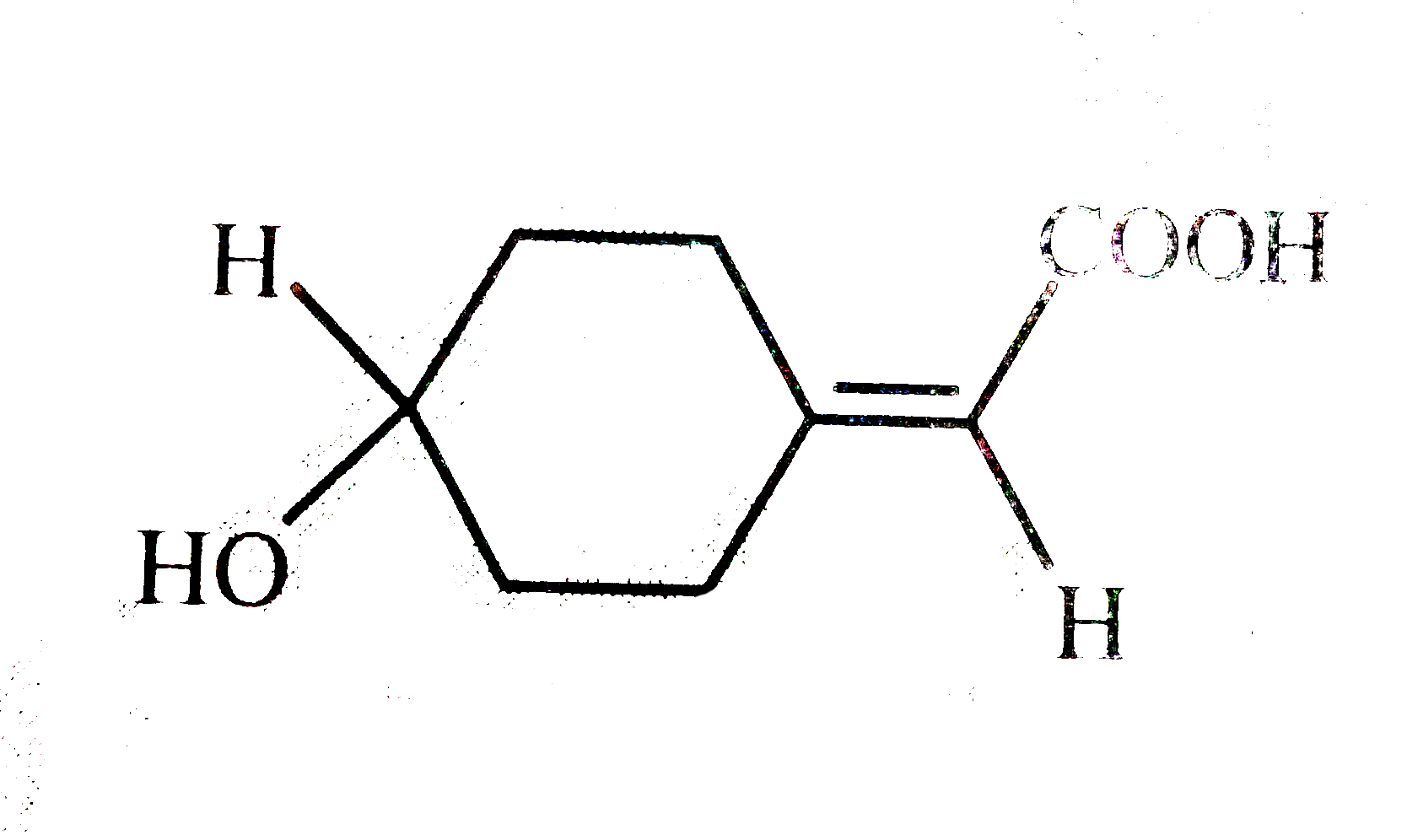
areAenantiomersBdiasteromersCconformersDrotamersThe total possible stereoisomers of 1-chloro-3-methyl pentane is :
A2B3C4D5
Draw the structures of all possible isomers of pentane.
View Solution1-penten-3-one
View SolutionWrite all possible chain isomers for pentene and hexyne .
View SolutionDraw all the possible lines of symmetry of an equilateral triangle.
View SolutionThe number of theoretically possible stereoisomers in the following steroid is
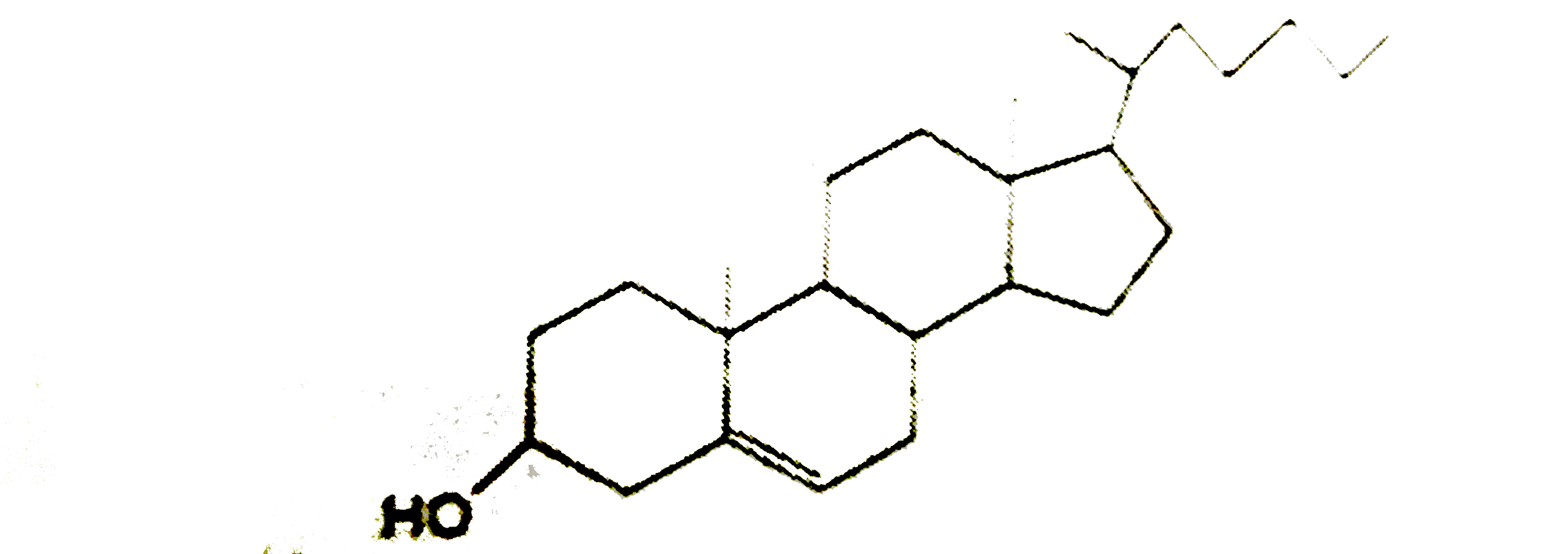
View Solution
