A glass bulb of volume 100 cm3 is connected by a narrow tube of negligible volume to another bulb of volume 300cm3. The apparatus is filled with air at a pressure of 76 cm of mercury and a temperatureof 12∘C, andthensealed. The smaller bulb is then immersedin melting ice whilst the larger bulb is placed in boiling water.Calculate the fraction of the total mass of air in the larger bulb, ignoring the expansion of the bulbs.
To solve the problem, we will follow these steps:
Step 1: Understand the System
We have two bulbs:
- Bulb 1 (smaller): Volume V1=100cm3
- Bulb 2 (larger): Volume V2=300cm3
The initial conditions are:
- Pressure Pi=76cmHg
- Temperature Ti=12∘C=285K
Step 2: Final Conditions
- Bulb 1 (smaller) is immersed in melting ice: Tf1=0∘C=273K
- Bulb 2 (larger) is placed in boiling water: Tf2=100∘C=373K
Step 3: Calculate Initial Moles of Air in Each Bulb
Using the ideal gas law, the number of moles n can be calculated using the formula:
n=PVRT
For Bulb 1:
ni1=Pi⋅V1R⋅Ti=76⋅100R⋅285
For Bulb 2:
ni2=Pi⋅V2R⋅Ti=76⋅300R⋅285
Step 4: Total Initial Moles
The total initial moles ni is:
ni=ni1+ni2=76⋅100R⋅285+76⋅300R⋅285
Step 5: Final Moles in Each Bulb
At equilibrium, the final number of moles in each bulb can be expressed as:
For Bulb 1:
nf1=P⋅V1R⋅Tf1=P⋅100R⋅273
For Bulb 2:
nf2=P⋅V2R⋅Tf2=P⋅300R⋅373
Step 6: Conservation of Moles
Since the total number of moles is conserved:
ni1+ni2=nf1+nf2
Substituting the expressions from Steps 3 and 5:
76⋅100R⋅285+76⋅300R⋅285=P⋅100R⋅273+P⋅300R⋅373
Step 7: Simplifying the Equation
Cancelling R from both sides and simplifying:
76⋅400285=P⋅100273+P⋅300373
Step 8: Solve for P
Rearranging gives:
P(100273+300373)=76⋅400285
Step 9: Calculate the Fraction of Mass in Bulb 2
The fraction of the total mass of air in the larger bulb is given by:
Fraction=nf2ni1+ni2=P⋅300R⋅37376⋅400285
Step 10: Substitute and Calculate
Substituting the value of P obtained from Step 8 into the fraction formula will yield the final result.
Final Calculation
After performing the calculations, we find that the fraction of the total mass of air in the larger bulb is approximately 0.23.
We have two bulbs:
- Bulb 1 (smaller): Volume V1=100cm3
- Bulb 2 (larger): Volume V2=300cm3
- Pressure Pi=76cmHg
- Temperature Ti=12∘C=285K
- Bulb 1 (smaller) is immersed in melting ice: Tf1=0∘C=273K
- Bulb 2 (larger) is placed in boiling water: Tf2=100∘C=373K
Using the ideal gas law, the number of moles n can be calculated using the formula:
n=PVRT
ni1=Pi⋅V1R⋅Ti=76⋅100R⋅285
ni2=Pi⋅V2R⋅Ti=76⋅300R⋅285
The total initial moles ni is:
ni=ni1+ni2=76⋅100R⋅285+76⋅300R⋅285
At equilibrium, the final number of moles in each bulb can be expressed as:
For Bulb 1:
nf1=P⋅V1R⋅Tf1=P⋅100R⋅273
nf2=P⋅V2R⋅Tf2=P⋅300R⋅373
Since the total number of moles is conserved:
ni1+ni2=nf1+nf2
76⋅100R⋅285+76⋅300R⋅285=P⋅100R⋅273+P⋅300R⋅373
Cancelling R from both sides and simplifying:
76⋅400285=P⋅100273+P⋅300373
Rearranging gives:
P(100273+300373)=76⋅400285
The fraction of the total mass of air in the larger bulb is given by:
Fraction=nf2ni1+ni2=P⋅300R⋅37376⋅400285
Substituting the value of P obtained from Step 8 into the fraction formula will yield the final result.
After performing the calculations, we find that the fraction of the total mass of air in the larger bulb is approximately 0.23.
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - Kinetic Theory of Gases and Gas LawsEXERCISE - Advance MCQs with One or More Options Correct15videos
Kinetic Theory of Gases and Gas Laws
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO66videos
HEAT TRANSFER
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - U.N.P79videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
Similar Questions
Two glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0∘C and a pressure of 76cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62∘C. What is the new value of the pressure inside the bulbs? The volume of the connecting tube is negligible.
View Solutiontwo glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0(∘)C at a pressure of 76cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62∘C.What is the new value of the pressure inside the bulbs? The volume of the connecting tube is neigligible.
View Solution
Knowledge Check
- Question 1 - Select One
A glass bulb of volume 400cm3 is connected to another bulb of volume 200cm3 by means of a tube of negligible volume. The bulbs contain dry air and are both at a common temperature and pressure of 20∘C and 1.000 atm, respectively. The larger bulb is immersed in steam at 100∘C and the smaller in melting ice at 0∘. Find the final common pressure.
A1.13atmB11.3atmC113atmDNone of these - Question 2 - Select One
Two bulbs of volumes 200 cm3 and 100 cm3 are connected by a short tube containing an insulating porous plug that permits equalization of pressure but not of temperature between the bulbs. The system is sealed at 77∘C when it contains oxygen under a pressure of 1 bar. The small bulb is immersed at 27∘C and the large bulb is placed at 127∘C. Neglecting thermal expansion of the bulbs, select the correct options.
AFinal pressure inside the system would be greater than 1 barBFinal pressure inside the system would be less than 1 barCNumber of moles of O2 would increase in small container compared to initialDNumber of moles of O2 would increase in big container compared to initial - Question 3 - Select One
Two identical glass bulbs are interconnected by a thin glass tube. A gas is filled in these bulbs at N.T.P. If one bulb is placed in ice and another bulb is placed in hot bath, then the pressure of the gas becomes 1.5 times. The temperature of hot bath will be
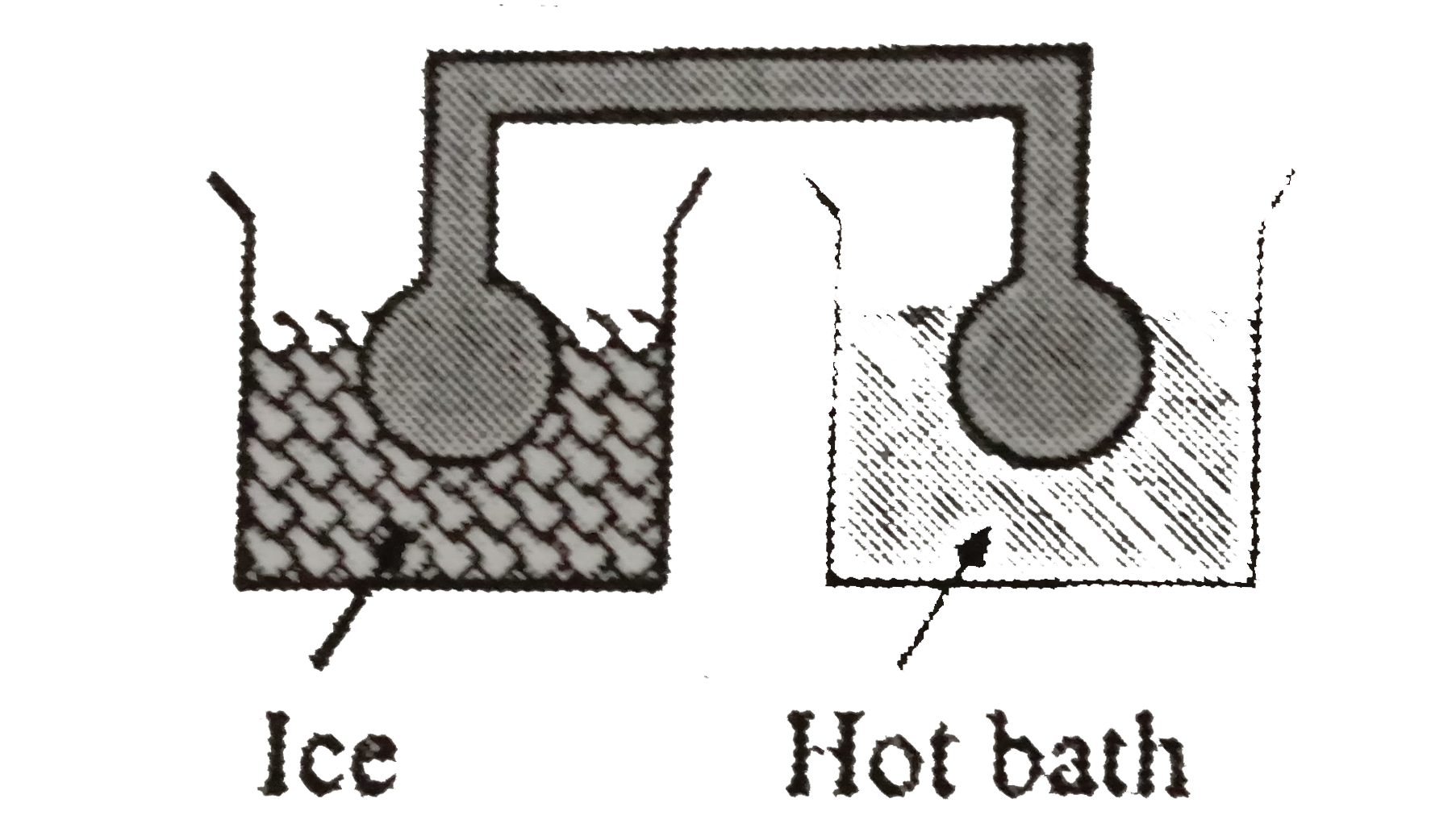 A100∘CB182∘CC256∘CD546∘C
A100∘CB182∘CC256∘CD546∘C
A bulb contains air at atmospheric pressure at 40∘C. The maximum pressure bulb can with stand is 2 atmosphere. Calculate the temperature of air when the bulb is on the point of bursting.
View SolutionTwo flacks of equal volume connected by a narrow tube of negligible volume are filled with N2 gas. When both are immersed in boiling water, the gas pressure inside the system is 0.5atm. Calculate the pressure of the system when one of the flasks is immersed in an ice-water mixture keeping the other in boiling order.
View SolutionA 100 W bulb is emmiting light of wavelength 300 nm .Calculate the number of photon emitted by the bulb in 1 min?
View SolutionAn electric bulb is connected to a 220V power supply line. If the bulb draws a current of 0.5A, calculate the power of the bulb.
View SolutionThe pressure of the gas in a constant volume gas thermometer is 80cm of mercury in melting ice at 1atm. When the bulb is placed in a liquid, the pressure becomes 160cm of mercury. Find the temperature of the liquid.
View Solution

