Define collinear vectors.Solution in Kannada
Step by step video & image solution for Define collinear vectors. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ANNUAL EXAMINATION QUESTION PAPER MARCH 2017BOOK - SUBHASH PUBLICATIONCHAPTER - ANNUAL EXAMINATION QUESTION PAPER MARCH 2017EXERCISE - PART-B
14 Videos
- ANNUAL EXAMINATION QUESTION PAPER MARCH 2017BOOK - SUBHASH PUBLICATIONCHAPTER - ANNUAL EXAMINATION QUESTION PAPER MARCH 2017EXERCISE - PART-C
15 Videos
- ANNUAL EXAMINATION QUESTION PAPER MAR-2019BOOK - SUBHASH PUBLICATIONCHAPTER - ANNUAL EXAMINATION QUESTION PAPER MAR-2019EXERCISE - PART E
4 Videos
- APPLICATION OF DERIVATIVES BOOK - SUBHASH PUBLICATIONCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - TRY YOURSELF
13 Videos
Similar Questions
If f:R→R,defined by F(x)=1+x2, then show that f is neither 1 - 1 nor onto.
View SolutionShow that the relation R in R (set of real numbers) is defined as R={(a,b),a≤b}is reflexive and transitive but not symmetric.
View SolutionProve that ∫a0f(x)dx=∫a0f(a−x)dx and hence evaluate π2∫0cos5xsin5+x+cos5xdx.
View SolutionFind the rate of change of the area of a circle with respect to its radius r when r = 5cm.
View SolutionAnswer the following as true or false.
(i) →aand −→aare collinear.
(ii) Two collinear vectors are always equal in magnitude.
(iii) Two vectors having same magnitude are collinear.
(iv) Two collinear vectors having the same magnitude
View SolutionIn Fig. ABCD is a regular hexagon, which vectors are: Collinear Equal Coinitial Collinear but not equal
View SolutionIn a regular hexagon find which vectors are collinear, equal, coinitial, collinear but not equal.
View SolutionIn the given figure, vectors which are
Collinear
Equal
Collinear but not equal.
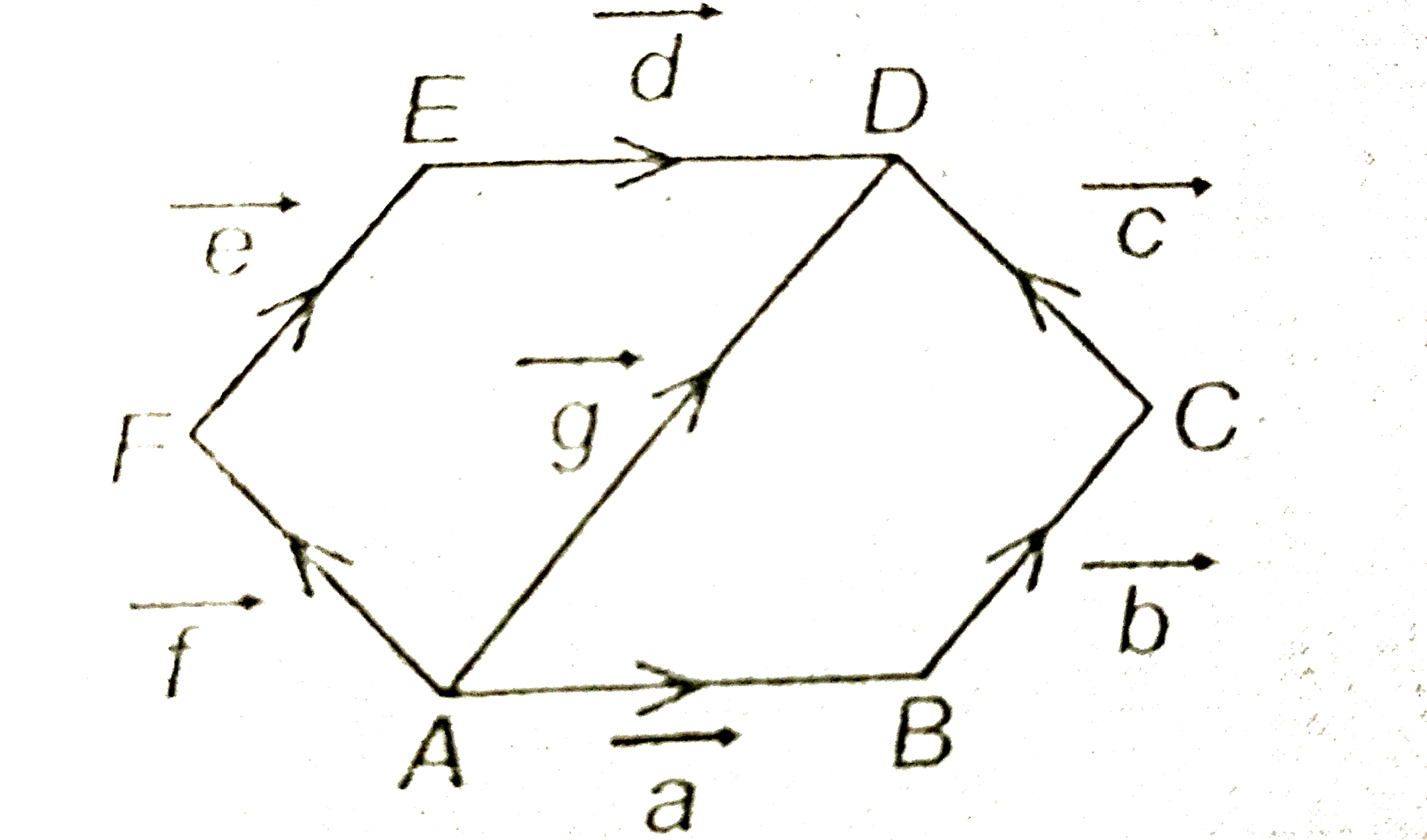
View SolutionIf ˆaandˆb are unit vectors then the vector defined as →V=(ˆa׈b)×(ˆa+ˆb) is collinear to the vector :
View SolutionDefine Coinitial vector and Collinear vector .
View SolutionLet a, b and c be three non zero vectors, no two of which are collinear. If the vector a + 2b is collinear with c, and b + 3c is collinear with a, then a + 2b + 6c =
View SolutionWhat is meant by Collinear vector ? Explain them .
View SolutionDefine : (i) collinear vectors and (ii) coplanar vectors.
View SolutionAnswer the following as true or false.(i) →aand −→aare collinear.(ii) Two collinear vectors are always equal in magnitude.(iii) Two vectors having same magnitude are collinear.(iv) Two collinear vectors having the same magni
View Solutioncollinear vectors|coplanar vectors
View SolutionAny two collinear vectors are
View Solution
