Question

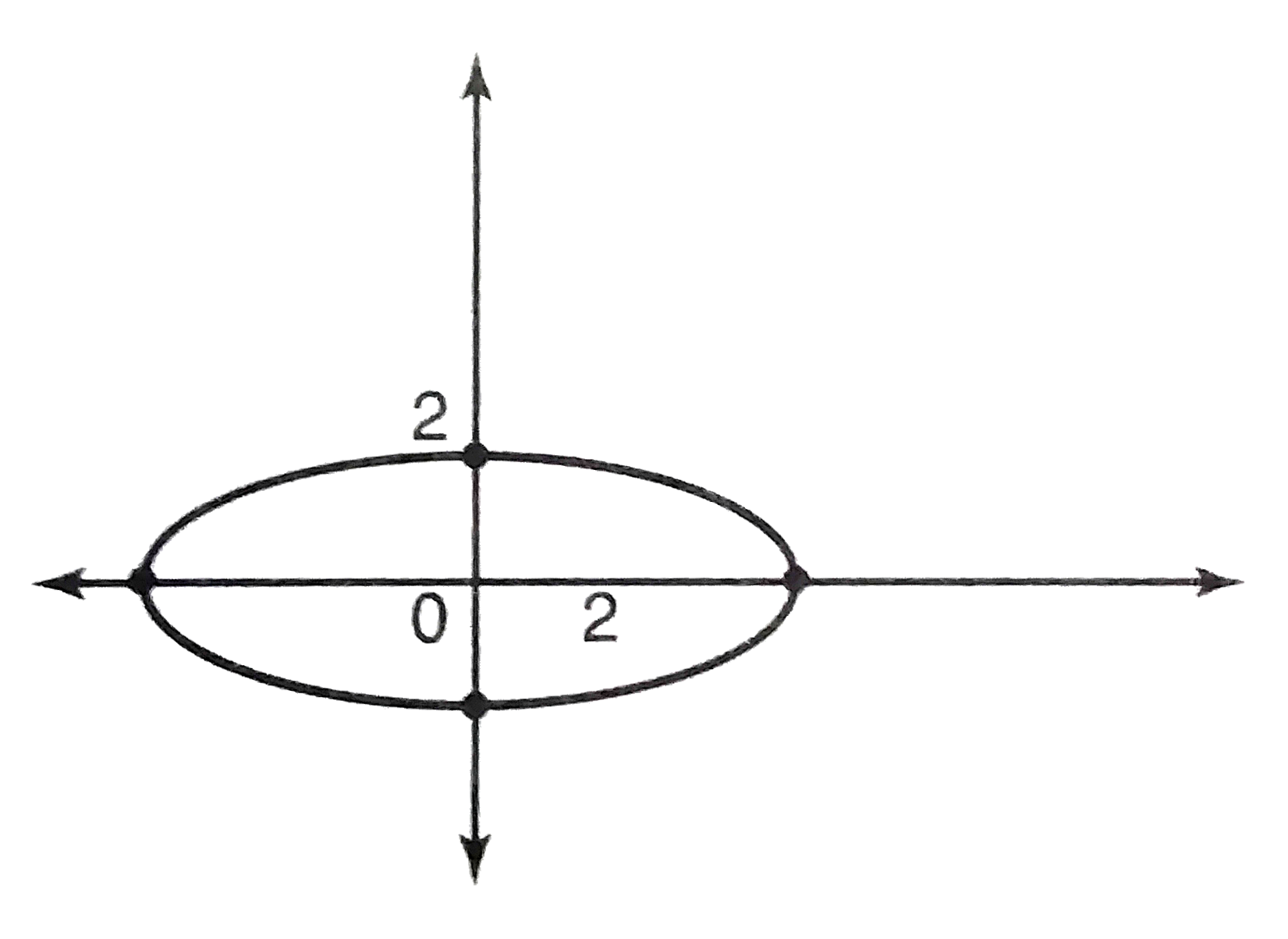
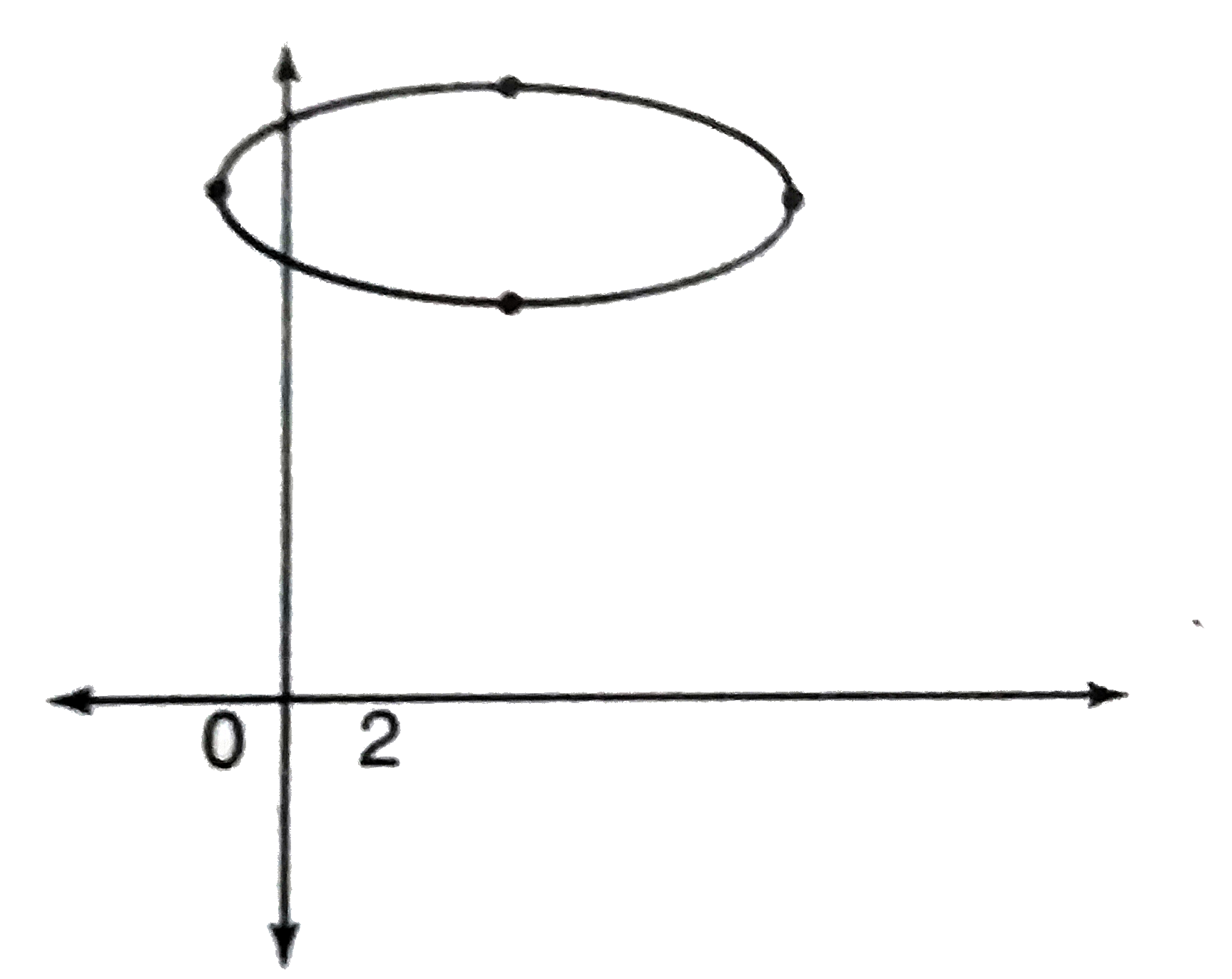
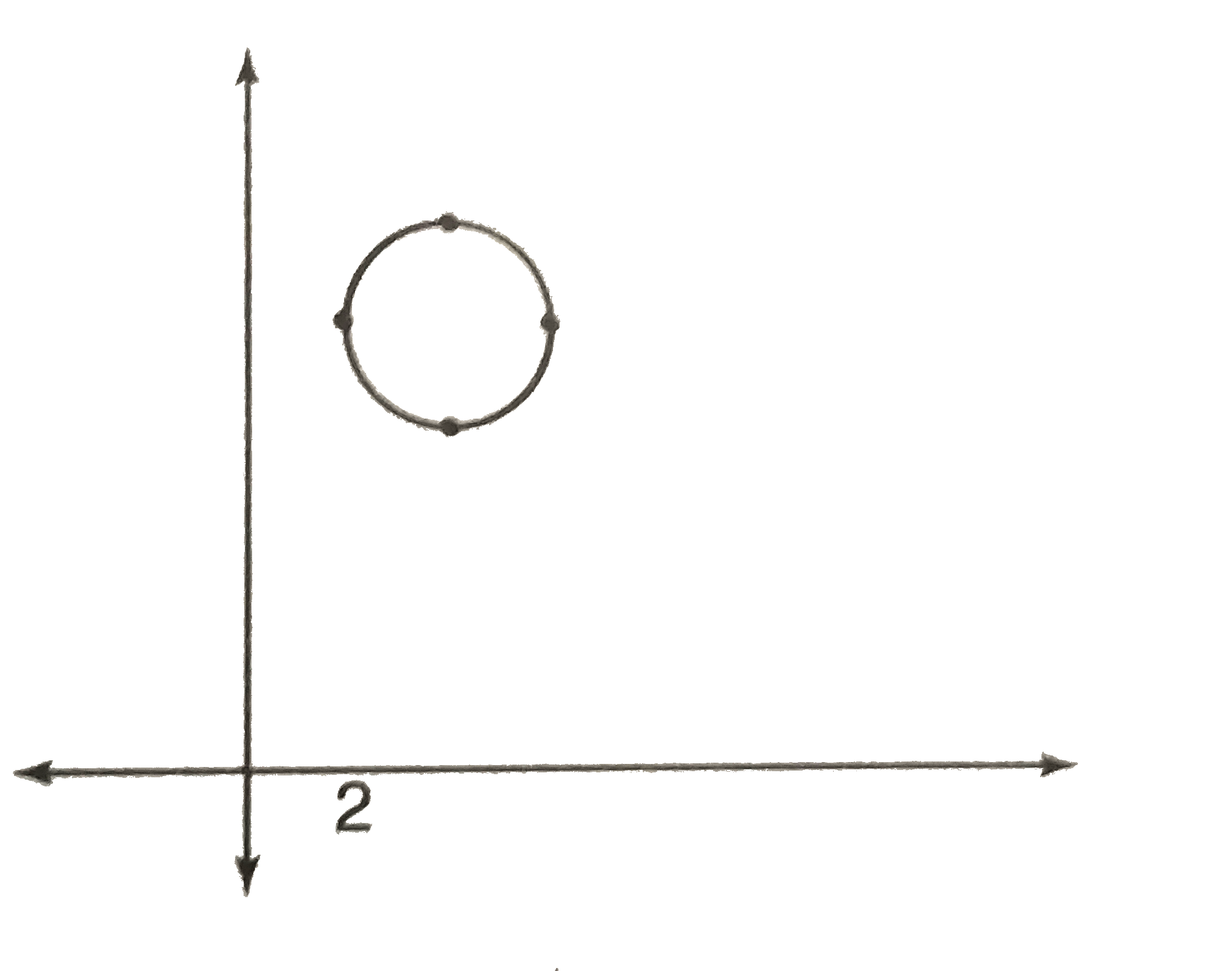
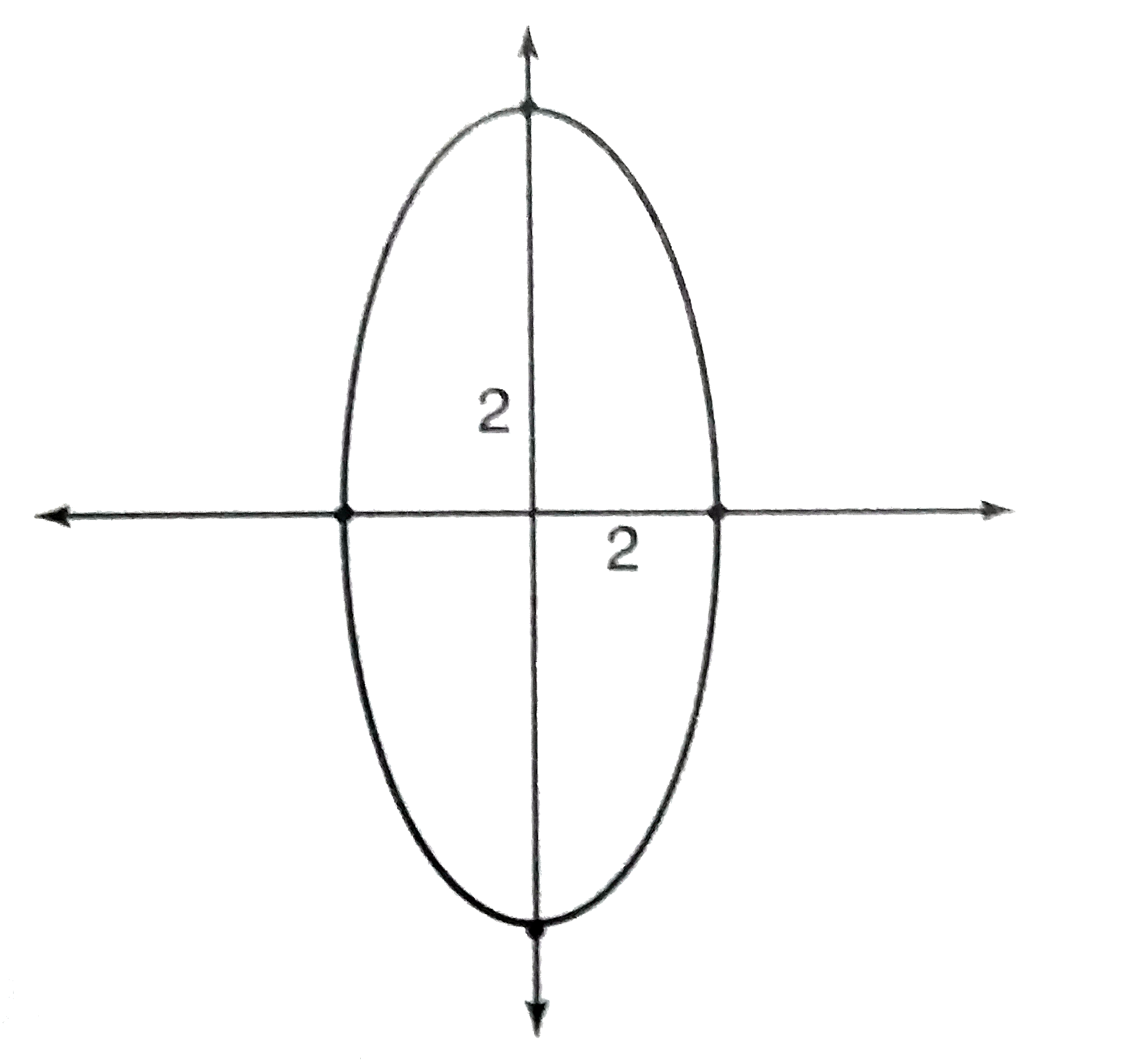
Select the graph of $\frac{{(x-4)}^2}{25}+\frac{{(y-9)}^2}{4}=1$

Options

A.
B.
C.
D.

Answer

B

Explanation

To solve the given problem, we will analyze the equation of the ellipse step by step and identify the correct graph representation.

Step 1: Identify the standard form of the ellipse
The given equation is:
\( \frac{(x-4)^2}{25} + \frac{(y-9)^2}{4} = 1 \)
This is in the standard form of an ellipse:
\( \frac{(x-h)^2}{a^2} + \frac{(y-k)^2}{b^2} = 1 \)
where \((h, k)\) is the center of the ellipse, \(a\) is the semi-major axis, and \(b\) is the semi-minor axis.

Step 2: Identify the center of the ellipse
From the equation, we can see that:
- \(h = 4\)
- \(k = 9\)

Thus, the center of the ellipse is at the point \((4, 9)\).

Step 3: Identify the lengths of the axes
Next, we determine the lengths of the semi-major and semi-minor axes:
- \(a^2 = 25 \Rightarrow a = \sqrt{25} = 5\)
- \(b^2 = 4 \Rightarrow b = \sqrt{4} = 2\)

Here, \(a = 5\) is the semi-major axis and \(b = 2\) is the semi-minor axis.

Step 4: Determine the orientation of the ellipse
Since \(a > b\), the major axis is horizontal. The lengths of the axes indicate that the ellipse stretches 5 units horizontally from the center and 2 units vertically.

Step 5: Plot the ellipse
The center of the ellipse is at \((4, 9)\), and it extends:
- Horizontally: from \(4 - 5 = -1\) to \(4 + 5 = 9\)
- Vertically: from \(9 - 2 = 7\) to \(9 + 2 = 11\)

Step 6: Compare with the given options
Now, we need to compare the characteristics of the ellipse we derived with the options provided:
- The center should be at \((4, 9)\).
- The horizontal extent should be from \(-1\) to \(9\).
- The vertical extent should be from \(7\) to \(11\).

Conclusion
After analyzing the options, we find that the graph that matches these characteristics is option B.

---